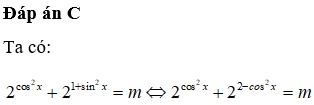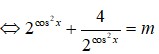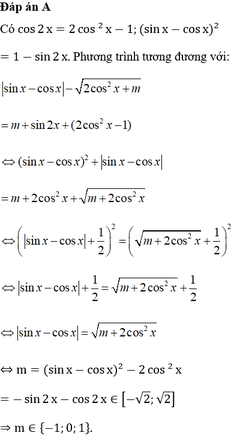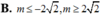2cos2x-sin2x-sin2x=m+1 tìm m để phương trình có nghiệm \(\dfrac{\pi}{2}\)+kπ
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
CN
1
HT
11 tháng 12 2020
Thấy cosx= 0 là nghiệm của phương trình => \(x=\dfrac{\pi}{2}+k\pi\)
Xét cosx khác 0, chia cả 2 vế cho cos^2 x
\(\Leftrightarrow\tan^2x-\sqrt{3}\tan x+2=1+\tan^2x\)
\(\Leftrightarrow\tan x=\dfrac{\sqrt{3}}{3}\Leftrightarrow x=\dfrac{\pi}{6}+k\pi\)
AH
Akai Haruma
Giáo viên
28 tháng 10 2021
Không có đáp án đúng. Theo đáp án thì $m=0$ thì $\sin 2x=2m$ có 2 nghiệm pb thuộc $[0;\pi]$
Tức là $\sin 2x=0$ có 2 nghiệm pb $[0;\pi]$. Mà pt này có 3 nghiệm lận:
$x=0$
$x=\frac{1}{2}\pi$
$x=\pi$
NV
Nguyễn Việt Lâm
Giáo viên
25 tháng 12 2020
\(\Leftrightarrow2\left(cos^2x-sin^2x\right)+sinx.cosx\left(sinx+cosx\right)=m\left(sinx+cosx\right)\)
\(\Leftrightarrow\left(2cosx-2sinx\right)\left(sinx+cosx\right)+sinx.cosx\left(sinx+cosx\right)=m\left(sinx+cosx\right)\)
\(\Leftrightarrow\left[{}\begin{matrix}sinx+cosx=0\left(\text{vô nghiệm trên đoạn xét}\right)\\2cosx-2sinx+sinx.cosx=m\left(1\right)\end{matrix}\right.\)
Xét (1), đặt \(t=cosx-sinx=\sqrt{2}cos\left(x+\dfrac{\pi}{4}\right)\)
\(\Rightarrow\left\{{}\begin{matrix}t\in\left[-1;1\right]\\sinx.cosx=\dfrac{1-t^2}{2}\end{matrix}\right.\)
\(\left(1\right)\Leftrightarrow2t+\dfrac{1-t^2}{2}=m\)
Xét hàm \(f\left(t\right)=-\dfrac{1}{2}t^2+2t+\dfrac{1}{2}\) trên \(\left[-1;1\right]\)
\(-\dfrac{b}{2a}=2\notin\left[-1;1\right]\) ; \(f\left(-1\right)=-2\) ; \(f\left(1\right)=2\)
\(\Rightarrow-2\le f\left(t\right)\le2\Rightarrow-2\le m\le2\)
