Giải phương trình : (x+5)4 + (x-4)4 = (2x+1)4
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
20 tháng 10 2018
Đặt \(\hept{\begin{cases}x+5=a\\x-4=b\end{cases}\Rightarrow2x+1=a+b}\)
\(\left(x+5\right)^4+\left(x-4\right)^4=\left(2x+1\right)^4\)
\(\Rightarrow a^4+b^4=\left(a+b\right)^4\)
\(\Rightarrow a^4+b^4=a^4+4a^3b+6a^2b^2+4ab^3+b^4\)
\(\Rightarrow4a^3b+6a^2b^2+4ab^3=0\)
\(\Rightarrow4ab\left[a^2+\frac{3}{2}ab+b^2\right]=0\)(1)
Mà \(a^2+\frac{3}{2}ab+b^2=\left(a+\frac{3}{4}b\right)^2+\frac{7}{16}b^2>0\)(2)
(vì nếu a và b đồng thời bằng 0 thì x + 5 và x - 4 đồng thời = 0 điều đó vô lý)
Từ (1) và (2), ta được
\(\orbr{\begin{cases}a=0\\b=0\end{cases}\Rightarrow}\orbr{\begin{cases}x+5=0\\x-4=0\end{cases}\Rightarrow\orbr{\begin{cases}x=-5\\x=4\end{cases}}}\)
Chúc bạn học tốt.
H
20 tháng 5 2023
`5-(x-6)=4(3-2x)`
`<=>5-x+6-4(3-2x)=0`
`<=> 5-x+6-12 +8x=0`
`<=> 7x -1=0`
`<=> 7x=1`
`<=>x=1/7`
Vậy pt đã cho có nghiệm `x=1/7`
__
`3-x(1-3x) =5(1-2x)`
`<=> 3-x+3x^2=5-10x`
`<=> 3-x+3x^2-5+10x=0`
`<=> 3x^2 +9x-2=0`
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{-9+\sqrt{105}}{6}\\x=\dfrac{-9-\sqrt{105}}{6}\end{matrix}\right.\)
Vậy pt đã cho có tập nghiệm \(S=\left\{\dfrac{-9+\sqrt{105}}{6};\dfrac{-9-\sqrt{106}}{5}\right\}\)
__
`(x-3)(x+4) -2(3x-2)=(x-4)^2`
`<=>x^2+4x-3x-12- 6x +4 =x^2 -8x+16`
`<=>x^2-5x-8=x^2-8x+16`
`<=> x^2 -5x-8-x^2+8x-16=0`
`<=> 3x-24=0`
`<=>3x=24`
`<=>x=8`
Vậy pt đã cho có nghiệm `x=8`
20 tháng 5 2023
a) 5-(x-6)=4(3-2x)
=> 5 – x + 6 = 12 – 8x
=> -x + 8x = 12 – 5 – 6
=> 7x = 1
=> x=1/7
Vậy phương trình có nghiệm x=1/7
b) 3 - x ( 1 - 3x)=5(1-2x)
=> 3-x+3x^2=5-10x
=> 3x^2+9x-2= 0
0=105
=> x =\(\dfrac{-9-\sqrt{105}}{6}\)
CM
25 tháng 3 2018
a) Điều kiện: x + 2 ≠ 0 và x – 2 ≠ 0 ⇔ x ≠ ± 2
(Khi đó: x2 – 4 = (x + 2)(x – 2) ≠ 0)
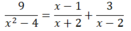
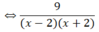
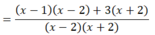
Vậy tập nghiệm của pt là: S = {-1; 1}
b) Điều kiện: 2x ≥ 0 ⇔ x ≥ 0
Khi đó: |x – 5| = 2x ⇔ x – 5 = 2x hoặc x – 5 = -2x
⇔ x = -5 hoặc x = 5/3
Vì x ≥ 0 nên ta lấy x = 5/3 . Tập nghiệm : S = {5/3}
c) x – 2)2 + 2(x – 1) ≤ x2 + 4
⇔ x2 – 4x + 4 + 2x – 2 ≤ x2 + 4
⇔ -2x ≤ 2
⇔ x ≥ -1
Tập nghiệm S = {x | x ≥ -1}
A4
1
15 tháng 5 2023
a: =>4x^2-24x+36-4x^2+4x-1<10
=>-20x<10-35=-25
=>x>=5/4
b: =>x(x^2-25)-x^3-8<=3
=>x^3-25x-x^3-8<=3
=>-25x<=11
=>x>=-11/25
XL
2
MA
25 tháng 2 2022
ĐKXĐ: ` x ne 1 ; x ne 4`
`(2x+1)/(x^2-5x+4) + 5/(x-1) = 2/(x-4)`
`<=> (2x+1)/[(x-1)(x-4)] + [5(x-4)]/[(x-1)(x-4)] = [2(x-1)]/[(x-1)(x-4)]`
`=> 2x+1 + 5x -20 = 2x-2`
`<=> 5x = 17`
`<=> x= 17/5`(thỏa mãn ĐKXĐ)
Vậy tập nghiệm của phương trình là `S={ 17/5}`
VG
1
TT
23 tháng 2 2020
1) \(\left(2x+5\right)\left(x-4\right)=\left(x-5\right)\left(4-x\right)\)
\(\Leftrightarrow\left(2x+5\right)\left(x-4\right)+\left(x-4\right)\left(x-5\right)=0\)
\(\Leftrightarrow\left(x-4\right)\cdot3x=0\)
\(\Leftrightarrow\orbr{\begin{cases}x=4\\x=0\end{cases}}\)
2) \(9x^2-1=3x+1\)
\(\Leftrightarrow\left(3x+1\right)\left(3x-1\right)-\left(3x+1\right)=0\)
\(\Leftrightarrow\left(3x+1\right)\left(3x-2\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}x=-\frac{1}{3}\\x=\frac{2}{3}\end{cases}}\)
Y
31 tháng 5 2023
\(a,4\left(x-3\right)^2-\left(2x-1\right)^2< 10\)
\(\Leftrightarrow4\left(x^2-6x+9\right)-\left(4x^2-4x+1\right)-10< 0\)
\(\Leftrightarrow4x^2-24x+36-4x^2+4x-1-10< 0\)
\(\Leftrightarrow-20x< -25\)
\(\Leftrightarrow x>\dfrac{5}{4}\)
\(b,x\left(x-5\right)\left(x+5\right)-\left(x+2\right)\left(x^2-2x+4\right)\le3\)
\(\Leftrightarrow x\left(x^2-25\right)-\left(x^3-2x^2+4x+2x^2-4x+8\right)\le3\)
\(\Leftrightarrow x^3-25x-\left(x^3+8\right)\le3\)
\(\Leftrightarrow x^3-25x-x^3-8-3\le0\)
\(\Leftrightarrow-25x\le11\)
\(\Leftrightarrow x\ge-\dfrac{11}{25}\)
NT
1
24 tháng 6 2018
| x | \(\frac{5}{2}\) | 4 | |||
| 2x-5 | - | 0 | + | | | + |
| x-4 | - | | | - | 0 | + |
+) Nếu \(x\le\frac{5}{2}\Leftrightarrow\left|2x-5\right|=5-2x\)
\(\left|x-4\right|=4-x\)
\(pt\Leftrightarrow5-2x-4+x=4x\)
\(\Leftrightarrow-5x=-1\)
\(\Leftrightarrow x=\frac{1}{5}\left(tm\right)\)
+) Nếu \(\frac{5}{2}< x\le4\Leftrightarrow\left|2x-5\right|=2x-5\)
\(\left|x-4\right|=4-x\)
\(pt\Leftrightarrow2x-5-4+x=4x\)
\(\Leftrightarrow-x=9\)
\(\Leftrightarrow x=-9\) (loại)
+) Nếu \(x>4\Leftrightarrow\left|2x-5\right|=2x-5\)
\(\left|x-4\right|=x-4\)
\(pt\Leftrightarrow2x-5-x+4=4x\)
\(\Leftrightarrow-3x=1\)
\(\Leftrightarrow x=-\frac{1}{3}\)( loại )
Vậy ...
( p/s : câu b tương tự )
AH
Akai Haruma
Giáo viên
15 tháng 4 2023
Lời giải:
ĐKXĐ: $x\neq 0; \frac{-3}{2}; \frac{-1}{2}; -3$
PT $\Leftrightarrow (\frac{1}{x}-\frac{3}{2x+1})+(\frac{5}{2x+3}-\frac{4}{x+3})=0$
$\Leftrightarrow \frac{1-x}{x(2x+1)}+\frac{3-3x}{(2x+3)(x+3)}=0$
$\Leftrightarrow \frac{1-x}{x(2x+1)}+\frac{3(1-x)}{(2x+3)(x+3)}=0$
$\Leftrightarrow (1-x)\left[\frac{1}{x(2x+1)}+\frac{3}{(2x+3)(x+3)}\right]=0$
TH1: $1-x=0\Leftrightarrow x=1$ (tm)
TH2: $\frac{1}{x(2x+1)}+\frac{3}{(2x+3)(x+3)}=0$
$\Rightarrow (2x+3)(x+3)+3x(2x+1)=0$
$\Leftrightarrow 8x^2+12x+9=0$
$\Leftrightarrow (2x+3)^2+4x^2=0$
$\Rightarrow (2x+3)^2=x^2=0$ (vô lý)
Do đó $x=1$ là nghiệm duy nhất.
giúp mình với
Theo bài ra , ta có :
\(\left(x+5\right)^4+\left(x-4\right)^4=\left(2x+1\right)^4\)
\(\Leftrightarrow\left(x+5\right)^2+\left(x-4\right)^2=\left(2x+1\right)^2\)
\(\Leftrightarrow x^2+10x+25+x^2-8x+16=4x^2+4x+1\)
\(\Leftrightarrow2x^2+2x+41=4x^2+4x+1\)
\(\Leftrightarrow2x^2-4x^2+2x-4x=1-41\)
\(\Leftrightarrow-2x^2-2x=-40\)
\(\Leftrightarrow-2\left(x^2+x\right)=-40\)
\(\Leftrightarrow x^2+x=20\)
\(\Leftrightarrow x\left(x+1\right)=20\)
Lập bảng số từ 1 ---) 4 ( Vì nếu x là 0 ptvn )
Nhìn vào bảng ta thấy tại giá trị x = 4 ta đk pt có giá trị bằng 20
Tập nghiệm của phương trình là \(S=\left\{4\right\}\)
Chúc bạn học tốt =))