Cho a + b = \(\dfrac{\Pi}{4}\). Tính: \(M=\left(1+tana\right)\left(1+tanb\right)\).
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
AH
Akai Haruma
Giáo viên
11 tháng 4 2018
Câu a)
Ta sử dụng 2 công thức:
\(\bullet \tan (180-\alpha)=-\tan \alpha\)
\(\bullet \tan (\alpha+\beta)=\frac{\tan \alpha+\tan \beta}{1-\tan \alpha.\tan \beta}\)
Áp dụng vào bài toán:
\(\text{VT}=\tan A+\tan B+\tan C=\tan A+\tan B+\tan (180-A-B)\)
\(=\tan A+\tan B-\tan (A+B)=\tan A+\tan B-\frac{\tan A+\tan B}{1-\tan A.\tan B}\)
\(=(\tan A+\tan B)\left(1+\frac{1}{1-\tan A.\tan B}\right)=(\tan A+\tan B).\frac{-\tan A.\tan B}{1-\tan A.\tan B}\)
\(=-\tan A.\tan B.\frac{\tan A+\tan B}{1-\tan A.\tan B}=-\tan A.\tan B.\tan (A+B)\)
\(=\tan A.\tan B.\tan (180-A-B)\)
\(=\tan A.\tan B.\tan C=\text{VP}\)
Do đó ta có đpcm
Tam giác $ABC$ có ba góc nhọn nên \(\tan A, \tan B, \tan C>0\)
Áp dụng BĐT Cauchy ta có:
\(P=\tan A+\tan B+\tan C\geq 3\sqrt[3]{\tan A.\tan B.\tan C}\)
\(\Leftrightarrow P=\tan A+\tan B+\tan C\geq 3\sqrt[3]{\tan A+\tan B+\tan C}\)
\(\Rightarrow P\geq 3\sqrt[3]{P}\)
\(\Rightarrow P^3\geq 27P\Leftrightarrow P(P^2-27)\geq 0\)
\(\Rightarrow P^2-27\geq 0\Rightarrow P\geq 3\sqrt{3}\)
Vậy \(P_{\min}=3\sqrt{3}\). Dấu bằng xảy ra khi \(\angle A=\angle B=\angle C=60^0\)
AH
Akai Haruma
Giáo viên
11 tháng 4 2018
Câu b)
Ta sử dụng 2 công thức chính:
\(\bullet \tan (\alpha+\beta)=\frac{\tan \alpha+\tan \beta}{1-\tan \alpha.\tan \beta}\)
\(\bullet \tan (90-\alpha)=\frac{1}{\tan \alpha}\)
Áp dụng vào bài toán:
\(\text{VT}=\tan \frac{A}{2}.\tan \frac{B}{2}+\tan \frac{B}{2}.\tan \frac{C}{2}+\tan \frac{C}{2}.\tan \frac{A}{2}\)
\(=\tan \frac{A}{2}.\tan \frac{B}{2}+\tan \frac{C}{2}(\tan \frac{A}{2}+\tan \frac{B}{2})\)
\(=\tan \frac{A}{2}.\tan \frac{B}{2}+\tan (90-\frac{A+B}{2})(\tan \frac{A}{2}+\tan \frac{B}{2})\)
\(=\tan \frac{A}{2}.\tan \frac{B}{2}+\frac{\tan \frac{A}{2}+\tan \frac{B}{2}}{\tan (\frac{A+B}{2})}\)
\(=\tan \frac{A}{2}.\tan \frac{B}{2}+\frac{\tan \frac{A}{2}+\tan \frac{B}{2}}{\frac{\tan \frac{A}{2}+\tan \frac{B}{2}}{1-\tan \frac{A}{2}.\tan \frac{B}{2}}}\)
\(=\tan \frac{A}{2}.\tan \frac{B}{2}+1-\tan \frac{A}{2}.\tan \frac{B}{2}=1=\text{VP}\)
Ta có đpcm.
Cũng giống phần a, ta biết do ABC là tam giác nhọn nên
\(\tan A, \tan B, \tan C>0\)
Đặt \(\tan A=x, \tan B=y, \tan C=z\). Ta có: \(xy+yz+xz=1\)
Và \(T=x+y+z\)
\(\Rightarrow T^2=x^2+y^2+z^2+2(xy+yz+xz)\)
Theo hệ quả quen thuộc của BĐT Cauchy:
\(x^2+y^2+z^2\geq xy+yz+xz\)
\(\Rightarrow T^2\geq 3(xy+yz+xz)=3\)
\(\Rightarrow T\geq \sqrt{3}\Leftrightarrow T_{\min}=\sqrt{3}\)
Dấu bằng xảy ra khi \(x=y=z=\frac{1}{\sqrt{3}}\Leftrightarrow \angle A=\angle B=\angle C=60^0\)
15 tháng 10 2017
mình làm cách này là cách khj nào mà ko cách nào khác ms làm vậy thôi, áp dụng định lí sin và cosin trong tam giác
23 tháng 3 2018
rút gọn biểu thức:
E=cos(\(\dfrac{3\pi}{3}-\alpha\))-sin(\(\dfrac{3\pi}{2}-\alpha\))+sin(\(\alpha+4\pi\))
AH
Akai Haruma
Giáo viên
27 tháng 2 2019
Bài 1:
a)
\(\sin ^2x+\sin ^2x\cot^2x=\sin ^2x(1+\cot^2x)=\sin ^2x(1+\frac{\cos ^2x}{\sin ^2x})\)
\(=\sin ^2x.\frac{\sin ^2x+\cos^2x}{\sin ^2x}=\sin ^2x+\cos^2x=1\)
b)
\((1-\tan ^2x)\cot^2x+1-\cot^2x\)
\(=\cot^2x(1-\tan^2x-1)+1=\cot^2x(-\tan ^2x)+1=-(\tan x\cot x)^2+1\)
\(=-1^2+1=0\)
c)
\(\sin ^2x\tan x+\cos^2x\cot x+2\sin x\cos x=\sin ^2x.\frac{\sin x}{\cos x}+\cos ^2x.\frac{\cos x}{\sin x}+2\sin x\cos x\)
\(=\frac{\sin ^3x}{\cos x}+\frac{\cos ^3x}{\sin x}+2\sin x\cos x=\frac{\sin ^4x+\cos ^4x+2\sin ^2x\cos ^2x}{\sin x\cos x}=\frac{(\sin ^2x+\cos ^2x)^2}{\sin x\cos x}=\frac{1}{\sin x\cos x}\)
\(=\frac{1}{\frac{\sin 2x}{2}}=\frac{2}{\sin 2x}\)
AH
Akai Haruma
Giáo viên
27 tháng 2 2019
Bài 2:
Áp dụng BĐT Cauchy Schwarz ta có:
\(P=\frac{a^2}{\sqrt{a(2c+a+b)}}+\frac{b^2}{\sqrt{b(2a+b+c)}}+\frac{c^2}{\sqrt{c(2b+c+a)}}\)
\(\geq \frac{(a+b+c)^2}{\sqrt{a(2c+a+b)}+\sqrt{b(2a+b+c)}+\sqrt{c(2b+c+a)}}(*)\)
Tiếp tục áp dụng BĐT Cauchy-Schwarz:
\((\sqrt{a(2c+a+b)}+\sqrt{b(2a+b+c)}+\sqrt{c(2b+c+a)})^2\leq (a+b+c)(2c+a+b+2a+b+c+2b+c+a)\)
\(\Leftrightarrow (\sqrt{a(2c+a+b)}+\sqrt{b(2a+b+c)}+\sqrt{c(2b+c+a)})^2\leq 4(a+b+c)^2\)
\(\Rightarrow \sqrt{a(2c+a+b)}+\sqrt{b(2a+b+c)}+\sqrt{c(2b+c+a)}\leq 2(a+b+c)(**)\)
Từ \((*); (**)\Rightarrow P\geq \frac{(a+b+c)^2}{2(a+b+c)}=\frac{a+b+c}{2}=\frac{3}{2}\)
Vậy \(P_{\min}=\frac{3}{2}\)
Dấu "=" xảy ra khi $a=b=c=1$
HP
8 tháng 2 2022
a, ĐK: \(x\ne\dfrac{5\pi}{6}+k2\pi;x\ne\dfrac{\pi}{6}+k2\pi\)
\(\dfrac{2sin^2\left(\dfrac{3x}{2}-\dfrac{\pi}{4}\right)+\sqrt{3}cos^3x\left(1-3tan^2x\right)}{2sinx-1}=-1\)
\(\Leftrightarrow2sin^2\left(\dfrac{3x}{2}-\dfrac{\pi}{4}\right)+\sqrt{3}cos^3x\left(1-3tan^2x\right)=1-2sinx\)
\(\Leftrightarrow-cos\left(3x-\dfrac{\pi}{2}\right)+\sqrt{3}cos^3x.\dfrac{cos^2x-3sin^2x}{cos^2x}=-2sinx\)
\(\Leftrightarrow-sin3x+\sqrt{3}cosx.\left(cos^2x-3sin^2x\right)=-2sinx\)
\(\Leftrightarrow-sin3x+\sqrt{3}cosx.\left(4cos^2x-3\right)=-2sinx\)
\(\Leftrightarrow-sin3x+\sqrt{3}cos3x=-2sinx\)
\(\Leftrightarrow\dfrac{1}{2}sin3x-\dfrac{\sqrt{3}}{2}cos3x-sinx=0\)
\(\Leftrightarrow sin\left(3x-\dfrac{\pi}{3}\right)-sinx=0\)
\(\Leftrightarrow2cos\left(2x-\dfrac{\pi}{6}\right)sin\left(x-\dfrac{\pi}{6}\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cos\left(2x-\dfrac{\pi}{6}\right)=0\\sin\left(x-\dfrac{\pi}{6}\right)=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}2x-\dfrac{\pi}{6}=\dfrac{\pi}{2}+k\pi\\x-\dfrac{\pi}{6}=k\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\pi}{3}+\dfrac{k\pi}{2}\\x=\dfrac{\pi}{6}+k\pi\end{matrix}\right.\)
Đối chiếu điều kiện ta được:
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\pi}{3}+k\pi\\x=\dfrac{7\pi}{6}+k2\pi\\x=-\dfrac{\pi}{6}+k2\pi\end{matrix}\right.\)
NV
Nguyễn Việt Lâm
Giáo viên
15 tháng 2 2022
(Giả sử chọn k=-1)
Đặt \(u_n=v_n-1\Rightarrow v_{n+1}-1=\dfrac{5\left(v_n-1\right)+4}{v_n-1+2}=\dfrac{5v_n-1}{v_n+1}\)
\(\Rightarrow v_{n+1}=1+\dfrac{5v_n-1}{v_n+1}=\dfrac{6v_n}{v_n+1}\)
Mục đích chỉ cần biến đổi tới đây, sau đó nghịch đảo 2 vế:
\(\Rightarrow\dfrac{1}{v_{n+1}}=\dfrac{v_n+1}{6v_n}=\dfrac{1}{6v_n}+\dfrac{1}{6}\)
Đặt \(\dfrac{1}{v_n}=x_n\Rightarrow\left\{{}\begin{matrix}x_1=\dfrac{1}{v_1}=\dfrac{1}{u_1+1}=\dfrac{1}{6}\\x_{n+1}=\dfrac{1}{6}x_n+\dfrac{1}{6}\end{matrix}\right.\)
Rồi đó, đưa về dãy cơ bản \(\Rightarrow x_{n+1}-\dfrac{1}{5}=\dfrac{1}{6}\left(x_n-\dfrac{1}{5}\right)\)
Đặt \(x_n-\dfrac{1}{5}=y_n\Rightarrow\left\{{}\begin{matrix}y_1=x_1-\dfrac{1}{5}=-\dfrac{1}{30}\\y_{n+1}=\dfrac{1}{6}y_n\end{matrix}\right.\)
\(\Rightarrow y_n=-\dfrac{1}{30}\left(\dfrac{1}{6}\right)^{n-1}\Rightarrow x_n=y_n+\dfrac{1}{5}=-\dfrac{1}{30}.\left(\dfrac{1}{6}\right)^{n-1}+\dfrac{1}{5}\)
\(\Rightarrow v_n=\dfrac{1}{x_n}=...\Rightarrow u_n=v_n-1=\dfrac{1}{x_n}-1=...\)
Cách này là cách cơ bản, có hướng làm cố định để đưa về các dãy quen thuộc
NV
Nguyễn Việt Lâm
Giáo viên
26 tháng 2 2023
a.
\(\sqrt{2}sin\left(2x+\dfrac{\pi}{4}\right)=3sinx+cosx+2\)
\(\Leftrightarrow sin2x+cos2x=3sinx+cosx+2\)
\(\Leftrightarrow2sinx.cosx-3sinx+2cos^2x-cosx-3=0\)
\(\Leftrightarrow sinx\left(2cosx-3\right)+\left(cosx+1\right)\left(2cosx-3\right)=0\)
\(\Leftrightarrow\left(2cosx-3\right)\left(sinx+cosx+1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cosx=\dfrac{3}{2}\left(vn\right)\\sinx+cosx+1=0\end{matrix}\right.\)
\(\Rightarrow\sqrt{2}sin\left(x+\dfrac{\pi}{4}\right)=-1\)
\(\Leftrightarrow sin\left(x+\dfrac{\pi}{4}\right)=-\dfrac{\sqrt{2}}{2}\)
\(\Leftrightarrow...\)
NV
Nguyễn Việt Lâm
Giáo viên
26 tháng 2 2023
b.
ĐKXĐ: \(cosx\ne\dfrac{1}{2}\Rightarrow\left[{}\begin{matrix}x\ne\dfrac{\pi}{3}+k2\pi\\x\ne-\dfrac{\pi}{3}+k2\pi\end{matrix}\right.\)
\(\dfrac{\left(2-\sqrt{3}\right)cosx-2sin^2\left(\dfrac{x}{2}-\dfrac{\pi}{4}\right)}{2cosx-1}=1\)
\(\Rightarrow\left(2-\sqrt{3}\right)cosx+cos\left(x-\dfrac{\pi}{2}\right)=2cosx\)
\(\Leftrightarrow-\sqrt{3}cosx+sinx=0\)
\(\Leftrightarrow sin\left(x-\dfrac{\pi}{3}\right)=0\)
\(\Rightarrow x-\dfrac{\pi}{3}=k\pi\)
\(\Rightarrow x=\dfrac{\pi}{3}+k\pi\)
Kết hợp ĐKXĐ \(\Rightarrow x=\dfrac{4\pi}{3}+k2\pi\)
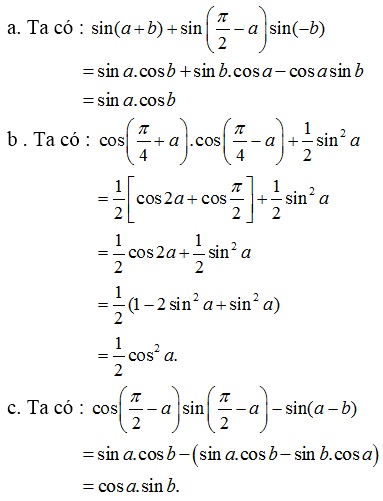
\(1=tan\left(\dfrac{\pi}{4}\right)=tan\left(a+b\right)=\dfrac{tana+tanb}{1-tana.tanb}\)
\(\Rightarrow tana+tanb=1-tana.tanb\)
\(\Rightarrow tana+tanb+tana.tanb=1\)
Do đó:
\(M=1+tana+tanb+tana.tanb=1+1=2\)