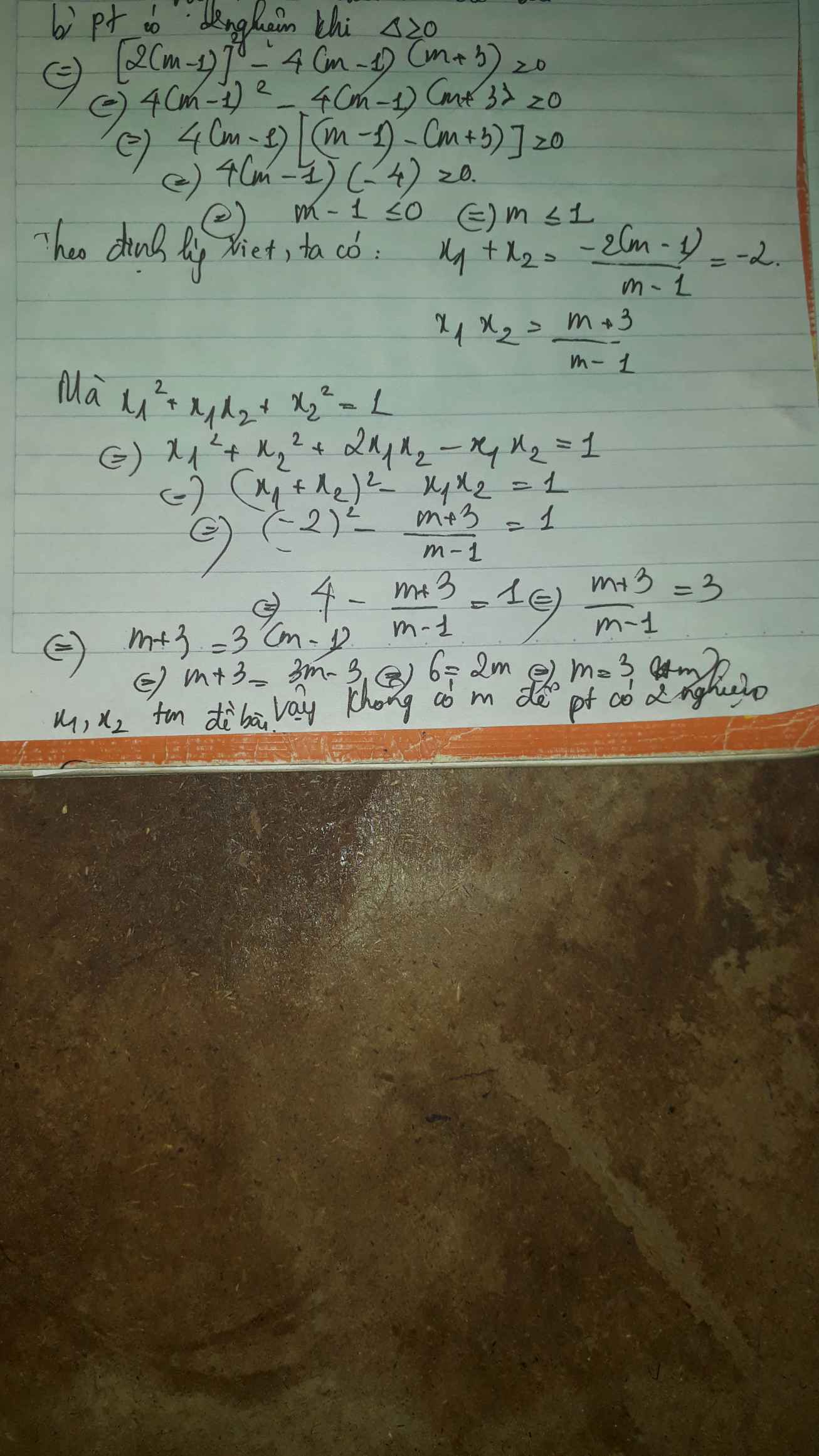tìm m để pt x\(^2-2mx+m^2-4=0\) có 2 nghiệm phân biệt thỏa mãn \(\dfrac{3x_1+x_2}{x_1x_2}\)
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
15 tháng 5 2022
\(\Delta=\left[-2\left(m+1\right)\right]^2-4\left(m^2+2\right)\)
\(=4m^2+8m+4-4m^2-8\)
\(=8m-4\)
Để pt có 2 nghiệm thì \(\Delta>0\)
\(\Leftrightarrow8m-4>0\)
\(\Leftrightarrow m>\dfrac{1}{2}\)
Theo hệ thức Vi-ét, ta có:\(\left\{{}\begin{matrix}x_1+x_2=2\left(m+1\right)\\x_1x_2=m^2+2\end{matrix}\right.\)
\(x_1^2+x_1x_2+2=3x_1+x_2\)
\(\Leftrightarrow x_1^2+m^2+2+2=2x_1+2\left(m+1\right)\)
\(\Leftrightarrow x_1^2-2x_1+4+m^2-2m-2=0\)
\(\Leftrightarrow x_1^2-2x_1+2+m^2-2m=0\)
\(\Leftrightarrow x_1^2-2x_1+1+m^2-2m+1=0\)
\(\Leftrightarrow\left(x_1-1\right)^2+\left(m-1\right)^2=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x_1=1\\m=1\end{matrix}\right.\)(tm)
Vậy \(m=1\)
25 tháng 6 2021
ĐK:`x_1,x_2 ne 0=>x_1.x_2 ne 0`
`=>-2m-1 ne 0=>m ne -1/2`
Ta có:`a=1,b=2m,c=-2m-1`
`=>a+b+c=1+2m-2m-1=0`
`<=>` \(\left[ \begin{array}{l}x=1\\x=-2m-1\end{array} \right.\)
PT có 2 nghiệm pn
`=>-2m-1 ne 1`
`=>-2m ne 2`
`=>m ne -1`
Nếu `x_1=1,x_2=-2m-1`
`pt<=>6=1+1/(-2m-1)`
`<=>5=1/(-2m-1)`
`<=>2m+1=-1/5`
`<=>2m=-6/5`
`<=>m=-3/5(tm)`
Nếu `x_2=1,x_1=-2m-1`
`pt<=>6/(-2m-1)=-2m-1+1=-2m`
`<=>6/(2m+1)=2m`
`<=>3/(2m+1)=m`
`<=>2m^2+m-3=0`
`a+b+c=0`
`=>m_1=1(tm),m_2=-c/a=-3/2(tm)`
Vậy `m in {-3/5,1,-3/2}` thì ....
7 tháng 2 2022
Theo Vi et \(\left\{{}\begin{matrix}x_1+x_2=2\left(m-4\right)\\x_1x_2=-m^2+4\end{matrix}\right.\)
\(\dfrac{x_1+x_2}{x_1x_2}+\dfrac{4}{x_1x_2}=1\)
Thay vào ta được : \(\dfrac{2\left(m-4\right)+4}{-m^2+4}=1\Leftrightarrow\dfrac{2m-4}{\left(2-m\right)\left(m+2\right)}=1\Leftrightarrow\dfrac{-2}{m+2}=1\Rightarrow-2=m+2\Leftrightarrow m=-4\)
HP
12 tháng 8 2021
b) phương trình có 2 nghiệm \(\Leftrightarrow\Delta'\ge0\)
\(\Leftrightarrow\left(m-1\right)^2-\left(m-1\right)\left(m+3\right)\ge0\)
\(\Leftrightarrow m^2-2m+1-m^2-3m+m+3\ge0\)
\(\Leftrightarrow-4m+4\ge0\)
\(\Leftrightarrow m\le1\)
Ta có: \(x_1^2+x_1x_2+x_2^2=1\)
\(\Leftrightarrow\left(x_1+x_2\right)^2-2x_1x_2=1\)
Theo viet: \(\left\{{}\begin{matrix}x_1+x_2=-\dfrac{b}{a}=2\left(m-1\right)\\x_1x_2=\dfrac{c}{a}=m+3\end{matrix}\right.\)
\(\Leftrightarrow\left[-2\left(m-1\right)^2\right]-2\left(m+3\right)=1\)
\(\Leftrightarrow4m^2-8m+4-2m-6-1=0\)
\(\Leftrightarrow4m^2-10m-3=0\)
\(\Leftrightarrow\left[{}\begin{matrix}m_1=\dfrac{5+\sqrt{37}}{4}\left(ktm\right)\\m_2=\dfrac{5-\sqrt{37}}{4}\left(tm\right)\end{matrix}\right.\Rightarrow m=\dfrac{5-\sqrt{37}}{4}\)
NV
Nguyễn Việt Lâm
Giáo viên
14 tháng 4 2022
1.
\(a+b+c=0\) nên pt luôn có 2 nghiệm
\(\left\{{}\begin{matrix}x_1+x_2=m\\x_1x_2=m-1\end{matrix}\right.\)
\(A=\dfrac{2x_1x_2+3}{x_1^2+x_2^2+2x_1x_2+2}=\dfrac{2x_1x_2+3}{\left(x_1+x_2\right)^2+2}=\dfrac{2\left(m-1\right)+3}{m^2+2}=\dfrac{2m+1}{m^2+2}\)
\(A=\dfrac{m^2+2-\left(m^2-2m+1\right)}{m^2+2}=1-\dfrac{\left(m-1\right)^2}{m^2+2}\le1\)
Dấu "=" xảy ra khi \(m=1\)
2.
\(\Delta=m^2-4\left(m-2\right)=\left(m-2\right)^2+4>0;\forall m\) nên pt luôn có 2 nghiệm pb
Theo Viet: \(\left\{{}\begin{matrix}x_1+x_2=m\\x_1x_2=m-2\end{matrix}\right.\)
\(\dfrac{\left(x_1^2-2\right)\left(x_2^2-2\right)}{\left(x_1-1\right)\left(x_2-1\right)}=4\Rightarrow\dfrac{\left(x_1x_2\right)^2-2\left(x_1^2+x_2^2\right)+4}{x_1x_2-\left(x_1+x_2\right)+1}=4\)
\(\Rightarrow\dfrac{\left(x_1x_2\right)^2-2\left(x_1+x_2\right)^2+4x_1x_2+4}{x_1x_2-\left(x_1+x_2\right)+1}=4\)
\(\Rightarrow\dfrac{\left(m-2\right)^2-2m^2+4\left(m-2\right)+4}{m-2-m+1}=4\)
\(\Rightarrow-m^2=-4\Rightarrow m=\pm2\)
