-x2 + 2xy - y2 / x+ y = ? / y2 - x2
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
MS
1
25 tháng 10 2021
a: \(\dfrac{x^2+2xy+y^2}{x+y}=x+y\)
b: \(\dfrac{64x^3+1}{4x+1}=16x^2-4x+1\)
MD
2
28 tháng 9 2021
a) \(\left(x^2+2xy+y^2\right):\left(x+y\right)=\left(x+y\right)^2:\left(x+y\right)=x+y\)
b) \(=\left[\left(5x+1\right)\left(25x^2-5x+1\right)\right]:\left(5x+1\right)=25x^2-5x+1\)
c) \(=\left(y-x\right)^2:\left(y-x\right)=y-x\)
28 tháng 9 2021
\(a,=\left(x+y\right)^2:\left(x+y\right)=x+y\\ b,=\left(5x+1\right)\left(25x^2-5x+1\right):\left(5x+1\right)=25x^2-5x+1\\ c,=\left(y-x\right)^2:\left(y-x\right)=y-x\)
NH
2
11 tháng 8 2021
a) Ta có: \(M=x^2-2xy+y^2-10x+10y\)
\(=\left(x-y\right)^2-10\left(x-y\right)\)
\(=9^2-10\cdot9=-9\)
S
6 tháng 12 2021
Lời giải:
a) (x2 + 2xy + y2) : (x + y)
= (x + y)2 : (x + y)
= x + y
b) (125x3 + 1) : (5x + 1)
= [(5x)3 + 1] : (5x + 1)
= (5x + 1)[(5x)2 – 5x + 1]] : (5x + 1)
= (5x)2 – 5x + 1
= 25x2 – 5x + 1
c) (x2 – 2xy + y2) : (y – x)
= (x – y)2 : [-(x – y)]
= -(x – y)
= y – x
Hoặc (x2 – 2xy + y2) : (y – x)
= (y2 – 2yx + x2) : (y – x)
= (y – x)2 : (y – x)
= y – x
PN
1
7 tháng 3 2021
Theo bài ra, ta có: \(x^2-y=y^2-x\Leftrightarrow x^2-y^2=-x+y\)
\(\Leftrightarrow\left(x-y\right)\left(x+y\right)=-\left(x-y\right)\)
\(\Leftrightarrow\left(x+y\right)=-1\)
Ta lại có: \(A=x^2+2xy+y^2-3x-3y=\left(x+y\right)^2-3\left(x+y\right)\)
Thay x+y=-1 vào biểu thức A, ta được: \(A=\left(-1\right)^2-3.\left(-1\right)=1+3=4\)
Vậy A=4
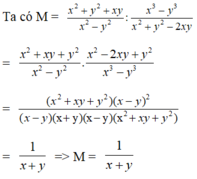
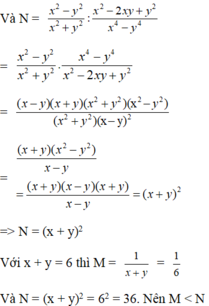
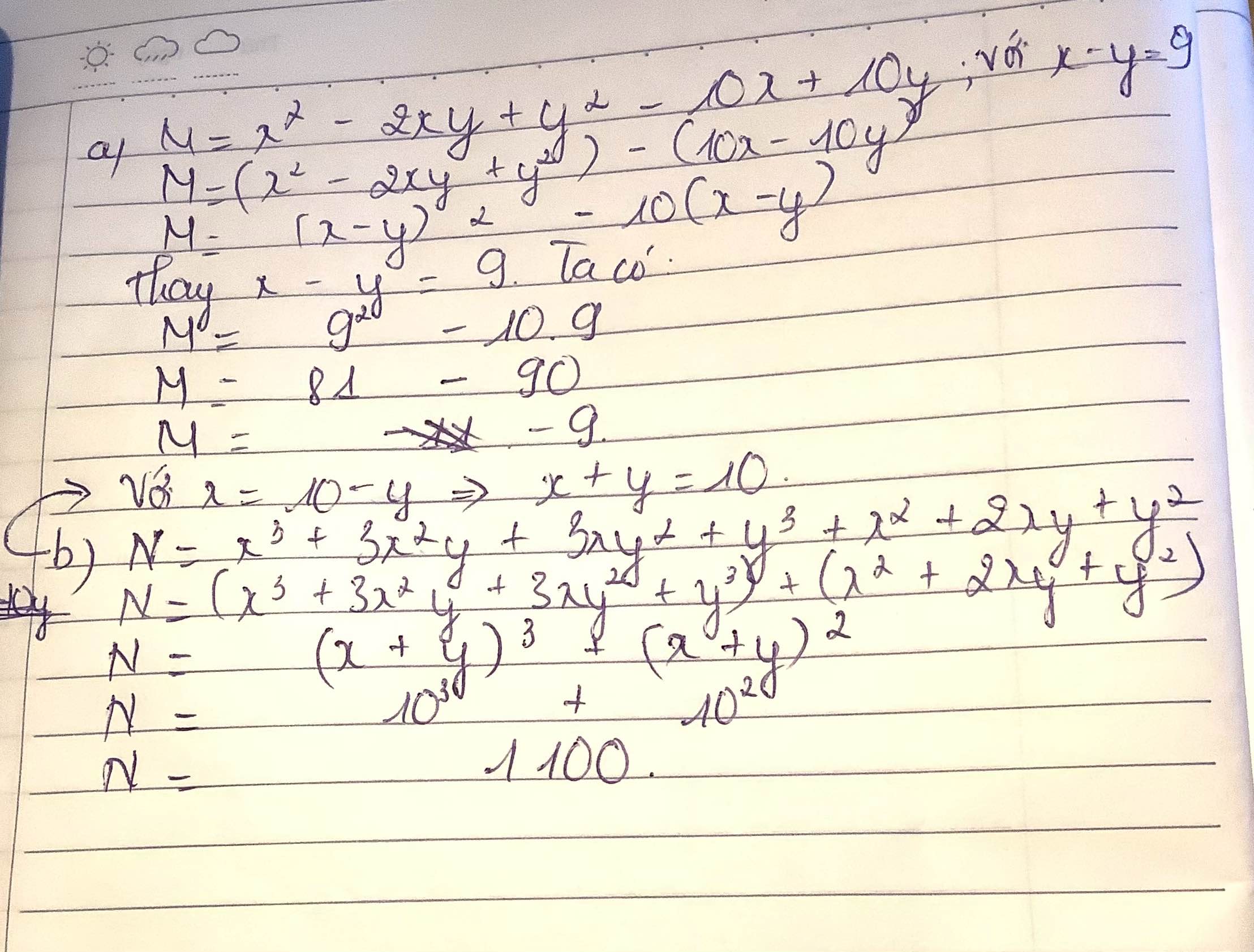
\(\dfrac{-x^2+2xy-y^2}{x+y}=\dfrac{-\left(x^2-2xy+y^2\right)}{x+y}=\dfrac{-\left(x-y\right)^2}{\left(x+y\right)}=\dfrac{-\left(x-y\right)^3}{\left(x+y\right)\left(x-y\right)}=\dfrac{-\left(x-y\right)^3}{x^2-y^2}=\dfrac{\left(x-y\right)^3}{y^2-x^2}\Rightarrow?=\left(x-y\right)^3\)
\(\dfrac{-x^2+2xy-y^2}{x+y}=\dfrac{?}{y^2-x^2}\)
\(\dfrac{-\left(x-y\right)^2}{x+y}=\dfrac{-?}{x^2-y^2}\)
\(\dfrac{-\left(x-y\right)^2}{x+y}=\dfrac{-?}{\left(x-y\right)\left(x+y\right)}\)
\(-?\left(x+y\right)=-\left(x-y\right)^3\left(x+y\right)\)
\(?=\left(x-y\right)^3\)