gaasppppppppppppppppppp lắm ạ giúp em với
BÀI 1:Quy đồng mẫu thức các phân thức sau:
a)\(\dfrac{y-1}{y+1};\dfrac{y+1}{y-1};\dfrac{1}{y^2-1}\)
b) \(\dfrac{2}{y^2-4y};\dfrac{y}{y^2-16}\)
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

1.
\(\dfrac{3x-2}{3}-2=\dfrac{4x+1}{4}\\ \Leftrightarrow\dfrac{4.\left(3x-2\right)}{12}-\dfrac{24}{12}=\dfrac{3.\left(4x+1\right)}{12}\\ \Leftrightarrow12x-8-24=12x+3\\ \Leftrightarrow12x-8-24-12x-3=0\\ \Leftrightarrow-35=0\)
Vậy PT vô nghiệm
2.
\(\dfrac{x-3}{4}+\dfrac{2x-1}{3}=\dfrac{2-x}{6}\\ \Leftrightarrow\dfrac{3\left(x-3\right)}{12}+\dfrac{4\left(2x-1\right)}{12}=\dfrac{2\left(2-x\right)}{12}\\ \Leftrightarrow3x-9+8x-4=4-2x=0\\ \Leftrightarrow13x-17=0\\ \Leftrightarrow13x=17\\ \Leftrightarrow x=\dfrac{17}{13}\)
Vậy PT có tập nghiệm là S = { \(\dfrac{17}{13}\) }
3.
\(\dfrac{-\left(x-3\right)}{2}-2=\dfrac{5\left(x+2\right)}{4}\\ \Leftrightarrow\dfrac{-2.\left(x-3\right)}{4}-\dfrac{8}{4}=\dfrac{5\left(x+2\right)}{4}\\ \Leftrightarrow-2x+6-8=5x+10\\ \Leftrightarrow-2x+6-8-5x-10=0\\ \Leftrightarrow-7x-12=0\\ \Leftrightarrow-7x=12\\ \Leftrightarrow x=-\dfrac{12}{7}\)
Vậy PT có tập nghiệm là S = { \(-\dfrac{12}{7}\) }
4.
\(\dfrac{2\left(2x+1\right)}{5}-\dfrac{6+x}{3}=\dfrac{5-4x}{15}\\ \Leftrightarrow\dfrac{6\left(2x+1\right)}{15}-\dfrac{5\left(6+x\right)}{15}=\dfrac{5-4x}{15}\\ \Leftrightarrow12x+6-30-5x=5-4x\\ \Leftrightarrow12x+6-30-5x-5+4x=0\\ \Leftrightarrow11x-29=0\\ \Leftrightarrow11x=29\\ \Leftrightarrow x=\dfrac{29}{11}\)
Vậy PT có tập nghiệm S = { \(\dfrac{29}{11}\) }
Hmmm tớ cx k chắc lắm
Ok cảm ơn nhiều nha
Năm mới chúc bạn hp bên gia đình và học giỏi nha

f: \(\dfrac{2}{\sqrt{6}-\sqrt{5}}=2\sqrt{6}+2\sqrt{5}\)
l: \(\dfrac{3}{\sqrt{10}+\sqrt{7}}=\sqrt{10}-\sqrt{7}\)

Quy đồng mẫu hai phân số ta có :
\(\frac{4}{7}=\frac{4\times12}{7\times12}=\frac{48}{84}\)
\(\frac{5}{12}=\frac{5\times7}{12\times7}=\frac{35}{84}\)

Chọn D
Ta có: x(3 + 5i) - y(1 + 2i) = 9 + 16i <=> (3x - y) + (5x - 2y) = 9 + 16i
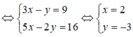
Vậy: T = |x - y| = 5
Bạn thử nhân liên hợp cả tử và mẫu theo (A+B)×(A-B)=A^2 -B^2 xem s
\(a,\dfrac{y-1}{y+1}=\dfrac{\left(y-1\right)^2}{\left(y-1\right)\left(y+1\right)};\dfrac{y+1}{y-1}=\dfrac{\left(y+1\right)^2}{\left(y-1\right)\left(y+1\right)};\dfrac{1}{y^2-1}=\dfrac{1}{\left(y-1\right)\left(y+1\right)}\\ b,\dfrac{2}{y^2-4y}=\dfrac{2\left(y+4\right)}{y\left(y-4\right)\left(y+4\right)};\dfrac{y}{y^2-16}=\dfrac{y^2}{y\left(y-4\right)\left(y+4\right)}\)