Có bao nhiêu số phức z thỏa mãn
z
-
i
=
2
v
à
z
2
là số thuần ảo ...
Đọc tiếp
Có bao nhiêu số phức z thỏa mãn z - i = 2 v à z 2 là số thuần ảo
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
PT
1
CM
30 tháng 3 2018
Đáp án C
Gọi z=a+bi
Để 
là số thuần ảo
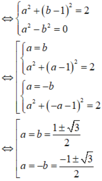
Vậy có 4 số phức thỏa mãn yêu cầu đề bài.
PT
1
CM
15 tháng 11 2017
Đáp án C.
Đặt z = x + yi x , y ∈ ℝ , ta có z 2 = x + y i 2 = x 2 - y 2 + 2 x y i là số thuần ảo
⇔ x 2 - y 2 = 0 2 x y ≠ 0 ( 1 ) .
Mặt khác z - i = 2 ⇔ x + y - 1 i = 2 ⇔ x 2 + y - 1 2 = 2 (2).
Từ (1),(2) suy ra x 2 = y 2 x 2 + y - 1 2 = 2 ⇔ x 2 = y 2 y 2 + y - 1 2 = 2 ⇒ có 4 số phức cần tìm.
PT
1
PT
1
PT
1
NV
Nguyễn Việt Lâm
Giáo viên
18 tháng 5 2021
Đặt \(z=x+yi\Rightarrow x^2+y^2=2\)
\(\left(z+2i\right)\left(\overline{z}-2\right)=\left(x+\left(y+2\right)i\right)\left(x-2-yi\right)\)
\(=x\left(x-2\right)+y\left(y+2\right)+\left[\left(x-2\right)\left(y+2\right)-xy\right]i\)
\(=x^2+y^2-2x+2y+\left(2x-2y-4\right)i\)
Số phức đã cho thuần ảo khi \(\left\{{}\begin{matrix}x^2+y^2=2\\x^2+y^2-2x+2y=0\\2x-2y-4\ne0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x^2+y^2=2\\y=x-1\\x-y-2\ne0\end{matrix}\right.\) \(\Rightarrow\left(x;y\right)=\left(\dfrac{1+\sqrt{3}}{2};\dfrac{1-\sqrt{3}}{2}\right);\left(\dfrac{1-\sqrt{3}}{2};\dfrac{1+\sqrt{3}}{2}\right)\)
Có 2 số phức thỏa mãn
PT
1
ĐÁP ÁN: C