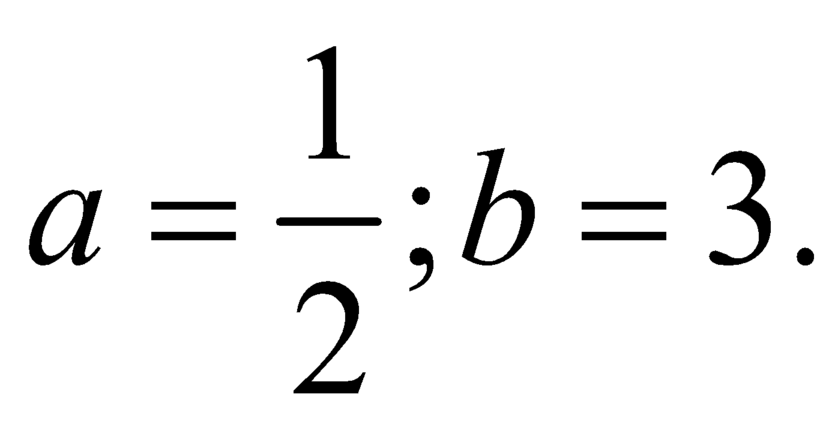Tìm tham số a, b để hàm số:
y = ( 3 a - 1 ) sin x + b cos x , k h i x < 0 a sin x + ( 3 - 2 b ) cos x , k h i x ≥ 0 l à h à m s ố l ẻ
A.![]()
B. ![]()
C. ![]()
D. ![]()
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

24.
\(cos\left(x-\dfrac{\pi}{2}\right)\le1\Rightarrow y\le3.1+1=4\)
\(y_{max}=4\)
26.
\(y=\sqrt{2}cos\left(2x-\dfrac{\pi}{4}\right)\)
Do \(cos\left(2x-\dfrac{\pi}{4}\right)\le1\Rightarrow y\le\sqrt{2}\)
\(y_{max}=\sqrt{2}\)
b.
\(\dfrac{1}{2}sinx+\dfrac{\sqrt{3}}{2}cosx=\dfrac{1}{2}\)
\(\Leftrightarrow cos\left(x-\dfrac{\pi}{6}\right)=\dfrac{1}{2}\)
\(\Leftrightarrow\left[{}\begin{matrix}x-\dfrac{\pi}{6}=\dfrac{\pi}{3}+k2\pi\\x-\dfrac{\pi}{6}=-\dfrac{\pi}{3}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\pi}{2}+k2\pi\\x=-\dfrac{\pi}{6}+k2\pi\end{matrix}\right.\)

3.
\(f\left(x+\frac{\pi}{3}\right)=cos\left(x+\frac{\pi}{3}\right)\Rightarrow f'\left(x+\frac{\pi}{3}\right)=-sin\left(x+\frac{\pi}{3}\right)\)
\(f'\left(x-\frac{\pi}{6}\right)=-sin\left(x-\frac{\pi}{6}\right)\)
\(f'\left(0\right)=-sin\left(0\right)=0\)
\(2f'\left(x+\frac{\pi}{3}\right).f'\left(x-\frac{\pi}{6}\right)=2sin\left(x+\frac{\pi}{3}\right)sin\left(x-\frac{\pi}{6}\right)\)
\(=cos\left(\frac{\pi}{2}\right)-cos\left(2x+\frac{\pi}{6}\right)=-cos\left(2x+\frac{\pi}{6}\right)\)
\(f'\left(0\right)-f\left(2x+\frac{\pi}{6}\right)=0-cos\left(2x+\frac{\pi}{6}\right)=-cos\left(2x+\frac{\pi}{6}\right)\)
\(\Rightarrow2f'\left(x+\frac{\pi}{3}\right)f'\left(x-\frac{\pi}{6}\right)=f'\left(0\right)-f\left(2x+\frac{\pi}{6}\right)\) (đpcm)
4.
\(y=3\left(sin^4x+cos^4x\right)-2\left(sin^6x+cos^6x\right)\)
\(=3\left(sin^2x+cos^2x\right)^2-6sin^2x.cos^2x-2\left(sin^2x+cos^2x\right)^3+6sin^2x.cos^2x\left(sin^2x+cos^2x\right)\)
\(=3-2=1\)
\(\Rightarrow y'=0\) ; \(\forall x\)
5.
\(y=\left(\frac{sinx}{1+cosx}\right)^3=\left(\frac{sinx\left(1-cosx\right)}{1-cos^2x}\right)^3=\left(\frac{sinx\left(1-cosx\right)}{sin^2x}\right)^3=\left(\frac{1-cosx}{sinx}\right)^3\)
\(y'=3\left(\frac{1-cosx}{sinx}\right)^2\left(\frac{sin^2x-cosx\left(1-cosx\right)}{sin^2x}\right)=3\left(\frac{1-cosx}{sinx}\right)^2\left(\frac{1-cosx}{sin^2x}\right)=\frac{3\left(1-cosx\right)^3}{sin^4x}\)
\(\Rightarrow y'.sinx-3y=\frac{3\left(1-cosx\right)^3}{sin^3x}-3\left(\frac{1-cosx}{sinx}\right)^3=0\) (đpcm)

Giá trị của \(f\left(-x\right)\) và \(f\left(x\right)\) khi \(x=0\) phải bằng nhau
Bạn thay \(x=0\) vào 2 biểu thức chứa dấu "=" là ra đẳng thức đó thôi
\(f\left(-x\right)=\left\{{}\begin{matrix}\left(1-3a\right)sinx+b.cosx,khi.x>0\\-a.sinx+\left(3-2b\right)cosx,khi.x\le0\end{matrix}\right.\)
Hàm đã cho là hàm lẻ khi và chỉ khi:
\(\left\{{}\begin{matrix}b=3-2b\\\left(3a-1\right)sinx+b.cosx=-a.sinx+\left(3-2b\right)cosx\\a.sinx+\left(3-2b\right)cosx=\left(1-3a\right)sinx+b.cosx\end{matrix}\right.\) \(\forall x\)
\(\Leftrightarrow\left\{{}\begin{matrix}b=1\\\left(4a-1\right)sinx+\left(3b-3\right)cosx=0\\\left(4a-1\right)sinx+\left(3-3b\right)cosx=0\end{matrix}\right.\) ;\(\forall x\)
\(\Leftrightarrow\left\{{}\begin{matrix}b=1\\4a-1=0\\3b-3=0\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}a=\frac{1}{4}\\b=1\end{matrix}\right.\)

a.
\(-1\le sin\left(1-x^2\right)\le1\)
\(\Rightarrow y_{min}=-1\) khi \(1-x^2=-\dfrac{\pi}{2}+k2\pi\) \(\Rightarrow x^2=\dfrac{\pi}{2}+1+k2\pi\) (\(k\ge0\))
\(y_{max}=1\) khi \(1-x^2=\dfrac{\pi}{2}+k2\pi\Rightarrow x^2=1-\dfrac{\pi}{2}+k2\pi\) (\(k\ge1\))
b.
Đặt \(\sqrt{2-x^2}=t\Rightarrow t\in\left[0;\sqrt{2}\right]\subset\left[0;\pi\right]\)
\(y=cost\) nghịch biến trên \(\left[0;\pi\right]\Rightarrow\) nghịch biến trên \(\left[0;\sqrt{2}\right]\)
\(\Rightarrow y_{max}=y\left(0\right)=cos0=1\) khi \(x^2=2\Rightarrow x=\pm\sqrt{2}\)
\(y_{min}=y\left(\sqrt{2}\right)=cos\sqrt{2}\) khi \(x=0\)
Đáp án A
TXĐ: D = R. Suy ra
Với x , 0 thì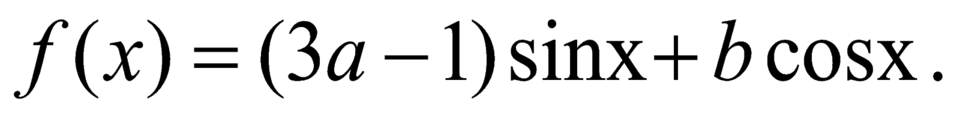
Để hàm số lẻ thì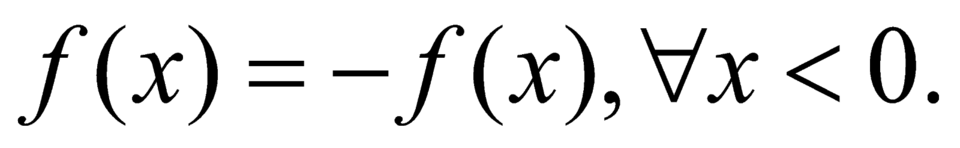
Từ đó suy ra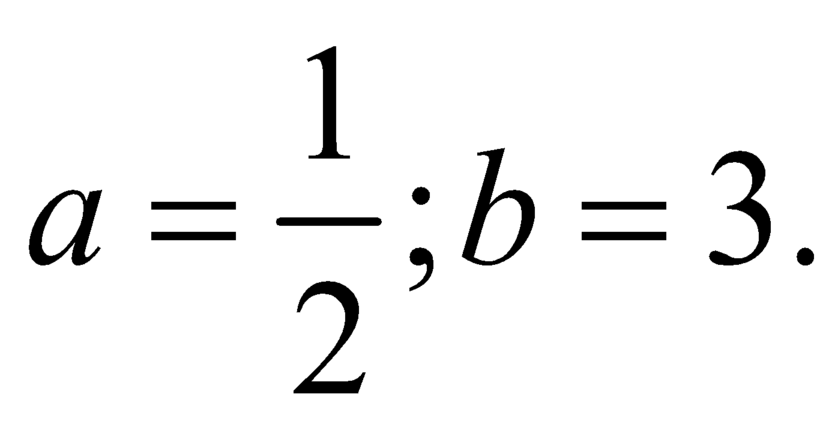
Với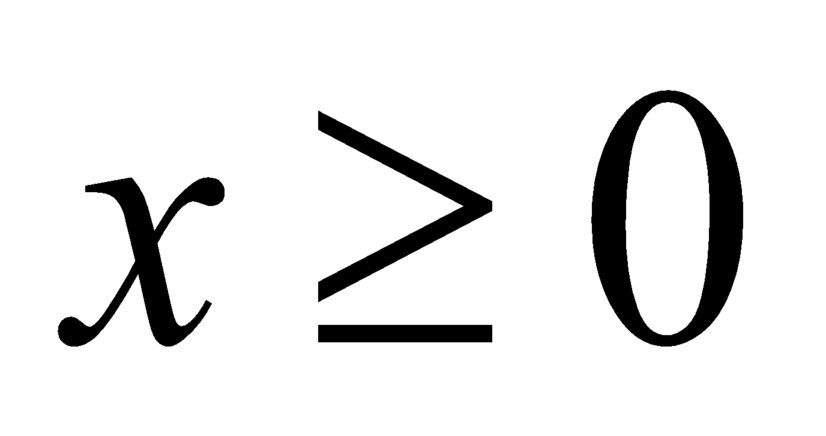 thì
thì 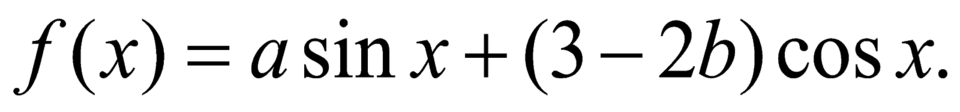
Hàm số lẻ nên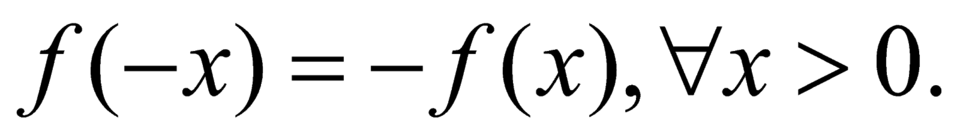
Từ đó sy ra