Cho hai hàm số f(x) , g(x) đều có đạo hàm trên ℝ và thỏa mãn: f 3 2 − x − 2 f 2 2 3 x x 2...

PT

Pham Trong Bach

20 tháng 1 2017

Cho hai hàm số f(x) , g(x) đều có đạo hàm trên ℝ và thỏa mãn: f 3 2 − x − 2 f 2 2 + 3 x + x 2 . g x + 36 x = 0 ∀ x ∈ ℝ . Tính A = 3 f 2 + 4 f ' 2 A. 11 B. 13 C. 14 D....

Đọc tiếp

#Mẫu giáo

1

CM

Cao Minh Tâm

20 tháng 1 2017

Đáp án D

f 3 2 − x − 2 f 2 2 + 3 x + x 2 . g x + 36 x = 0 ∀ x ∈ ℝ 1

− 3 f 2 2 − x . f ' 2 − x − 12 f 2 + 3 x . f ' 2 + 3 x + 2 x . g x + x 2 . g ' x + 36 = 0 ∀ x ∈ ℝ

Đúng(0)

PT

Pham Trong Bach

29 tháng 3 2017

Cho hàm số y = f(x) có đạo hàm trên ℝ , thỏa mãn f(2) = f(-2) =2019. Hàm số y = f'(x) có đồ thị hàm số như hình vẽ. Hỏi hàm số g(x)= f x - 2019 2 (1;2). Ngịch biến trên khoảng nào dưới đây A . 1 ; 2 B . - 2 ; 2 C . 2 ; + ∞ D . - 2 ; - 1 ...

Đọc tiếp

#Toán lớp 12

1

CM

Cao Minh Tâm

29 tháng 3 2017

Đúng(0)

PT

Pham Trong Bach

13 tháng 9 2018

Cho hàm số y=f(x) có đạo hàm liên tục trên R thỏa mãn x f ( x ) . f ' ( x ) = f 2 ( x ) - x , ∀ x ∈ ℝ và f(2)=1 .Tích phân bằng A. 3 2 B. 4 3 C. 2 D....

Đọc tiếp

#Toán lớp 12

1

CM

Cao Minh Tâm

13 tháng 9 2018

Chọn đáp án C.

Lấy tích phân hai vế trên đoạn [0;2] có

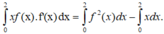
Tích phân từng phần có

Đúng(0)

PT

Pham Trong Bach

7 tháng 6 2019

Cho hàm số y = f(x) xác định trên ℝ và có đạo hàm f '(x) thỏa mãn f ' x = 1 - x x + 2 . g x + 2018 trong đó g x < 0 , ∀ x ∈ ℝ . Hàm số y = f 1 - x + 2018 x + 2019 nghịch biến trên khoảng nào? A. 1 ; + ∞ B. 0 ; 3 ...

Đọc tiếp

#Mẫu giáo

1

CM

Cao Minh Tâm

7 tháng 6 2019

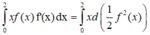
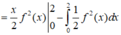
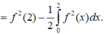
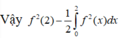
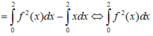
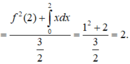
Đáp án D

Ta có y ' = f 1 - x + 2018 x + 2019 ' = 1 - x ' . f ' 1 - x + 2018 = - f ' 1 - x + 2018

= - x 3 - x . g 1 - x - 2018 + 2018 = - x 3 - x . g 1 - x mà g 1 - x < 0 ; ∀ x ∈ ℝ

Nên y ' < 0 ⇔ - x 3 - x . g 1 - x < 0 ⇔ x 3 - x . g 1 - x > 0 ⇔ x 3 - x < 0 ⇔ [ x > 3 x < 0

Khi đó, hàm số y = f 1 - x + 2018 x + 2019 nghịch biến trên khoảng 3 ; + ∞

Đúng(0)

PT

Pham Trong Bach

25 tháng 11 2017

Cho hàm số y = f(x) có đạo hàm trên ℝ , thỏa mãn f(2) = f(-2) = 2019. Hàm số y = f'(x) có đồ thị như hình vẽ. Hỏi hàm số g x = f x - 2019 2 (1;2) nghịch biến trên khoảng nào dưới đây? ...

Đọc tiếp

#Toán lớp 12

1

CM

Cao Minh Tâm

25 tháng 11 2017

Đúng(0)

PT

Pham Trong Bach

17 tháng 1 2019

Cho hàm số f(x) có đạo hàm liên tục trên và thỏa mãn f ( x ) > 0 , ∀ ∈ ℝ . Biết f(0) = 1 và f ' x f x = 2 - 2 x . Tìm các giá trị thực của tham số m để phương trình f(x) = m có hai nghiệm thực phân biệt. A. m > e B. 0 < m ≤ 1 C. 0 < m < e D. 1 < m <...

Đọc tiếp

#Mẫu giáo

1

CM

Cao Minh Tâm

17 tháng 1 2019

Đáp án C

Với f x > 0 , ∀ x ∈ ℝ . Xét biểu thức f ' x f x = 2 - 2 x *

Lấy nguyên hàm 2 vế (*), ta được ∫ d f x f x = ∫ 2 - 2 x d x

⇔ ∫ d f x f x = - x 2 + 2 x + C ⇔ ln f x = - x 2 + 2 x + C

Mà f(0) =1 suy ra C = lnf(0) = ln1 = 0. Do đó f x = e - x 2 + 2 x

Xét hàm số f x = e - x 2 + 2 x trên - ∞ ; + ∞ , có f ' x = - 2 x + 2 = 0 ⇔ x = 1

Tính giá trị f 1 = e ; lim x → - ∞ f x = 0 ; lim x → - ∞ f x = 0

Suy ra để phương trình f(x) = m có hai nghiệm thực phân biệt ⇔ 0 < m < e .

Đúng(0)

B

B.Trâm

4 tháng 4 2021

1. Cho hàm số \(y=x^3-3mx^2+3\left(2m-1\right)x+1\) . Với giá trị nào của m thì \(f'\left(x\right)-6x0\) với mọi x>2A. m > 1/2 B. m < -1/2 C. m >1 D. m ≤ 02. Cho hai hàm số f(x) và g(x) đều có đạo hàm trên R và thỏa mãn : \(f^3\left(2-x\right)-2f^2\left(2+3x\right)+x^2g\left(x\right)+36x=0\) với mọi x thuộc R.Tính \(A=3f\left(2\right)+4f'\left(2\right)\)3. Biết hàm số f(x) - f(2x) có đạo hàm bằng 18 tại x=1 và đạo hàm bằng...

Đọc tiếp

#Toán lớp 11

3

NV

Nguyễn Việt Lâm

Giáo viên

4 tháng 4 2021

1.

\(f'\left(x\right)=3x^2-6mx+3\left(2m-1\right)\)

\(f'\left(x\right)-6x=3x^2-3.2\left(m+1\right)x+3\left(2m-1\right)>0\)

\(\Leftrightarrow x^2-2\left(m+1\right)x+2m-1>0\)

\(\Leftrightarrow x^2-2x-1>2m\left(x-1\right)\)

Do \(x>2\Rightarrow x-1>0\) nên BPT tương đương:

\(\dfrac{x^2-2x-1}{x-1}>2m\Leftrightarrow\dfrac{\left(x-1\right)^2-2}{x-1}>2m\)

Đặt \(t=x-1>1\Rightarrow\dfrac{t^2-2}{t}>2m\Leftrightarrow f\left(t\right)=t-\dfrac{2}{t}>2m\)

Xét hàm \(f\left(t\right)\) với \(t>1\) : \(f'\left(t\right)=1+\dfrac{2}{t^2}>0\) ; \(\forall t\Rightarrow f\left(t\right)\) đồng biến

\(\Rightarrow f\left(t\right)>f\left(1\right)=-1\Rightarrow\) BPT đúng với mọi \(t>1\) khi \(2m< -1\Rightarrow m< -\dfrac{1}{2}\)

Đúng(1)

NV

Nguyễn Việt Lâm

Giáo viên

4 tháng 4 2021

2.

Thay \(x=0\) vào giả thiết:

\(f^3\left(2\right)-2f^2\left(2\right)=0\Leftrightarrow f^2\left(2\right)\left[f\left(2\right)-2\right]=0\Rightarrow\left[{}\begin{matrix}f\left(2\right)=0\\f\left(2\right)=2\end{matrix}\right.\)

Đạo hàm 2 vế giả thiết:

\(-3f^2\left(2-x\right).f'\left(2-x\right)-12f\left(2+3x\right).f'\left(2+3x\right)+2x.g\left(x\right)+x^2.g'\left(x\right)+36=0\) (1)

Thế \(x=0\) vào (1) ta được:

\(-3f^2\left(2\right).f'\left(2\right)-12f\left(2\right).f'\left(2\right)+36=0\)

\(\Leftrightarrow f^2\left(2\right).f'\left(2\right)+4f\left(2\right).f'\left(2\right)-12=0\) (2)

Với \(f\left(2\right)=0\) thế vào (2) \(\Rightarrow-12=0\) ko thỏa mãn (loại)

\(\Rightarrow f\left(2\right)=2\)

Thế vào (2):

\(4f'\left(2\right)+8f'\left(2\right)-12=0\Leftrightarrow f'\left(2\right)=1\)

\(\Rightarrow A=3.2+4.1\)

Đúng(1)

QA

Quỳnh Anh

21 tháng 4 2022

Cho hai hàm số \(f\left(x\right),g\left(x\right)\) đều có đạo hàm trên R và thỏa mãn: \(f^3\left(2-x\right)-2f^2\left(2+3x\right)+x^2.g\left(x\right)+36x=0\forall x\in R\). Tính \(A=3f\left(2\right)+4f'\left(2\right)\)

A. A = -10

B. A = 10

C. A = 1

D. A = 9

#Toán lớp 11

1

NV

Nguyễn Việt Lâm

Giáo viên

21 tháng 4 2022

\(f^3\left(2-x\right)-2f^2\left(2+3x\right)+x^2g\left(x\right)+36x=0\) (1)

Thay \(x=0\Rightarrow f^3\left(2\right)-2f^2\left(2\right)=0\Rightarrow\left[{}\begin{matrix}f\left(2\right)=0\\f\left(2\right)=2\end{matrix}\right.\)

Đạo hàm 2 vế của (1):

\(\Rightarrow-3f^2\left(2-x\right).f'\left(2-x\right)-12f\left(2+3x\right).f'\left(2+3x\right)+2x.g\left(x\right)+x^2.g'\left(x\right)+36=0\)

Thay \(x=0\)

\(\Rightarrow-3f^2\left(2\right).f'\left(2\right)-12f\left(2\right).f'\left(2\right)+36=0\)

TH1: \(f\left(2\right)=0\Rightarrow36=0\) (ktm)

TH2: \(f\left(2\right)=2\)

\(\Rightarrow-3.2^2.f'\left(2\right)-12.2.f'\left(2\right)+36=0\Rightarrow f'\left(2\right)=1\)

\(\Rightarrow A=3.2+4.1=10\)

Đúng(1)

PT

Pham Trong Bach

9 tháng 2 2019