Giải giúp mk với mk cần gấp
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Câu 1.
Công suất của bếp điện:
\(P=\dfrac{U^2}{R}=\dfrac{220^2}{48,4}=1000W\)
Câu 2.
a)Đổi: \(341mA=341\cdot10^{-3}A\)
Điện trở đèn: \(R=\dfrac{U}{I}=\dfrac{220}{341\cdot10^{-3}}\approx645,16\Omega\)
Công suất đèn: \(P=U.I=220\cdot341\cdot10^{-3}=75,02W\)
b)Điện năng tiêu thụ của đèn: \(A=UIt=Pt=75,02\cdot30\cdot4\cdot3600=32408640J=9,0024kWh\)
Số đếm tương ứng của công tơ điện là 9,0024 số điện.
Bài 2 tính công thực hiện của bạn nhân 4 chứ không phải nhân 24 nhé.


a: \(=\dfrac{1}{3}-\dfrac{17}{6}+\dfrac{4}{3}=\dfrac{5}{3}-\dfrac{17}{6}=\dfrac{10-17}{6}=\dfrac{-7}{7}\)
b: \(=\dfrac{5+6}{12}=\dfrac{11}{12}\)
c: \(=\dfrac{-12+7}{28}\cdot\dfrac{28}{15}=\dfrac{-5}{15}=\dfrac{-1}{3}\)
d: \(=\dfrac{2}{3}+\dfrac{1}{5}-\dfrac{4}{15}=\dfrac{10+3-4}{15}=\dfrac{9}{15}=\dfrac{3}{5}\)
e: \(=\dfrac{-3}{16}\left(\dfrac{8}{15}+\dfrac{7}{15}\right)-\dfrac{5}{16}=\dfrac{-3-5}{16}=\dfrac{-1}{2}\)
f: \(=\dfrac{-20}{23}-\dfrac{2}{23}+\dfrac{2}{3}+\dfrac{2}{5}+\dfrac{7}{15}\)
\(=-1+\dfrac{10+6+7}{15}=\dfrac{-15+23}{15}=\dfrac{8}{15}\)
g: =5/7(5/11+2/11-14/11)
=-7/11*5/7=-5/11
h: =-5/7(10/13+3/13)+1+5/7
=-5/7+1+5/7
=1
i: \(=\dfrac{7}{4}\left(\dfrac{29}{5}-\dfrac{9}{5}\right)+3+\dfrac{2}{13}=7+3+\dfrac{2}{13}=10+\dfrac{2}{13}=\dfrac{132}{13}\)



5.
\(n_{Na}=a\left(mol\right)\)
\(Na+H_2O\rightarrow NaOH+\dfrac{1}{2}H_2\)
\(a...............a.....0.5a\)
\(m_{\text{dung dịch sau phản ứng}}=23a+500-0.5a\cdot2=22a+500\left(g\right)\)
\(m_{NaOH}=40a\left(g\right)\)
\(C\%_{NaOH}=\dfrac{40a}{22a+500}\cdot100\%=20\%\)
\(\Rightarrow a=2.8\)
\(b.\)
\(n_{H^+}=10^{-3}\cdot V\cdot\left(1+0.5\cdot2\right)=2\cdot10^{-3}V\left(mol\right)\)
\(\Rightarrow n_{NaOH}=2\cdot10^{-3}V=2.8\left(mol\right)\)
\(\Rightarrow V=1400\)

ĐK: \(\left\{{}\begin{matrix}x\ne-y\\y\ge\dfrac{3}{2}\end{matrix}\right.\).
\(\left\{{}\begin{matrix}\dfrac{2x-y+3}{x+y}=1\\2x-\sqrt{2y-3}=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}\dfrac{2x-y+3}{x+y}-1=0\\2x-\sqrt{2y-3}=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}\dfrac{2x-y+3}{x+y}-\dfrac{x+y}{x+y}=0\\2x-\sqrt{2y-3}=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}2x-y+3-x-y=0\\2x-\sqrt{2y-3}=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x-2y+3=0\\2x-\sqrt{2y-3}=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x-\left(2y-3\right)=0\\2x-\sqrt{2y-3}=0\end{matrix}\right..\)
Đặt a = x, b = \(\sqrt{2y-3}\).
Hệ phương trình trở thành: \(\left\{{}\begin{matrix}a-b^2=0\\2a-b=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}a=b^2\\2b^2-b=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}a=b^2\\b\left(2b-1\right)=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}a=b^2\\\left[{}\begin{matrix}b=0\\b=\dfrac{1}{2}\end{matrix}\right.\end{matrix}\right.\left\{{}\begin{matrix}\left[{}\begin{matrix}a=0\\a=\dfrac{1}{4}\end{matrix}\right.\\\left[{}\begin{matrix}b=0\\b=\dfrac{1}{2}\end{matrix}\right.\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}\left[{}\begin{matrix}x=0\\x=\dfrac{1}{4}\end{matrix}\right.\\\left[{}\begin{matrix}y=\dfrac{3}{2}\\2y-3=\dfrac{1}{4}\end{matrix}\right.\end{matrix}\right.\left\{{}\begin{matrix}\left[{}\begin{matrix}x=0\\x=\dfrac{1}{4}\end{matrix}\right.\\\left[{}\begin{matrix}y=\dfrac{3}{2}\\2y=\dfrac{13}{4}\end{matrix}\right.\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}\left[{}\begin{matrix}x=0\\x=\dfrac{1}{4}\end{matrix}\right.\\\left[{}\begin{matrix}y=\dfrac{3}{2}\\y=\dfrac{13}{8}\end{matrix}\right.\end{matrix}\right..\)
Vậy hệ phương trình có nghiệm (x;y) \(\in\) \(\left\{\left(0;\dfrac{3}{2}\right),\left(\dfrac{1}{4};\dfrac{13}{8}\right)\right\}\).


a, \(2\sqrt{3}-\sqrt{4+x^2}=0\Leftrightarrow\sqrt{4+x^2}=2\sqrt{3}\)
\(\Leftrightarrow x^2+4=12\Leftrightarrow x^2=8\Leftrightarrow x=\pm2\sqrt{2}\)
b, \(\sqrt{16x+16}-\sqrt{9x+9}=0\)ĐK : x >= -1
\(\Leftrightarrow4\sqrt{x+1}-3\sqrt{x+1}=0\Leftrightarrow\sqrt{x+1}=0\Leftrightarrow x+1=0\Leftrightarrow x=-1\)
c, \(\sqrt{4\left(x+2\right)^2}=8\Leftrightarrow2\left|x+2\right|=8\Leftrightarrow\left|x+2\right|=4\)
TH1 : \(x+2=4\Leftrightarrow x=2\)
TH2 : \(x+2=-4\Leftrightarrow x=-6\)
c: Ta có: \(\sqrt{4\left(x+2\right)^2}=8\)
\(\Leftrightarrow\left|x+2\right|=4\)
\(\Leftrightarrow\left[{}\begin{matrix}x+2=4\\x+2=-4\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=2\\x=-6\end{matrix}\right.\)

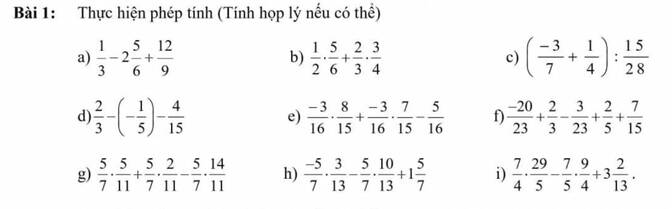 Giải giúp mk với mk đang cần gấp
Giải giúp mk với mk đang cần gấp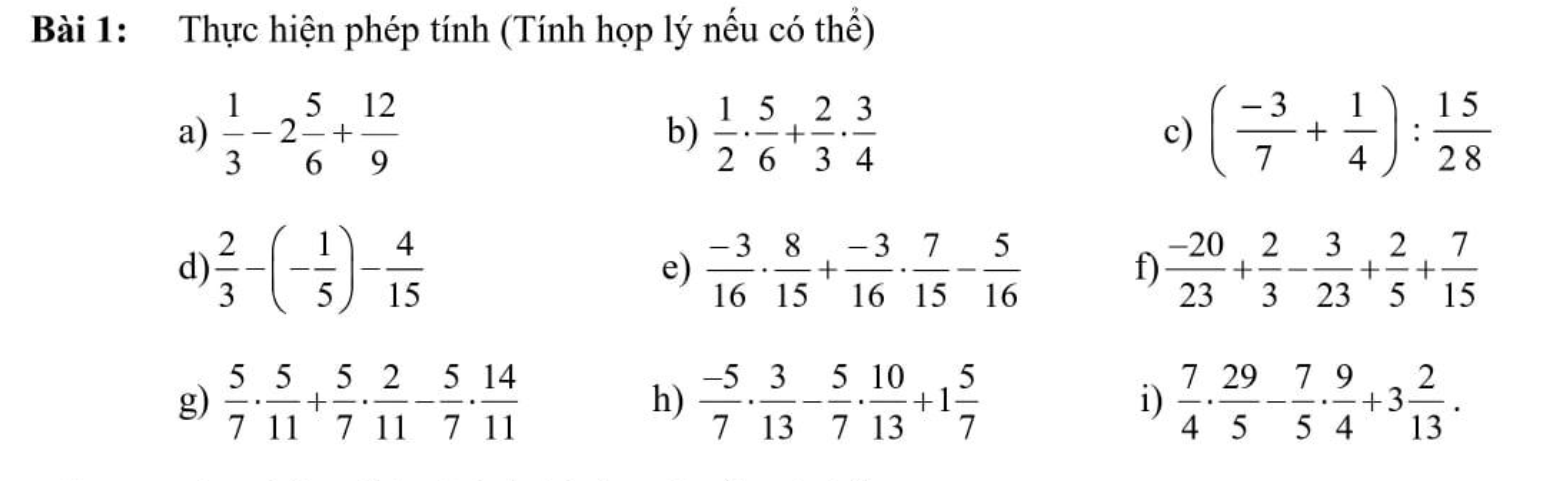
 giúp mk với, mk cần gấp ạ. camon mn rất nhìu, giải chi tiết dễ hiểu giúp mk nha
giúp mk với, mk cần gấp ạ. camon mn rất nhìu, giải chi tiết dễ hiểu giúp mk nha


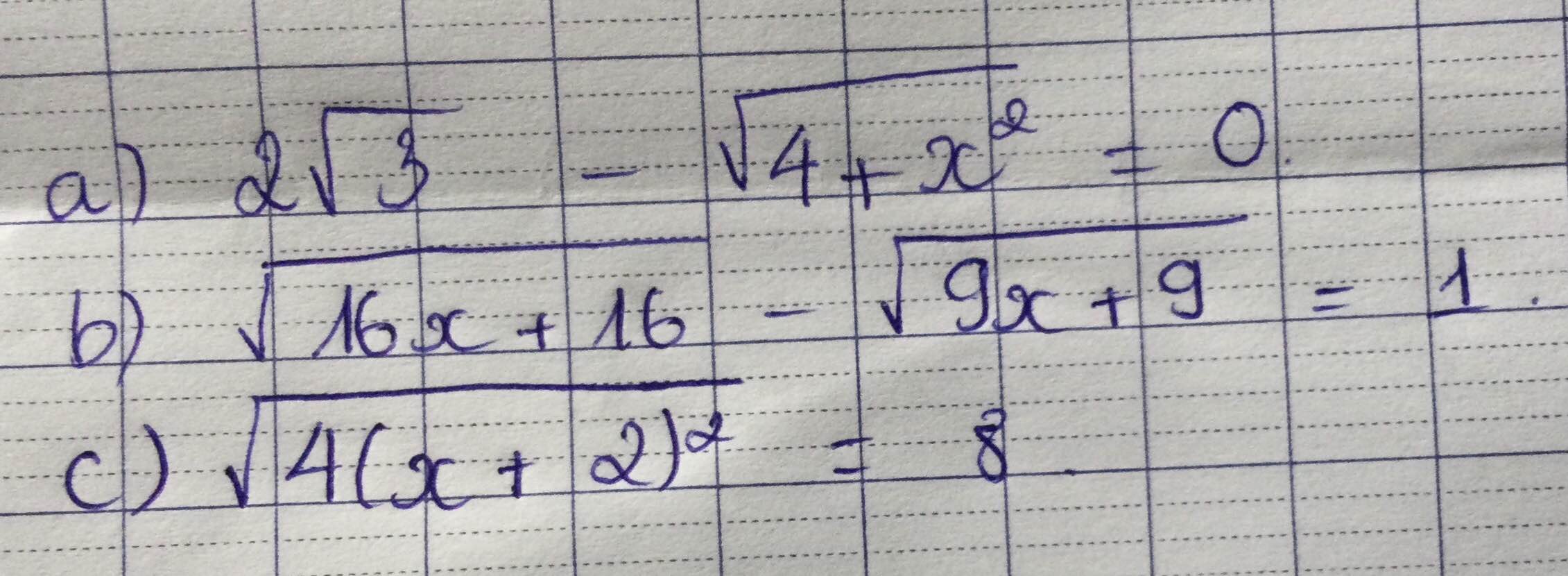 Các bạn ơi giúp mk với. Mk cần gấp lắm ạ.Các bạn giải chi tiết giúp mk nhé . Mk hứa sẽ vote 5* cho . Mk cảm ơn nhiều.
Các bạn ơi giúp mk với. Mk cần gấp lắm ạ.Các bạn giải chi tiết giúp mk nhé . Mk hứa sẽ vote 5* cho . Mk cảm ơn nhiều.
A
C
C
B
B