Cho phương trình : \(sin^2x+cosx-1=0\left(1\right)\) . Bằng cách đặt \(t=cosx\left(-1\le t\le1\right)\) thì phương trình (1) trở thành phương trình nào sau đây ?
A. \(-t^2+t=0\)
B. \(-2t^2+t=0\)
C. \(-t^2+t-2=0\)
D. \(t^2+t-2=0\)
Trình bày bài làm chi tiết rồi ms chọn đáp án nha các bạn

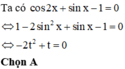


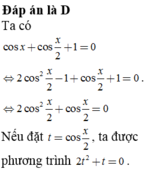
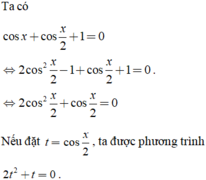
help me
\(\Leftrightarrow1-cos^2x+cosx-1=0\)
\(\Leftrightarrow-cos^2x+cosx=0\)
Đặt \(cosx=t\)
\(\Rightarrow-t^2+t=0\)