Tùm giá trị nhỏ nhất của:A=50+|x-5|+|y-3|
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
TA
23 tháng 7 2021
1) `(x-3)^4 >=0`
`2.(x-3)^4>=0`
`2.(x-3)^4-11 >=-11`
`=> A_(min)=-11 <=> x-3=0<=>x=3`
2) `|5-x|>=0`
`-|5-x|<=0`
`-3-|5-x|<=-3`
`=> B_(max)=-3 <=>x=5`.
24 tháng 7 2021
Bài 1:
Ta có: \(\left(x-3\right)^4\ge0\forall x\)
\(\Leftrightarrow2\left(x-3\right)^4\ge0\forall x\)
\(\Leftrightarrow2\left(x-3\right)^4-11\ge-11\forall x\)
Dấu '=' xảy ra khi x=3
DD
1
30 tháng 6 2021
$a)ĐK:8x+2\ge 0$
$\to 8x \ge -2$
$\to x \ge -\dfrac14$
$b)ĐK:\dfrac{-5}{6-3x} \ge 0(x \ne 2)$
Mà $-5<0$
$\to 6-3x<0$
$\to 6<3x$
$\to x>2$
$*A=x-2\sqrt{x-2}+3(x \ge 2)$
$=x-2-2\sqrt{x-2}+1+4$
$=(\sqrt{x-2}-1)^2+4 \ge 4$
Dấu "=" xảy ra khi $\sqrt{x-2}-1=0 \Leftrightarrow \sqrt{x-2}=1\Leftrightarrow x=3$
HD
Tìm giá trị nhỏ nhất của:A=/2.5-x/+5,8
Tìm giá trị lớn nhất của:B=2-/x+2/3/ (là 2 phần 3 nha mấy bạn)
1
LT
6 tháng 7 2017
ta có: /2,5-x/\(\ge\)0, nên A= /2,5-x/ + 5,8 \(\ge\)5,8
vậy giá trị nn của A là 5,8, A=5,8 khi /2,5-x/=0
<=> x=2,5
ta có: /x+2/3/ \(\ge\)0 nên B= 2 - /x+2/3/ \(\le\)2
vậy gtln của B là 2, B=2 khi /x+2/3/=0 <=> x= -2/3
TL
1
24 tháng 4 2022
\(A=\dfrac{27-12x}{x^2+9}=\dfrac{x^2-12x+36-\left(x^2+9\right)}{x^2+9}=\dfrac{\left(x-6\right)^2}{x^2+9}-1\ge-1\)
\(A_{min}=-1\Leftrightarrow x=6\)
\(A=\dfrac{27-12x}{x^2+9}=\dfrac{4\left(x^2+9\right)-\left(4x^2+12x+9\right)}{x^2+9}=4-\dfrac{\left(2x+3\right)^2}{x^2+9}\le4\)
\(A_{max}=4\Leftrightarrow x=\dfrac{-3}{2}\)
LB
3
18 tháng 7 2021
\(A=\left|x-2\right|+\dfrac{4}{7}\ge\dfrac{4}{7}\)
dấu"=" xảy ra \(< =>x=2\)
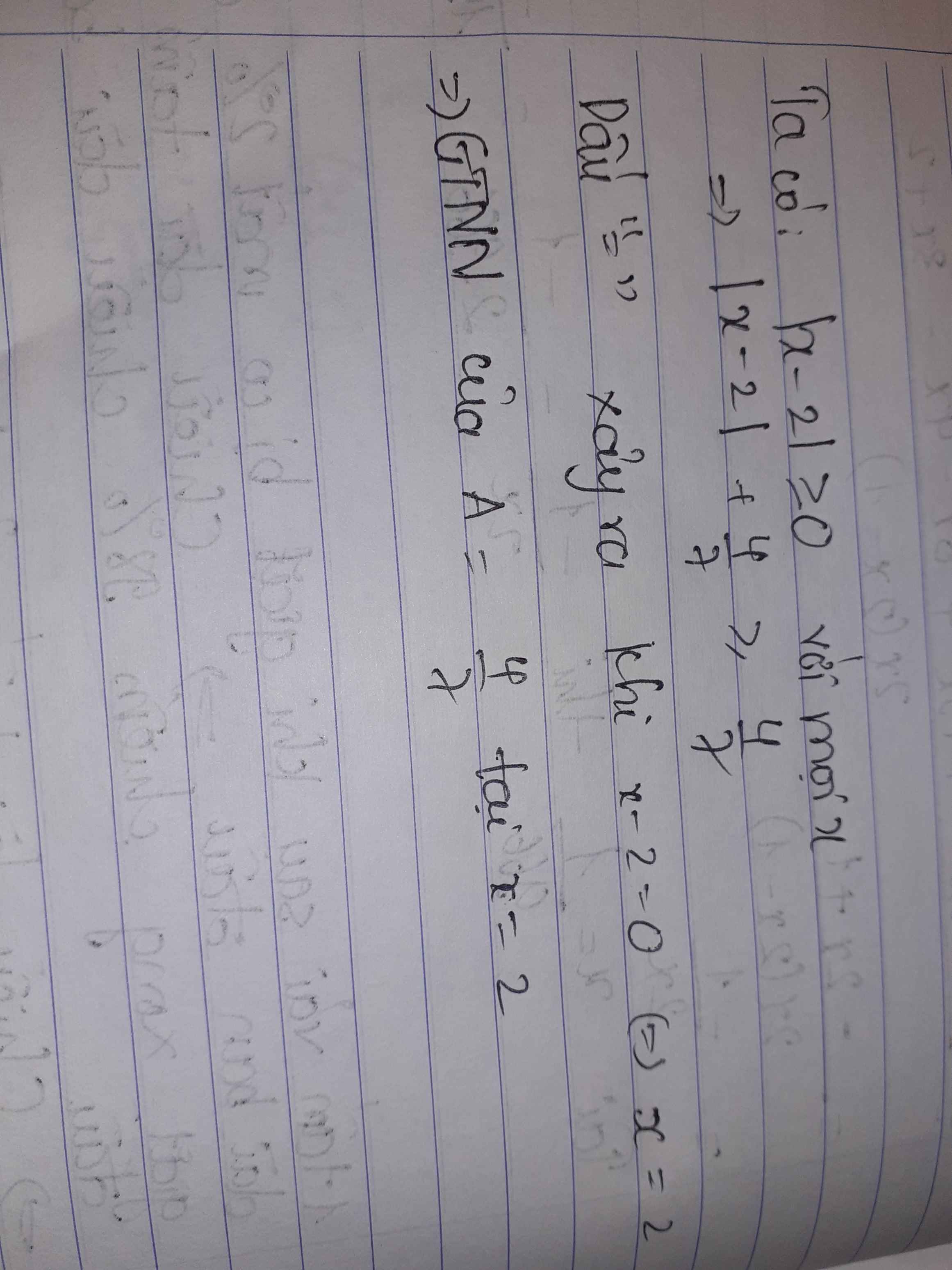
Ta có : \(\left|x-5\right|\ge0\forall x\)
\(\left|y-3\right|\ge0\forall y\)
\(\Rightarrow50+\left|x-5\right|+\left|y-3\right|\ge50\)
\(\Rightarrow\text{ Min A = 50}\)
Dấu "=" xảy ra khi
\(\hept{\begin{cases}\left|x-5\right|=0\\\left|y-3\right|=0\end{cases}\Rightarrow\hept{\begin{cases}x-5=0\\y-3=0\end{cases}\Rightarrow}\hept{\begin{cases}x=5\\y=3\end{cases}}}\)
Vậy GTNN của A = 50 khi \(\hept{\begin{cases}x=5\\y=3\end{cases}}\)