Giải pt bằng cách đặt 1 ẩn phụ
x2+\(\sqrt{x+1}=1\)
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


ĐKXĐ : \(1\le x\le3\)
\(x-\sqrt{x-1}-3=0\)
\(\Leftrightarrow\left(x-1\right)-\sqrt{x-1}-2=0\)
Đặt \(t=\sqrt{x-1},t\ge0\), suy ra pt trên trở thành \(t^2-t-2=0\Leftrightarrow\left(t-2\right)\left(t+1\right)=0\Leftrightarrow\orbr{\begin{cases}t=2\left(\text{nhận}\right)\\t=-1\left(\text{loại}\right)\end{cases}}\)
Với t = 2 suy ra x = 5

3.(x2 + x)2 – 2(x2 + x) – 1 = 0 (1)
Đặt t = x2 + x,
Khi đó (1) trở thành : 3t2 – 2t – 1 = 0 (2)
Giải (2) : Có a = 3 ; b = -2 ; c = -1
⇒ a + b + c = 0
⇒ (2) có hai nghiệm t1 = 1; t2 = c/a = -1/3.
+ Với t = 1 ⇒ x2 + x = 1 ⇔ x2 + x – 1 = 0 (*)
Có a = 1; b = 1; c = -1 ⇒ Δ = 12 – 4.1.(-1) = 5 > 0
(*) có hai nghiệm
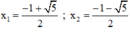
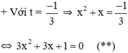
Có a = 3; b = 3; c = 1 ⇒ Δ = 32 – 4.3.1 = -3 < 0
⇒ (**) vô nghiệm.
Vậy phương trình (1) có tập nghiệm 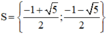

Lời giải:
ĐK: $x\geq 0$
Đặt $\sqrt{x+1}=a; \sqrt{x}=b$. ĐK $a,b\geq 0$ thì ta có:
$a-b-ab=a^2-2b^2$
$\Leftrightarrow a-b=a^2+ab-2b^2=(a-b)(a+2b)$
$\Leftrightarrow (a-b)(a+2b-1)=0$
$\Leftrightarrow a=b$ hoặc $a+2b=1$
Nếu $a=b\Rightarrow a^2=b^2\Leftrightarrow x+1=x$ (vô lý)
Nếu $a+2b=1$
$\Leftrightarrow \sqrt{x+1}-1+2\sqrt{x}=0$
$\Leftrightarrow \frac{x}{\sqrt{x+1}+1}+2\sqrt{x}=0$
$\Leftrightarrow \sqrt{x}(\frac{\sqrt{x}}{\sqrt{x+1}+1}+2)=0$
Dễ thấy biểu thức trong ngoặc lớn hơn $0$ nên \sqrt{x}=0$
$\Leftrightarrow x=0$
Vậy.......

Đặt \(\dfrac{x}{\sqrt{4x-1}}=a\)
Theo đề, ta có phương trình:
a+1/a=2
\(\Leftrightarrow a+\dfrac{1}{a}=2\)
\(\Leftrightarrow\dfrac{a^2+1-2a}{a}=0\)
=>a=1
=>\(x=\sqrt{4x-1}\)
\(\Leftrightarrow\left\{{}\begin{matrix}x^2=4x-1\\x>=\dfrac{1}{4}\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}\left(x-2\right)^2=3\\x>=\dfrac{1}{4}\end{matrix}\right.\Leftrightarrow x\in\left\{2+\sqrt{3};2-\sqrt{3}\right\}\)

a)
3 · x 2 + x 2 - 2 x 2 + x - 1 = 0 ( 1 )
Đặt t = x 2 + x ,
Khi đó (1) trở thành : 3 t 2 – 2 t – 1 = 0 ( 2 )
Giải (2) : Có a = 3 ; b = -2 ; c = -1
⇒ a + b + c = 0
⇒ (2) có hai nghiệm t 1 = 1 ; t 2 = c / a = - 1 / 3 .
+ Với t = 1 ⇒ x 2 + x = 1 ⇔ x 2 + x – 1 = 0 ( * )
Có a = 1; b = 1; c = -1 ⇒ Δ = 1 2 – 4 . 1 . ( - 1 ) = 5 > 0
(*) có hai nghiệm
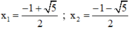
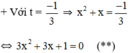
Có a = 3; b = 3; c = 1 ⇒ Δ = 3 2 – 4 . 3 . 1 = - 3 < 0
⇒ (**) vô nghiệm.
Vậy phương trình (1) có tập nghiệm 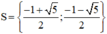
b)
x 2 − 4 x + 2 2 + x 2 − 4 x − 4 = 0 ⇔ x 2 − 4 x + 2 2 + x 2 − 4 x + 2 − 6 = 0 ( 1 )
Đặt x 2 – 4 x + 2 = t ,
Khi đó (1) trở thành: t 2 + t – 6 = 0 ( 2 )
Giải (2): Có a = 1; b = 1; c = -6
⇒ Δ = 1 2 – 4 . 1 . ( - 6 ) = 25 > 0
⇒ (2) có hai nghiệm
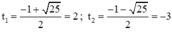
+ Với t = 2 ⇒ x 2 – 4 x + 2 = 2
⇔ x 2 – 4 x = 0
⇔ x(x – 4) = 0
⇔ x = 0 hoặc x = 4.
+ Với t = -3 ⇒ x 2 – 4 x + 2 = - 3
⇔ x2 – 4x + 5 = 0 (*)
Có a = 1; b = -4; c = 5 ⇒ Δ ’ = ( - 2 ) 2 – 1 . 5 = - 1 < 0
⇒ (*) vô nghiệm.
Vậy phương trình ban đầu có tập nghiệm S = {0; 4}.
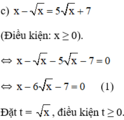
Khi đó (1) trở thành: t 2 – 6 t – 7 = 0 ( 2 )
Giải (2): Có a = 1; b = -6; c = -7
⇒ a – b + c = 0
⇒ (2) có nghiệm t 1 = - 1 ; t 2 = - c / a = 7 .
Đối chiếu điều kiện chỉ có nghiệm t = 7 thỏa mãn.
+ Với t = 7 ⇒ √x = 7 ⇔ x = 49 (thỏa mãn).
Vậy phương trình đã cho có nghiệm x = 49.
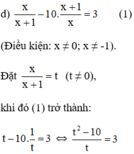
⇔ t 2 – 10 = 3 t ⇔ t 2 – 3 t – 10 = 0 ( 2 )
Giải (2): Có a = 1; b = -3; c = -10
⇒ Δ = ( - 3 ) 2 - 4 . 1 . ( - 10 ) = 49 > 0
⇒ (2) có hai nghiệm:
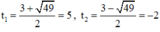
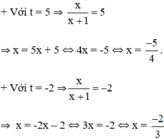
Cả hai nghiệm đều thỏa mãn điều kiện xác định.
Vậy phương trình đã cho có tập nghiệm 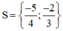

\(x^2+6x-3=4x\sqrt{2x-1}\left(1\right)\) ĐK: \(x\ge\frac{1}{2}\)
Đặt \(\sqrt{2x-1}=a\ge0\)
\(\Rightarrow6x-3=3a^2\)
=> (1) <=> x^2 +3a^2 = 4ax
<=> x^2 -4ax +3a^2 =0
<=> x^2 -ax - 3ax + 3a^2 =0
<=> x(x-a) -3a(x-a) =0
<=> (x-a) ( x-3a ) =0
\(\Leftrightarrow\orbr{\begin{cases}x=a\\x=3a\end{cases}}\)
TH1: x=a
\(\Rightarrow x=\sqrt{2x-1}\)\(\left(x\ge0\right)\)
\(\Leftrightarrow x^2=2x-1\)
\(\Leftrightarrow\left(x-1\right)^2=0\)
<=> x=1 (tm)
TH2: x= 3a
\(\Rightarrow x=3\sqrt{2x-1}\left(x\ge0\right)\)
\(\Leftrightarrow x^2=18x-9\)
\(\Leftrightarrow x^2-18x+9=0\)
\(\Delta=288\)
=> pt có 2 nghiệm pb \(\orbr{\begin{cases}x=\frac{18+12\sqrt{2}}{2}=9+6\sqrt{2}\left(tm\right)\\x=\frac{18-12\sqrt{2}}{2}=9-6\sqrt{2}\left(tm\right)\end{cases}}\)
Vậy ...

ĐK \(x\ge0\)
Đặt \(x=a,x+1=b\)
\(PT\Leftrightarrow a^4+b^4=\left(a+b\right)^4\)
<=> 4a3b+6a2b2+4ab3=0
<=> ab(2a2+3ab+2b2)=0
=>ab=0 (vì 2a2+3ab+2b2>0)
=>\(\orbr{\begin{cases}a=0\\b=0\end{cases}}\)<=>\(\orbr{\begin{cases}x=0\\x=-1\end{cases}}\)
Vậy.............................

ĐKXĐ: \(x\ge\dfrac{3}{4}\)
\(\Leftrightarrow\sqrt{5x^2+5x}=\sqrt{8x^2+10x-12}\) (1)
\(\Leftrightarrow\left(\sqrt{5x^2+5x}\right)^2=\left(\sqrt{8x^2+10x-12}\right)^2\)
\(\Leftrightarrow5x^2+5x=8x^2+10x-12\)
\(\Leftrightarrow5x^2+5x-\left(8x^2+10x-12\right)=8x^2+10x-12-\left(8x^2+10x-12\right)\)
\(\Leftrightarrow-3x^2-5x+12=0\)
\(\Leftrightarrow\left(-3x+4\right)\left(x+3\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}-3x+4=0\\x+3=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}-3x=-4\\x=-3\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{4}{3}\left(OK\right)\\x=-3\left(loại\right)\end{matrix}\right.\)
Vậy tập nghiệm phương trình (1) là \(S=\left\{\dfrac{4}{3}\right\}\)
ĐKXĐ: \(x\ge-1\)
\(x^2-1+\sqrt{x+1}=0\Rightarrow\left(x-1\right)\left(x+1\right)+\sqrt{x+1}=0\)
\(\Rightarrow\left(x+1-2\right)\left(x+1\right)+\sqrt{x+1}=0\)
Đặt \(\sqrt{x+1}=t\ge0\Rightarrow x+1=t^2\) ta được:
\(\left(t^2-2\right)t^2+t=0\Rightarrow t\left(\left(t^2-2\right)t+1\right)=0\)
\(\Rightarrow t\left(t^3-2t+1\right)=0\Rightarrow t\left(t-1\right)\left(t^2+t-1\right)=0\)
\(\Rightarrow\left[{}\begin{matrix}t=0\\t-1=0\\t^2+t-1=0\end{matrix}\right.\) \(\Rightarrow\left[{}\begin{matrix}t=0\\t=1\\t=\dfrac{-1+\sqrt{5}}{2}\\t=\dfrac{-1-\sqrt{5}}{2}< 0\left(l\right)\end{matrix}\right.\)
TH1: \(t=0\Rightarrow\sqrt{x+1}=0\Rightarrow x=-1\)
TH2: \(t=1\Rightarrow\sqrt{x+1}=1\Rightarrow x+1=1\Rightarrow x=0\)
TH3: \(t=\dfrac{-1+\sqrt{5}}{2}\Rightarrow\sqrt{x+1}=\dfrac{-1+\sqrt{5}}{2}\Rightarrow x+1=\dfrac{3-\sqrt{5}}{2}\)
\(\Rightarrow x=\dfrac{3-\sqrt{5}}{2}-1=\dfrac{1-\sqrt{5}}{2}\)
Vậy pt có 3 nghiệm \(\left[{}\begin{matrix}x=-1\\x=0\\x=\dfrac{1-\sqrt{5}}{2}\end{matrix}\right.\)
Lời giải:
Đặt \(\sqrt{x+1}=a\Rightarrow 1=a^2-x\)
PT trở thành: \(x^2+a=a^2-x\)
\(\Leftrightarrow x^2-a^2+(a+x)=0\)
\(\Leftrightarrow (x+a)(x-a+1)=0\Rightarrow \left[\begin{matrix} x=-a\\ x+1=a\end{matrix}\right.\)
Nếu \(x=-a=-\sqrt{x+1}\Rightarrow \left\{\begin{matrix} x\leq 0\\ x^2=x+1\end{matrix}\right.\Rightarrow x=\frac{1+\sqrt{5}}{2}\)
Nếu \(x+1=a=\sqrt{x+1}\Rightarrow (x+1)^2=(x+1)\Rightarrow x(x+1)=0\)
\(\Rightarrow \left[\begin{matrix} x=0\\ x=-1\end{matrix}\right.\) (đều thỏa mãn)
Vậy.........