BÀI 1: Giải phương trình sau:
a. (x - 4)3 = (x + 4)(x2 - x - 16)
b. \(\dfrac{x+2}{x}=\dfrac{x^2+5x+4}{x^2+2x}+\dfrac{x}{x+2}\)
c. \(\dfrac{x+1}{x-2}-\dfrac{5}{x+2}=\dfrac{12}{x^2-4}+1\)
d. (x + 3)2 - 25 = 0
e. \(\dfrac{3}{2x+10}+\dfrac{2x}{25-x^2}+\dfrac{3}{x-5}=0\)
f. \(\dfrac{x+5}{x-1}-\dfrac{x-1}{x-3}=\dfrac{8}{x^2-4x-3}\)
BÀI 2: Tìm m để phương trình sau số nghiệm x = 1:
3.(2x + m)(x + 2) - 2.(2x+1) = 18
BÀI 3: Giải phương trình:
a. (x-2)2 - 4.(x + 3) = x.(x-4)
b....
Đọc tiếp
BÀI 1: Giải phương trình sau:
a. (x - 4)3 = (x + 4)(x2 - x - 16)
b. \(\dfrac{x+2}{x}=\dfrac{x^2+5x+4}{x^2+2x}+\dfrac{x}{x+2}\)
c. \(\dfrac{x+1}{x-2}-\dfrac{5}{x+2}=\dfrac{12}{x^2-4}+1\)
d. (x + 3)2 - 25 = 0
e. \(\dfrac{3}{2x+10}+\dfrac{2x}{25-x^2}+\dfrac{3}{x-5}=0\)
f. \(\dfrac{x+5}{x-1}-\dfrac{x-1}{x-3}=\dfrac{8}{x^2-4x-3}\)
BÀI 2: Tìm m để phương trình sau số nghiệm x = 1:
3.(2x + m)(x + 2) - 2.(2x+1) = 18
BÀI 3: Giải phương trình:
a. (x-2)2 - 4.(x + 3) = x.(x-4)
b. \(\dfrac{3}{x+1}+\dfrac{x-1}{x-2}=\dfrac{x}{x-2}\)
c. \(\dfrac{x}{2x-6}+\dfrac{x}{2x+2}=\dfrac{2x^2}{x^2-2x-3}\)
d. (x + 3)2 - (x - 3)2 = 6x + 18
e. \(\dfrac{x+3}{x-2}=\dfrac{5}{\left(x-2\right)\left(3-x\right)}\)
f. \(\dfrac{12x^2+30x-21}{16x^2-9}-\dfrac{3x-7}{3-4x}=\dfrac{6x+5}{4x+3}\)
g. \(\dfrac{4}{x+1}-\dfrac{2}{x-2}=\dfrac{x+3}{x^2-x-2}\)
GIÚP MÌNH VỚI NHA !!!!

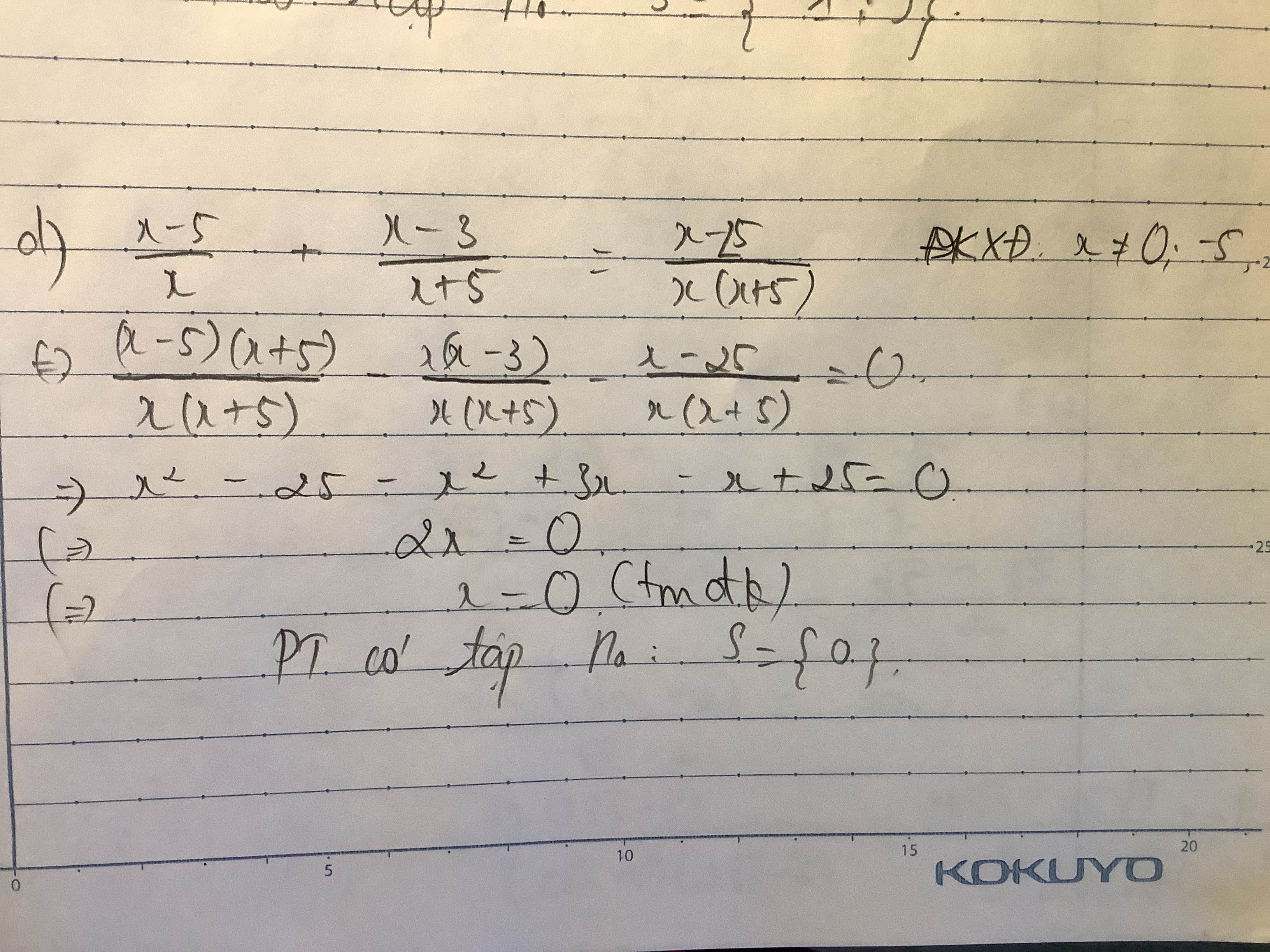

1.a/ \(\left\{{}\begin{matrix}3^{x+1}>0\\5^{x^2}>0\end{matrix}\right.\) \(\forall x\) \(\Rightarrow\) pt vô nghiệm
b/ Mình làm câu b, câu c bạn tự làm tương tự, 3 câu này cùng dạng
Lấy ln hai vế:
\(ln\left(3^{x^2-2}.4^{\dfrac{2x-3}{x}}\right)=ln18\Leftrightarrow ln3^{x^2-2}+ln4^{\dfrac{2x-3}{x}}-ln18=0\)
\(\Leftrightarrow\left(x^2-2\right)ln3+\dfrac{2x-3}{x}2ln2-ln\left(2.3^2\right)=0\)
\(\Leftrightarrow x^3ln3-2x.ln3+4x.ln2-6ln2-x.ln2-2x.ln3=0\)
\(\Leftrightarrow x^3ln3-4x.ln3+3x.ln2-6ln2=0\)
\(\Leftrightarrow x.ln3\left(x^2-4\right)+3ln2\left(x-2\right)=0\)
\(\Leftrightarrow\left(x-2\right)\left(x^2ln3+2x.ln3+3ln2\right)=0\)
\(\Rightarrow\left[{}\begin{matrix}x-2=0\Rightarrow x=2\\x^2ln3+2x.ln3+3ln2=0\left(1\right)\end{matrix}\right.\)
Xét (1): \(\left(x^2+2x\right)ln3=-3ln2\Leftrightarrow x^2+2x=\dfrac{-3ln2}{ln3}=-3log_32\)
\(\Leftrightarrow\left(x+1\right)^2=1-3log_32=log_33-log_38=log_3\dfrac{3}{8}< 0\)
\(\Rightarrow\left(1\right)\) vô nghiệm
\(\Rightarrow\) pt có nghiệm duy nhất \(x=2\)
2/ Pt đã cho tương đương:
\(2017^{sin^2x}-2017^{cos^2x}=cos^2x-sin^2x\)
\(\Leftrightarrow2017^{sin^2x}+sin^2x=2017^{cos^2x}+cos^2x\)
Xét hàm \(f\left(t\right)=2017^t+t\) (\(0\le t\le1\))
\(\Rightarrow f'\left(t\right)=2017^t.ln2017+1>0\) \(\forall t\) \(\Rightarrow f\left(t\right)\) đồng biến
\(\Rightarrow f\left(t_1\right)=f\left(t_2\right)\Leftrightarrow t_1=t_2\)
\(\Rightarrow sin^2x=cos^2x\Rightarrow cos^2x-sin^2x=0\Rightarrow cos2x=0\)
\(\Rightarrow x=\dfrac{\pi}{4}+\dfrac{k\pi}{2}\)
Thế k=0; k=1 ta được 2 nghiệm thuộc đoạn đã cho là \(x=\dfrac{\pi}{4};x=\dfrac{3\pi}{4}\)
\(\Rightarrow\) tổng nghiệm là \(T=\dfrac{\pi}{4}+\dfrac{3\pi}{4}=\pi\)