Tại sao sin^2 π/3 = 1 tương đương Cos^2 π/3 = 0
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
12 tháng 5 2022
\(\cos a=\dfrac{-12}{13}\)
\(\sin b=\dfrac{4}{5}\)
\(\sin\left(a+b\right)=\sin a\cos b+\sin b\cos a\)
\(=\dfrac{5}{13}\cdot\dfrac{3}{5}+\dfrac{4}{5}\cdot\dfrac{-12}{13}=\dfrac{-45}{65}=\dfrac{-9}{13}\)
NS
1
NV
Nguyễn Việt Lâm
Giáo viên
21 tháng 4 2021
Chắc là \(0< a< \dfrac{\pi}{2}\)?
\(0< a< \dfrac{\pi}{2}\Rightarrow sina;cosa>0\)
\(\left\{{}\begin{matrix}sina=\sqrt{3}cosa\\sin^2a+cos^2a=1\end{matrix}\right.\) \(\Rightarrow\left(\sqrt{3}cosa\right)^2+cos^2a=1\)
\(\Rightarrow4cos^2a=1\Rightarrow cosa=\dfrac{1}{2}\)
\(\Rightarrow sina=\sqrt{3}cosa=\dfrac{\sqrt{3}}{2}\)
NV
Nguyễn Việt Lâm
Giáo viên
7 tháng 11 2021
\(sin^2x-2m.sinx.cosx-sinx.cosx+2mcos^2x=0\)
\(\Leftrightarrow sinx\left(sinx-cosx\right)-2mcosx\left(sinx-cosx\right)=0\)
\(\Leftrightarrow\left(sinx-cosx\right)\left(sinx-2m.cosx\right)=0\)
\(\Rightarrow\left[{}\begin{matrix}sinx=cosx\\sinx=2m.cosx\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}tanx=1\\tanx=2m\end{matrix}\right.\)
Do \(tanx=1\) ko có nghiệm đã cho nên \(tanx=2m\) phải có nghiệm trên khoảng đã cho
\(\Rightarrow tan\left(\dfrac{\pi}{4}\right)< 2m< tan\left(\dfrac{\pi}{3}\right)\)
\(\Rightarrow1< 2m< \sqrt[]{3}\)
\(\Rightarrow m\in\left(\dfrac{1}{2};\dfrac{\sqrt{3}}{2}\right)\) (hoặc có thể 1 đáp án là tập con của tập này cũng được)
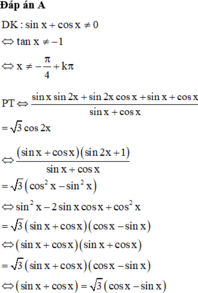
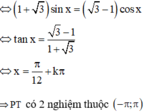
ta có : \(sin^2\dfrac{\pi}{3}+cos^2\dfrac{\pi}{3}=1\Rightarrow cos^2\dfrac{\pi}{3}=1-sin^2\dfrac{\pi}{3}=1-1=0\)