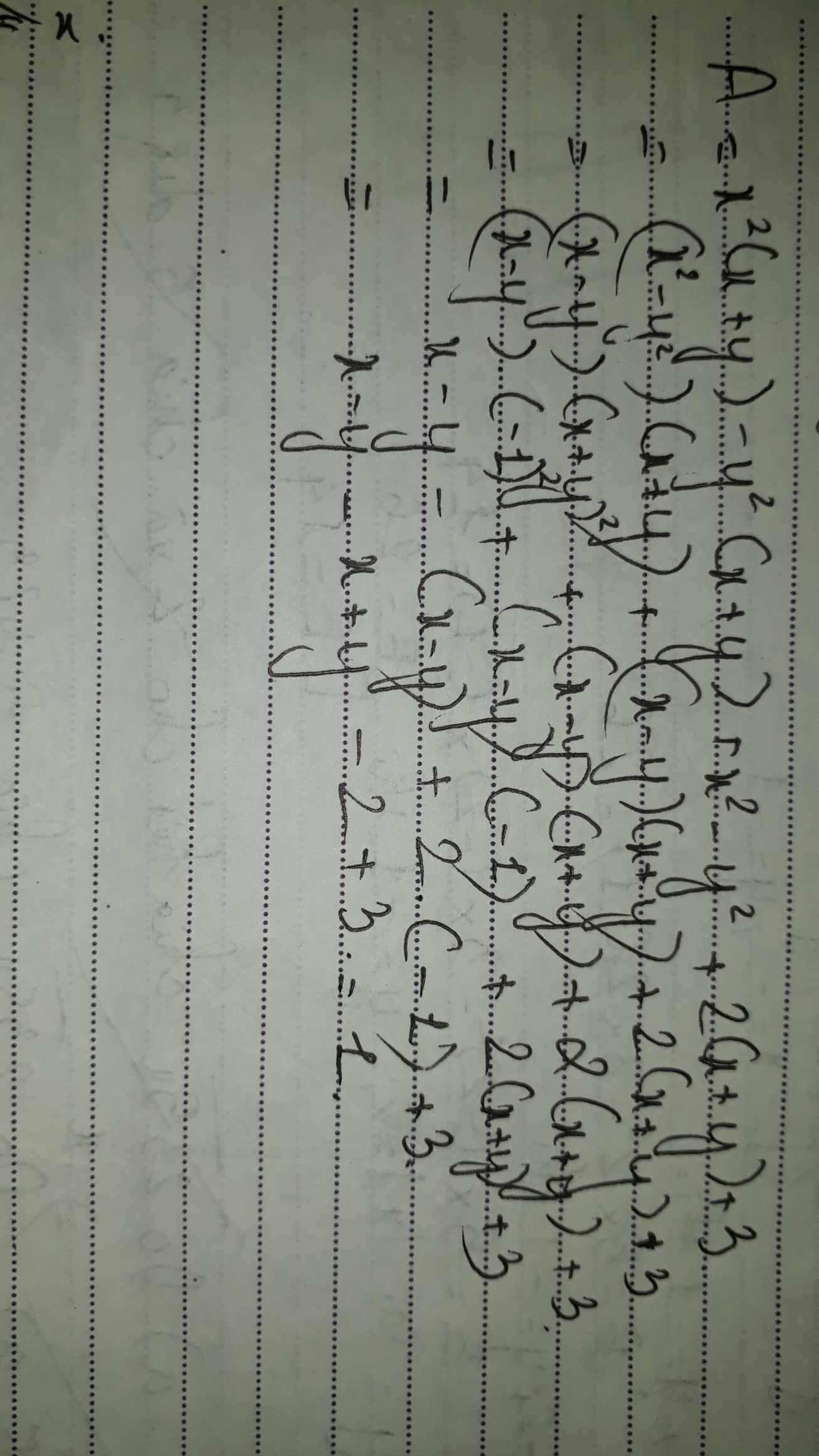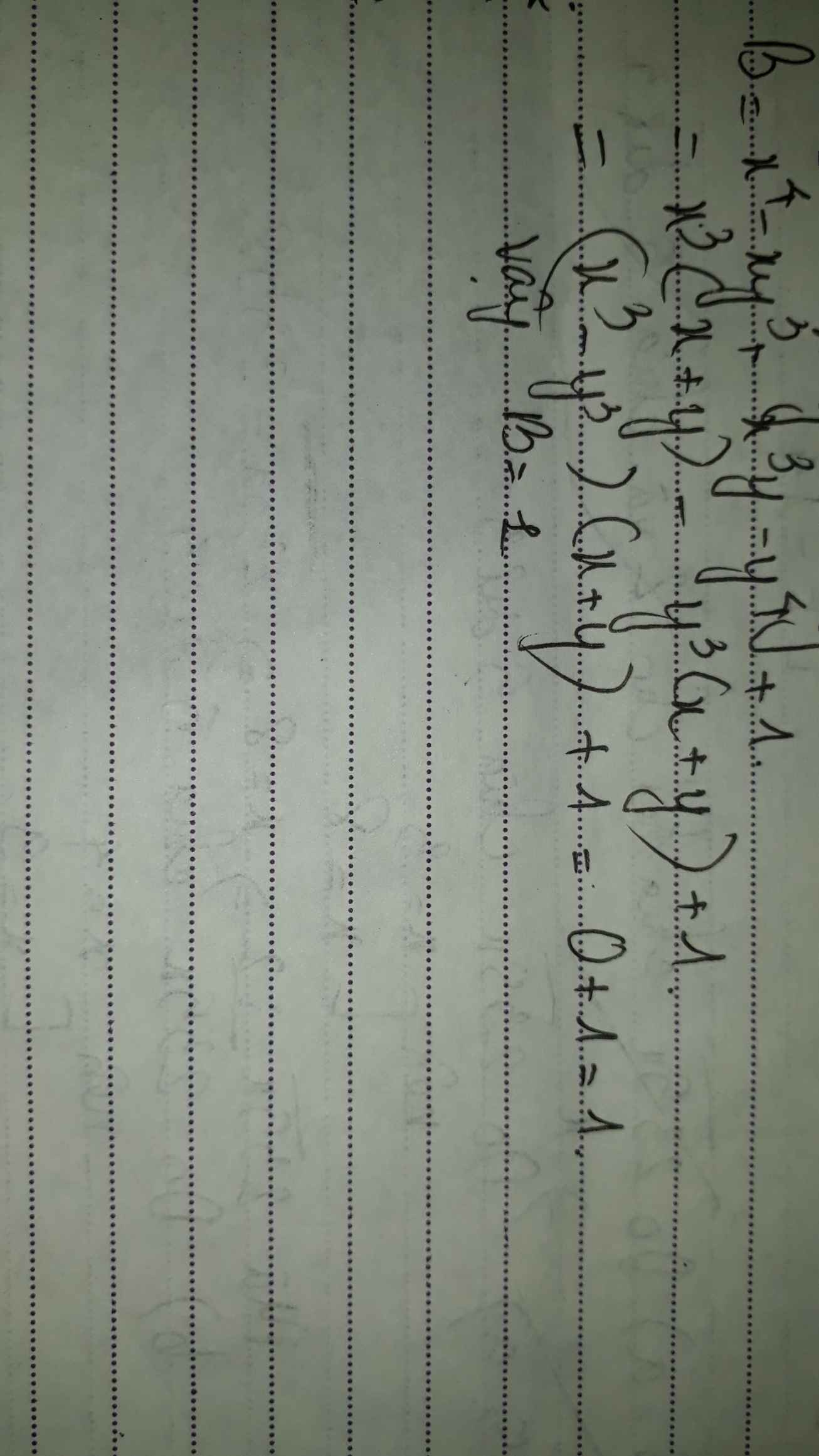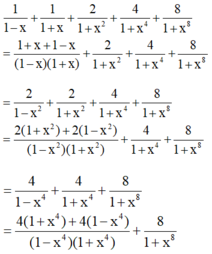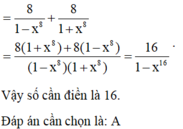cho x=y+1. CMR:(x+y)(x2+y2)(x4+y4)(x8+y8)=x16+y16
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
28 tháng 8 2021
a: \(A=x^2+y^2=\left(x+y\right)^2-2xy=15^2-2\cdot50=115\)
c: \(x-y=\sqrt{\left(x+y\right)^2-4xy}=\sqrt{15^2-4\cdot50}=5\)
\(C=x^2-y^2=\left(x+y\right)\left(x-y\right)=15\cdot5=75\)
VT
1
19 tháng 10 2021
\(g,=\left(x^2-y^2\right)\left(x^2+y^2\right)\left(x^4+y^4\right)=\left(x^4-y^4\right)\left(x^4+y^4\right)=x^8-y^8\)
\(b,=\left(x^2-9\right)\left(x-4\right)-\left(x^3+3x^2+3x+1\right)\\ =x^3-4x^2-9x+36-x^3-3x^2-3x-1\\ =-7x^2-12x+36\)
HT
1
PT
1
CM
11 tháng 2 2018
Ta có: VT = ( x 3 + x 2 y + x y 2 + y 3 )(x - y)
= ( x- y). ( x 3 + x 2 y + x y 2 + y 3 ).
= x. ( x 3 + x 2 y + x y 2 + y 3 ) - y( x 3 + x 2 y + x y 2 + y 3 )
= x 4 + x 3 y + x 2 y 2 + x y 3 – x 3 y – x 2 y 2 – x y 3 – y 4
= x 4 – y 4 = VP (đpcm)
Vế trái bằng vế phải nên đẳng thức được chứng minh.
25 tháng 8 2021
a) \(A=y\left(x^2-y^2\right)\left(x^2+y^2\right)-y\left(x^4-y^4\right)=y\left(x^4-y^4\right)-y\left(x^4-y^4\right)=0\)
b) \(B=\left(x-1\right)^3-\left(x-1\right)\left(x^2+x+1\right)-3\left(1-x\right)x=x^3-3x^2+3x-1-x^3-x^2-x+x^2+x+1-3x+3x^2=0\)
25 tháng 8 2021
a: Ta có: \(A=y\left(x^2-y^2\right)\left(x^2+y^2\right)-y\left(x^4-y^4\right)\)
\(=y\left(x^4-y^4\right)-y\left(x^4-y^4\right)\)
=0
b: Ta có: \(B=\left(x-1\right)^3-\left(x-1\right)\left(x^2+x+1\right)-3x\left(1-x\right)\)
\(=x^3-3x^2+3x-1-x^3+1-3x+3x^2\)
=0
29 tháng 8 2021
a: \(A=x^2+y^2=\left(x+y\right)^2-2xy=15^2-2\cdot50=125\)
b:\(B=x^4+y^4\)
\(=\left(x^2+y^2\right)^2-2x^2y^2\)
\(=125^2-2\cdot2500\)
=10625
c: \(x-y=\sqrt{\left(x+y\right)^2-4xy}=\sqrt{15^2-4\cdot50}=5\)
\(C=x^2-y^2=\left(x-y\right)\left(x+y\right)=15\cdot5=75\)
