chứng minh rằng: x-x2-1<0 với mọi số thực thuộc x
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
17 tháng 7 2021
a) Ta có: \(\dfrac{x^2+2x+1}{x^2+x}\)
\(=\dfrac{\left(x+1\right)^2}{x\left(x+1\right)}\)
\(=\dfrac{x+1}{x}\)
b) Ta có: \(\dfrac{x^2-4x+3}{x^2-x}\)
\(=\dfrac{\left(x-1\right)\left(x-3\right)}{x\left(x-1\right)}\)
\(=\dfrac{x-3}{x}\)
17 tháng 1 2021
x2 > 2( x - 1 )
<=> x2 - 2x + 2 > 0
<=> ( x2 - 2x + 1 ) + 1 > 0
<=> ( x - 1 )2 + 1 > 0 ( luôn đúng ∀ x ∈ R )
Vậy bđt ban đầu được chứng minh
PT
1
CM
7 tháng 10 2018
x y + ( 1 + x 2 ) ( 1 + y 2 ) = 1 ⇔ ( 1 + x ) 2 ( 1 + y ) 2 = 1 − x y ⇒ ( 1 + x 2 ) ( 1 + y 2 ) = 1 - x y 2 ⇔ 1 + x 2 + y 2 + x 2 y 2 = 1 − 2 x y + x 2 y 2 ⇔ x 2 + y 2 + 2 x y = 0 ⇔ x + y 2 = 0 ⇔ y = − x ⇒ x 1 + y 2 + y 1 + x 2 = x 1 + x 2 − x 1 + x 2 = 0
PT
Chứng minh rằng không có số hữu tỉ nào thoả mãn: a) x2 = 7 b) x2 – 3x = 1 c) x + với x khác 1 và -1.
0
PT
1
CM
31 tháng 7 2018
Thực hiện khai triển hằng đẳng thức
A = ( x 3 – 1) + ( x 3 – 6 x 2 + 12x – 8) – 2( x 3 + 1) + 6( x 2 – 2x + 1).
Rút gọn A = -5 không phụ thuộc biến x.
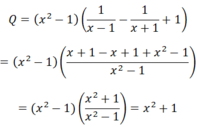
\(x-x^2-1=-x^2+x-1=-\left(x^2-2\cdot x\cdot\dfrac{1}{2}+\dfrac{1}{4}\right)-\dfrac{3}{4}=-\left(x-\dfrac{1}{2}\right)^2-\dfrac{3}{4}\)
Ta có: \(\left(x-\dfrac{1}{2}\right)^2\ge0\forall x\in R\)
\(\Rightarrow-\left(x-\dfrac{1}{2}\right)^2\le0\forall x\in R\)
\(\Rightarrow-\left(x-\dfrac{1}{2}\right)-\dfrac{3}{4}\le-\dfrac{3}{4}< 0\forall x\in R\)
\(\Rightarrow x-x^2-1< 0\forall x\in R\left(đpcm\right)\)
$x-x^2-1$
$=-(x^2-x+1)$
\(=-\left[\left(x-\dfrac{1}{2}\right)^2+\dfrac{3}{4}\right]\)
\(=-\left(x-\dfrac{1}{2}\right)^2-\dfrac{3}{4}\le-\dfrac{3}{4}< 0\)
Vậy \(x-x^2-1<0\)\(\forall x\in R\) \(\left(ĐPCM\right)\)