Phân tích đa thức sau thành nhân tử bằng nhiều cách
x2-8x+12
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
NC
2
BD
28 tháng 9 2017
C1
Ta có \(x^2-8x+12\)
\(=x^2-6x-2x+12\)
\(=x\left(x-6\right)-2\left(x-6\right)\)
\(=\left(x-6\right)\left(x-2\right)\)
BD
28 tháng 9 2017
C2
ta có \(x^2-8x+12\)
\(=x^2-8x+16-4\)
\(=\left(x-4\right)^2-2^2\)
\(=\left(x-4-2\right)\left(x-4+2\right)\)
\(=\left(x-6\right)\left(x-2\right)\)
18 tháng 6 2016
\(12x^2+7x-12=12x^2-5x+12x-12\)
\(=x\left(12x-5\right)+12\left(x-1\right)\)
Đề sai rồi bạn ời
16 tháng 8 2021
a: \(x^2-6x+5=\left(x-5\right)\left(x-1\right)\)
b: \(x^2-x-12=\left(x-4\right)\left(x+3\right)\)
c: \(x^2+8x+15=\left(x+5\right)\left(x+3\right)\)
d: \(2x^2-5x-12=\left(x-4\right)\left(2x+3\right)\)
e: \(x^2-13x+36=\left(x-9\right)\left(x-4\right)\)
CM
29 tháng 11 2018
3x2 + 8x + 2 = 0
Có a = 3; b' = 4; c = 2
⇒ Δ’ = 42 – 2.3 = 10 > 0
⇒ Phương trình có hai nghiệm phân biệt:
CP
2
10 tháng 8 2017
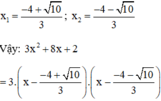
\(2x^3-5x^2+8x-3\)
\(\Leftrightarrow2x^3-x^2-4x^2+2x+6x+3\)
\(\Leftrightarrow x^2\cdot\left(2x-1\right)-2x\cdot\left(2x-1\right)+3\cdot\left(2x+1\right)\)
\(\Leftrightarrow\left(2x-1\right)\cdot\left(x^2-2x+3\right)\)
1 tháng 2 2018
\(2x^3-5x^2+8x-3\)
\(=2x^3-x^2-4x^2+2x+6x-3\)
\(=x^2\left(2x-1\right)-2x\left(2x-1\right)+3\left(2x-1\right)\)
\(=\left(2x-1\right)\left(x^2-2x+3\right)\)
CP
0
CP
1
1 tháng 8 2015
= 2x^3 - 4x^2 - x^2 + 2x + 6x - 3
= 2x^2 ( x - 1/2 ) - x ( x - 1/2 ) +3 ( x - 1/2 )
= ( x - 1/2 )( 2x^2 - x + 3 )
x2-8x + 16 - 4 = ( x - 4 )2-22= ( x -4-2 ) . ( x-4+2 ) = ( x - 6 ) .( x -2 )