cho đa thức A= x^5 - 2345x^4 + 2345x^3 + 2345X^2 + 2345x -2345
tính A biết x = 2344
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
NL
Nguyễn Lê Phước Thịnh
13 tháng 5 2022
Đúng(0)
Những câu hỏi liên quan
VC
0
20 tháng 2 2019
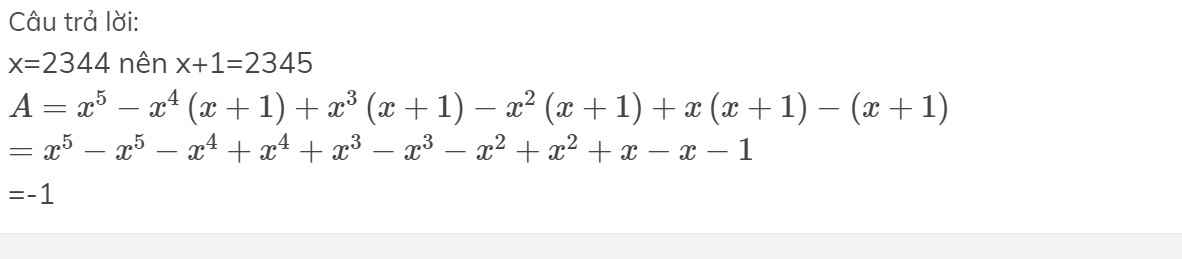
\(R=x^4\left(x-2345\right)+2345x^2\left(x-1\right)+2345\left(x-1\right)\)\(=-x^4+x^2\left(x+1\right)\left(x-1\right)+\left(x-1\right)\left(x+1\right)\)\(=-x^4+x^4-x^2+x^2-1=-1\)
Coi lại giúp mình nha
20 tháng 2 2019
\(gt\Rightarrow x+1=2345\)
\(R=x^5-\left(x+1\right)x^4+\left(x+1\right)x^3-\left(x+1\right)x^2+\left(x+1\right)x-\left(x+1\right)\)
\(R=x^5-x^5-x^4+x^4+x^3-x^3-x^2+x^2+x-x-1\)
\(R=-1\)
AH
Akai Haruma
Giáo viên
20 tháng 2 2019
Lời giải:
Ta biến đổi $R$ như sau :
\(R=x^5-2344x^4-x^4+2344x^3+x^3-2344x^2-x^2+2344x+x-2344-1\)
\(=(x^5-2344x^4)-(x^4-2344x^3)+(x^3-2344x^2)-(x^2-2344x)+(x-2344)-1\)
\(=x^4(x-2344)-x^3(x-2344)+x^2(x-2344)-x(x-2344)+(x-2344)-1\)
\(=(x-2344)(x^4-x^3+x^2-x+1)-1\)
Tại $x=2344$ thì \(x-2344=0\). Do đó:
\(R=0.(x^4-x^3+x^2-x+1)-1=-1\)
PH
0
AF
1
13 tháng 5 2022
x=2344 nên x+1=2345
\(A=x^5-x^4\left(x+1\right)+x^3\left(x+1\right)-x^2\left(x+1\right)+x\left(x+1\right)-\left(x+1\right)\)
\(=x^5-x^5-x^4+x^4+x^3-x^3-x^2+x^2+x-x-1\)
=-1
BH
0
T
2
28 tháng 1 2018
x + ( x + 1 ) + ( x + 2 ) + .... + ( x + 2344 ) + 2345 = 2345
( có 2346 số hạng )
x + ( x + 1 ) + ( x + 2 ) + ..... + ( x + 2344 ) = 2345 - 2345
x + ( x + 1 ) + ( x + 2 ) + ..... + ( x + 2344 ) = 0
( có 2345 số hạng )
[ ( x + 2344 ) + x ] . 2345 : 2 = 0
[ ( x + 2344 ) + x ] . 2345 = 0 . 2
( 2x + 2344 ) . 2345 = 0
2x + 2344 = 0 : 2345
2x + 2344 = 0
2x = 0 - 2344
2x = - 2344
x = - 2344 : 2 = - 1172
Vậy x = - 1172
