Tìm sso nguyên x lớn nhất sao cho giá trị biểu thức 0,2x + 0,5 lớn hơn giá trị biểu thức 3,4 - (2 - 0,7x)
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Để giá trị biểu thức 0,2x + 0,5 lớn hơn giá trị biểu thức 3,4 - ( 2 - 0,7x) thì :
\(0,2x+0,5>3,4-\left(2-0,7x\right)\\ \Leftrightarrow0,2x+0,5>3,4-2+0,7x\\ \Leftrightarrow-0,5x>0,9\\ \Leftrightarrow x< -\frac{9}{5}\)
Hay x < -1,8
=> x= -2
Vậy x=-2 là giá trị nguyên lớn nhất của x thỏa mãn yêu cầu.

1) \(A=1,7+\left|3,4-x\right|\ge1,7\)
\(minA=1,7\Leftrightarrow x=3,4\)
2) \(B=\left|x-2,8\right|-3,5\ge-3,5\)
\(minB=-3,5\Leftrightarrow x=2,8\)
3) \(C=0,5-\left|x-3,5\right|\le0,5\)
\(maxC=0,5\Leftrightarrow x=3,5\)

a: \(\dfrac{2x-2}{3}>=\dfrac{x+3}{6}\)
=>4x-4>=x+3
=>3x>=7
=>x>=7/3
b: (x+3)^2<(x-2)^2
=>6x+9<4x-4
=>2x<-13
=>x<-13/2
c: \(\dfrac{2x-3}{3}-x< =\dfrac{2x-3}{5}\)
=>2/3x-1-x<=2/5x-3/5
=>-11/15x<2/5
=>x>-6/11


Để giá trị của biểu thức x2 + 1 không lớn hơn giá trị của biểu thức (x - 2)2 thì:
x2 + 1 ≤ (x – 2)2
⇔ x2 + 1 ≤ x2 – 4x + 4
⇔ x2 – x2 + 4x ≤ 4 – 1 ( chuyển vế và đổi dấu hạng tử 1; x2 và – 4x).
⇔ 4x ≤ 3
⇔ 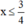 ( chia cả hai vế cho 4 > 0)
( chia cả hai vế cho 4 > 0)
Vậy 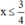

Answer:
a) \(\frac{5x}{2x+2}+1=\frac{6}{x+1}\)
\(\Rightarrow\frac{5x}{2\left(x+1\right)}+\frac{2\left(x+1\right)}{2\left(x+1\right)}=\frac{12}{2\left(x+1\right)}\)
\(\Rightarrow5x+2x+2-12=0\)
\(\Rightarrow7x-10=0\)
\(\Rightarrow x=\frac{10}{7}\)
b) \(\frac{x^2-6}{x}=x+\frac{3}{2}\left(ĐK:x\ne0\right)\)
\(\Rightarrow x^2-6=x^2+\frac{3}{2}x\)
\(\Rightarrow\frac{3}{2}x=-6\)
\(\Rightarrow x=-4\)
c) \(\frac{3x-2}{4}\ge\frac{3x+3}{6}\)
\(\Rightarrow\frac{3\left(3x-2\right)-2\left(3x+3\right)}{12}\ge0\)
\(\Rightarrow9x-6-6x-6\ge0\)
\(\Rightarrow3x-12\ge0\)
\(\Rightarrow x\ge4\)
d) \(\left(x+1\right)^2< \left(x-1\right)^2\)
\(\Rightarrow x^2+2x+1< x^2-2x+1\)
\(\Rightarrow4x< 0\)
\(\Rightarrow x< 0\)
e) \(\frac{2x-3}{35}+\frac{x\left(x-2\right)}{7}\le\frac{x^2}{7}-\frac{2x-3}{5}\)
\(\Rightarrow\frac{2x-3+5\left(x^2-2x\right)}{35}\le\frac{5x^2-7\left(2x-3\right)}{35}\)
\(\Rightarrow2x-3+5x^2-10x\le5x^2-14x+21\)
\(\Rightarrow6x\le24\)
\(\Rightarrow x\le4\)
f) \(\frac{3x-2}{4}\le\frac{3x+3}{6}\)
\(\Rightarrow\frac{3\left(3x-2\right)-2\left(3x+3\right)}{12}\le0\)
\(\Rightarrow9x-6-6x-6\le0\)
\(\Rightarrow3x\le12\)
\(\Rightarrow x\le4\)

Ta đặt t = \(\frac{1}{2004y}\)
Bài toán được đưa về tìm x để t bé nhất :
Ta có \(t=\frac{\left(x+2004\right)^2}{2004x}=\frac{x^2+2.2004x+2004^2}{2004x}=\frac{x}{2004}+2+\frac{2004}{x}=\frac{x^2+2004^2}{2004x}+2\) ( 1 )
Ta thấy : Theo bất đẳng thức Côsi cho 2 số dương ta có :
\(x^2+2004^2\ge2.2004.x\Rightarrow\frac{x^2+2004^2}{2004x}\ge2\) ( 2 )
Dấu " = " xảy ra khi x = 2004
Từ ( 1 ) và ( 2 ) \(\Rightarrow t\ge4\Rightarrow\) giá trị bé nhất của t = 4 khi x = 2004
Vậy \(y_{max}=\frac{1}{2004t}=\frac{1}{8016}\) . Khi \(x=2004\)
Chúc bạn học tốt !!!
TA CÓ :0,2x+0,5>3,4-2+0,7x
\(\Leftrightarrow-0,5x>0,9\)
\(\Leftrightarrow x< -1,8\)
Vậy x=-2
mình nghĩ là sai rùi
Ta có:\(0.2x+0.5>3.4-\left(2-0.7x\right)\)
\(\Leftrightarrow0.2x+0.5>3.4-2+0.7x\)
\(\Leftrightarrow-0.5x>3.4-2-0.5\)
\(\Leftrightarrow-0.5x>0.9\)
\(\Leftrightarrow x< -1.8\)
Mà x là số nguyên lớn nhất nên \(\text{x=}-2\)