Tính giá trị biểu thức A biết \(cosx=0,5;A=\dfrac{cosx+2sin^2x}{cos^2x-sinx}\)
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
DN
1
18 tháng 1 2022
Lần sau bạn vào cái hình E để gửi câu hỏi nha!
\(P=\dfrac{sin^2\alpha-sin\alpha\cdot cos\alpha+2cos^2\alpha}{2sin^2\alpha-cos^2\alpha}\)
\(P=\dfrac{tan^2\alpha-tan\alpha+2}{2tan^2\alpha-1}\) (Chia cả tử và mẫu cho \(cos^2\alpha\))
\(P=\dfrac{3^2-3+2}{2\cdot3^2-1}=\dfrac{8}{17}\)
Chúc bn học tốt!
HB
1
NV
Nguyễn Việt Lâm
Giáo viên
4 tháng 3 2021
\(tan^2x+cot^2x=2=2.tanx.cotx\)
\(\Leftrightarrow tan^2x+cot^2x-2tanx.cotx=0\)
\(\Leftrightarrow\left(tanx-cotx\right)^2=0\Leftrightarrow tanx=cotx=\dfrac{1}{tanx}\)
\(\Leftrightarrow tanx=\pm1\)
\(P=\dfrac{1}{cosx}-\dfrac{cosx}{1+sinx}=\dfrac{1+sinx-cos^2x}{cosx\left(1+sinx\right)}=\dfrac{sin^2x+sinx}{cosx\left(1+sinx\right)}\)
\(=\dfrac{sinx\left(1+sinx\right)}{cosx\left(1+sinx\right)}=tanx=\pm1\)
24 tháng 5 2023
a: A=(sinx+cosx)^2-1=m^2-1
b: B=căn (sinx+cosx)^2-4sinxcosx=căn m^2-4(m^2-1)=căn -3m^2+4
c: C=(sin^2x+cos^2x)^2-2(sinx*cosx)^2=1-2m^2
PT
1
CM
7 tháng 2 2018
Ta có : sin2 x + cos2 x = 1 ⇒ sin2 x = 1 – cos2 x.
⇒ P = 3.sin2 x + cos2 x
= 3.(1 – cos2x) + cos2 x
= 3 – 3.cos2x + cos2x
= 3 – 2.cos2x
= 3 – 2.(1/3)2
= 3 – 2/9
= 25/9.
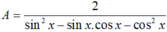
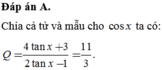
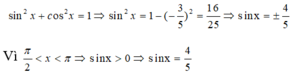
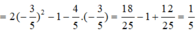
cos2x + sin2x=1
=>sin2x=1-cos2x=0.75
=>sinx=\(\pm\)\(\sqrt{3}\)/2
A= \(\dfrac{0,5+2.0,75}{0,5^2\pm\dfrac{\sqrt{3}}{2}}\)= \(\dfrac{-8\pm16\sqrt{3}}{11}\)