Cho hàm số FX có đạo hàm liên tục trên đoạn [0;1] bằng mãn f (1) = 1 và (f'(x))²+4(6x²-1).f(x)=40x⁶-44x⁴+32x²-4,Tích phân cân từ 0 đến 1 của xf(x)dx bằng
A.-13/15
B.5/12
C.13/15
B.-5/12
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
CM
23 tháng 9 2017
Đáp án A.
Mệnh đề 3 sai ví dụ hàm số y=|x| liên tục tại x = 0 nhưng không có đạo hàm tại điểm đó.
Mệnh đề 4 đúng vì nếu hàm số y=f(x) có đạo hàm trên [a;b] thì hàm số liên tục trên [a;b] do đó hàm số có nguyên hàm trên [a;b]
CM
24 tháng 7 2019
Đáp án D.
Ta có:
∫ 4 8 f ' x f x 2 d x = ∫ 4 8 f x − 2 d f x = f x − 1 − 1 4 8 = − 1 f 8 + 1 f 4 = − 2 + 4 = 2.
Gọi k là 1 hằng số thực. Xét
∫ 4 8 f ' x f 2 x + k 2 d x = ∫ 4 8 f ' x 2 f x 4 d x + 2 k ∫ 4 8 f ' x f 2 x d x + k 2 ∫ 4 8 d x = 1 + 2 k . k + 4 k 2 = 2 k + 1 2 .
Chọn k = − 1 2 , ta có ∫ 4 8 f ' x f 2 x − 1 2 2 d x = 0 , mà f ' x f 2 x − 1 2 2 ≥ 0 nên f ' x f 2 x − 1 2 2 = 0 ⇔ f ' x f 2 x = 1 2
⇒ ∫ f ' x f 2 x d x = x 2 + C ⇒ − 1 f x = x 2 + C .
Với x = 4 , ta có
− 1 f 4 = 2 + C ⇔ − 4 = 2 + C ⇔ C = − 6.
Do đó: f x = − 1 x 2 − 6 = 2 12 − x . Do đó f 6 = 2 12 − 6 = 2 6 = 1 3 .
CM
23 tháng 10 2019
Đáp án A.
Đặt u = f x d v = 3 x 2 d x ⇒ d u = f ' x d x v = x 3 ,
khi đó ∫ 0 1 3 x 2 f x d x = x 3 f x 1 0 − ∫ 0 1 x 3 f ' x d x .
⇒ 1 = f 1 − ∫ 0 1 x 3 f ' x d x ⇒ ∫ 0 1 x 3 f ' x d x = − 1 ⇔ ∫ 0 1 14 x 3 f ' x d x = − 7.
Mà ∫ 0 1 49 x 6 d x = 7
s u y r a ∫ 0 1 f ' x 2 d x + ∫ 0 1 7 ∫ 0 1 x 3 f ' x d x + ∫ 0 1 49 x 6 d x = 0 ⇔ ∫ 0 1 f ' x + 7 x 3 2 d x = 0.
Vậy
f ' x + 7 x 3 = 0 ⇒ 0 ⇒ f x = − 7 4 x 4 + C
CM
12 tháng 11 2017
Đặt
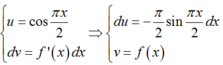
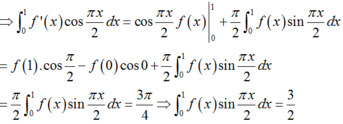
Xét tích phân
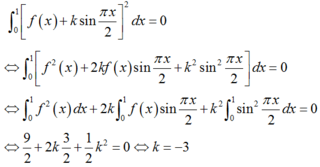
Khi đó ta có
∫ 0 1 f x = 3 sin π x 2 2 d x = 0 ⇔ f x - 3 sin π x 2 = 0 ⇔ f x = 3 sin π x 2
Vậy
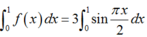
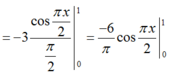
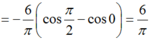
Chọn A.
CM
20 tháng 4 2018
Đáp án B.
Ta có ∫ 0 π 4 f ' x sin 2 x d x
Đặt u = sin 2 x d v = f ' x ⇒ d u = 2 cos 2 xdx v = f x suy ra
∫ 0 π 4 f ' x sin 2 x d x = sin 2 x f x π 4 0 − ∫ 0 π 4 2 cos 2 x . f x d x
⇒ − 2 ∫ 0 π 4 c o s 2 x . f x d x = − π 4 ⇒ ∫ 0 π 4 c o s 2 x . f x d x = π 8
Lại có: ∫ 0 π 4 c o s 2 2 x . f x d x = π 8 ⇒ ∫ 0 π 4 f x − c o s 2 x 2 d x = 0 ⇒ f x = c o s 2 x
Do đó ∫ 0 π 8 f 2 x d x = ∫ 0 π 8 c o s 4 x d x = sin 4 x 4 π 8 0 = 1 4 .
CM
23 tháng 12 2018
Đáp án B.
Ta có ∫ 0 1 f ' x . c o s π x d x
= ∫ 0 1 c o s π x d f x = f x . c o s π x 0 1 − ∫ 0 1 f x . c o s π x ' d x
= − f 1 + f 0 + π ∫ 0 1 f x . sin π x d x = π 2 ⇒ ∫ 0 1 f x . sin π x d x = 1 2 .
Xét ∫ 0 1 f x + k . sin π x 2 d x = 0
⇔ ∫ 0 1 f 2 x d x + 2 k . ∫ 0 1 f x . sin π x d x + k 2 . ∫ 0 1 sin 2 π x d x = 0
⇔ 1 2 k 2 + 2 k . 1 2 + 1 2 = 0 ⇔ k + 1 2 = 0 ⇔ k = − 1.
Suy ra ∫ 0 1 f x − sin π x 2 d x = 0.
Vậy f x = sin π x ⇒ ∫ 0 1 f x d x = ∫ 0 1 sin π x d x = 2 π .
CM
16 tháng 3 2019
Xét hàm f x = 3 x 2 + 6 x 2 + 6 x + 1 3 trên - 2 ; 1
Ta có
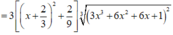
Nhận thấy f ' x > 0 , ∀ x ∈ ℝ
⇒ Hàm số đồng biến trên - 2 ; 1
Suy ra m a x [ - 2 , 1 ] f x = f 1 = 16 3
Chọn C
Lấy tích phân 2 vế:
\(\int\limits^1_0\left[f'\left(x\right)\right]^2dx+\int\limits^1_04\left(6x^2-1\right)f\left(x\right)dx=\int\limits^1_0\left(40x^6-44x^4+32x^2-4\right)dx=\frac{376}{105}\)
Xét \(I=\int\limits^1_0\left(6x^2-1\right)f\left(x\right)dx\)
Đặt \(\left\{{}\begin{matrix}u=f\left(x\right)\\dv=\left(6x^2-1\right)dx\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}du=f'\left(x\right)dx\\v=2x^3-x\end{matrix}\right.\)
\(\Rightarrow I=\left(2x^3-x\right)f\left(x\right)|^1_0-\int\limits^1_0\left(2x^3-x\right)f'\left(x\right)dx=1-\int\limits^1_0\left(2x^3-x\right)f'\left(x\right)dx\)
\(\Rightarrow\int\limits^1_0\left[f'\left(x\right)\right]^2dx+4-4\int\limits^1_0\left(2x^3-x\right)f'\left(x\right)dx=\frac{376}{105}\)
\(\Leftrightarrow\int\limits^1_0\left[f'\left(x\right)-2\left(2x^3-x\right)\right]^2dx-\int\limits^1_04\left(2x^3-x\right)^2dx=-\frac{44}{105}\)
\(\Leftrightarrow\int\limits^1_0\left[f'\left(x\right)-2\left(2x^3-x\right)\right]^2dx-\frac{44}{105}=-\frac{44}{105}\)
\(\Leftrightarrow\int\limits^1_0\left[f'\left(x\right)-\left(4x^3-2x\right)\right]^2dx=0\)
\(\Rightarrow f'\left(x\right)=4x^3-2x\Rightarrow f\left(x\right)=x^4-x^2+C\)
\(f\left(1\right)=1\Rightarrow1-1+C=1\Rightarrow C=1\)
\(\Rightarrow f\left(x\right)=x^4-x^2+1\)
\(\Rightarrow\int\limits^1_0x\left(x^4-x^2+1\right)dx=\frac{5}{12}\)