Giải giúp mình câu 17 này với ạ
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.



Nãy ghi nhầm =="
a)Hđ gđ là nghiệm pt
`x^2=2x+2m+1`
`<=>x^2-2x-2m-1=0`
Thay `m=1` vào pt ta có:
`x^2-2x-2-1=0`
`<=>x^2-2x-3=0`
`a-b+c=0`
`=>x_1=-1,x_2=3`
`=>y_1=1,y_2=9`
`=>(-1,1),(3,9)`
Vậy tọa độ gđ (d) và (P) là `(-1,1)` và `(3,9)`
b)
Hđ gđ là nghiệm pt
`x^2=2x+2m+1`
`<=>x^2-2x-2m-1=0`
PT có 2 nghiệm pb
`<=>Delta'>0`
`<=>1+2m+1>0`
`<=>2m> -2`
`<=>m> 01`
Áp dụng hệ thức vi-ét:`x_1+x_2=2,x_1.x_2=-2m-1`
Theo `(P):y=x^2=>y_1=x_1^2,y_2=x_2^2`
`=>x_1^2+x_2^2=14`
`<=>(x_1+x_2)^2-2x_1.x_2=14`
`<=>4-2(-2m-1)=14`
`<=>4+2(2m+1)=14`
`<=>2(2m+1)=10`
`<=>2m+1=5`
`<=>2m=4`
`<=>m=2(tm)`
Vậy `m=2` thì ....

11 He said that they were doing an experiment
12 She told me that I had to sign the paper again
13 Tam said they had watched a television documentary on the future of nuclear power
14 They announced that the 10 o'clock flight to Kuala Lumpur would be an hour late
15 Scientists said that in 50 years' time we might be living on the moon
16 My grandmother said that there had been no cell phones a long time before
17 Two days ago, Tom phoned me and said he couldn't come in that day, because he was sick

\(y'=\dfrac{\left(-2x+2\right)\left(x-3\right)-\left(-x^2+2x+c\right)}{\left(x-3\right)^2}=\dfrac{-x^2+6x-6-c}{\left(x-3\right)^2}\)
\(\Rightarrow\) Cực đại và cực tiểu của hàm là nghiệm của: \(-x^2+6x-6-c=0\) (1)
\(\Delta'=9-\left(6+c\right)>0\Rightarrow c< 3\)
Gọi \(x_1;x_2\) là 2 nghiệm của (1) \(\Rightarrow\left\{{}\begin{matrix}-x_1^2+6x_1-6=c\\-x_2^2+6x_2-6=c\end{matrix}\right.\)
\(\Rightarrow m-M=\dfrac{-x_1^2+2x_1+c}{x_1-3}-\dfrac{-x_2^2+2x_2+c}{x_2-3}=4\)
\(\Leftrightarrow\dfrac{-2x_1^2+8x_1-6}{x_1-3}-\dfrac{-2x_2^2+8x_2-6}{x_2-3}=4\)
\(\Leftrightarrow2\left(1-x_1\right)-2\left(1-x_2\right)=4\)
\(\Leftrightarrow x_2-x_1=2\)
Kết hợp với Viet: \(\left\{{}\begin{matrix}x_2-x_1=2\\x_1+x_2=6\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}x_1=2\\x_2=4\end{matrix}\right.\)
\(\Rightarrow c=2\)
Có 1 giá trị nguyên


a) \(d\left(A;\Delta\right)=\dfrac{\left|4.1-3.3+2\right|}{\sqrt{4^2+\left(-3\right)^2}}=\dfrac{3}{5}\)
b) \(\overrightarrow{AB}=\left(-3;-2\right)\) là VTCP của đường thẳng d
PT tham số của d: \(\left\{{}\begin{matrix}x=1-3t\\y=3-2t\end{matrix}\right.\left(t\in R\right)\)
c) Đường tròn (C) có bán kính \(R=AB=\sqrt{\left(1+2\right)^2+\left(3-1\right)^2}=\sqrt{13}\)
PT đường tròn (C): \(\left(x-1\right)^2+\left(y-3\right)^2=13\)


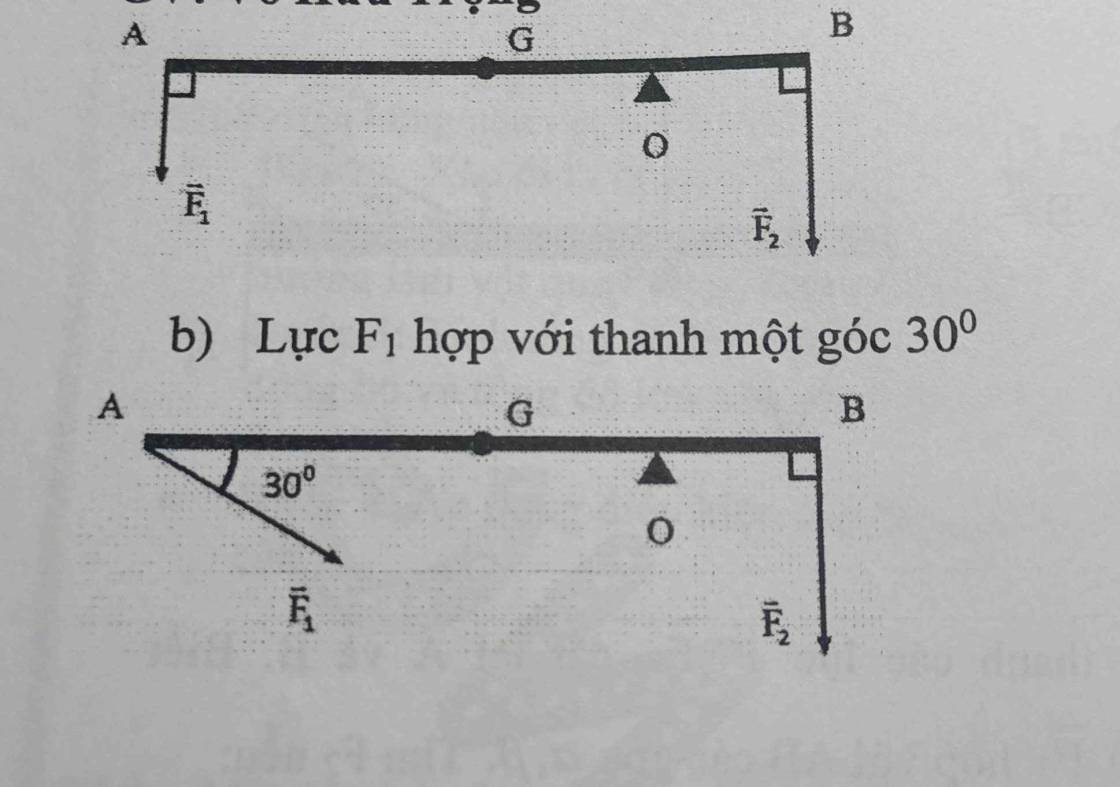
a) AD đk cân bằng momen ta có
`F_1 *OA + P*GO = OB*F_2`
`<=> F_1 *(AB-OB) + mg(AB-OB - AB/2) = OB *F_2`
`<=> F_1 (120 - 30) + 2*10*(120 - 30 -120/2) = 30*50`
`=> F_1 = 10N`
b) AD đk cân bằng momen ta có
`F_1 * sin30^o *OA + P*GO = OB*F_2`
`<=> F_1 *sin30^o *(AB-OB) + mg(AB-OB - AB/2) = OB *F_2`
`<=> F_1*sin30^o * (120 - 30) + 2*10*(120 - 30 -120/2) = 30*50`
`=> F_1 = 20(N)`








Giả sử: \(\left\{{}\begin{matrix}n_{Mg}=x\left(mol\right)\\n_{Fe}=y\left(mol\right)\end{matrix}\right.\)
⇒ 24x + 56y = 23,2 (1)
Ta có: \(n_{SO_2}=\dfrac{17,92}{22,4}=0,8\left(mol\right)\)
Theo ĐLBT mol e, có: 2x + 3y = 0,8.2 (2)
Từ (1) và (2) \(\Rightarrow\left\{{}\begin{matrix}x=0,5\left(mol\right)\\y=0,2\left(mol\right)\end{matrix}\right.\)
\(\Rightarrow\%m_{Mg}=\dfrac{0,5.24}{23,2}.100\%\approx51,7\%\)
Bạn tham khảo nhé!
Bài 17:
Đặt \(\left\{{}\begin{matrix}n_{Mg}=a\left(mol\right)\\n_{Fe}=b\left(mol\right)\end{matrix}\right.\) \(\Rightarrow24a+56b=23,2\) (1)
Ta có: \(n_{SO_2}=\dfrac{17,92}{22,4}=0,8\left(mol\right)\)
Bảo toàn electron: \(2a+3b=0,8\cdot2=1,6\) (2)
Từ (1) và (2) \(\Rightarrow\left\{{}\begin{matrix}a=0,5\\b=0,2\end{matrix}\right.\)
\(\Rightarrow\%m_{Mg}=\dfrac{0,5\cdot24}{23,2}\cdot100\%\approx51,72\%\)