SA
3
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
PT
1
CM
9 tháng 2 2017
Q = x 2 + 2 y 2 + 2 x y − 2 x − 6 y + 2015 = x 2 + 2 x y + y 2 − 2 x − 2 y + 1 + y 2 − 4 y + 4 + 2010 = x 2 + 2 x y + y 2 − 2 x + 2 y + 1 + y 2 − 4 y + 4 + 2010 = x + y 2 − 2 x + y + 1 + y 2 − 4 y + 4 + 2010 = x + y − 1 2 + y − 2 2 + 2010
HT
1
30 tháng 5 2022
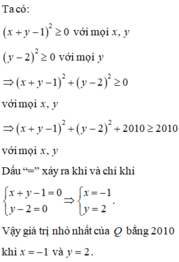
\(A=\left(x^2-2xy+y^2\right)+2\left(x-y\right)+1+y^2-8y+16-17\\ A=\left(x-y+1\right)^2+\left(y-4\right)^2-16\ge17\)
Vậy \(A_{min}=17\leftrightarrow\left\{{}\begin{matrix}x-y+1=0\\y-4=0\end{matrix}\right.\leftrightarrow\left\{{}\begin{matrix}x=3\\y=4\end{matrix}\right.\)
CM
29 tháng 7 2019
A = x 2 + 2 y 2 – 2 x y + 2 x – 10 y ⇔ A = x 2 + y 2 + 1 – 2 x y + 2 x – 2 y + y 2 – 8 y + 16 – 17 ⇔ A = ( x 2 + y 2 + 12 – 2 . x . y + 2 . x . 1 – 2 . y . 1 ) + ( y 2 – 2 . 4 . y + 4 2 ) – 17 ⇔ A = ( x – y + 1 ) 2 + ( y – 4 ) 2 – 17
Vì với mọi x; y nên A ≥ -17 với mọi x; y
=> A = -17
⇔ x − y + 1 = 0 y − 4 = 0 ⇔ x = y − 1 y = 4 ⇔ x = 3 y = 4
Vậy A đạt giá trị nhỏ nhất là A = -17 tại x = 3 y = 4
Đáp án cần chọn là: B
NT
1
S
29 tháng 12 2020
A= x2+2y2-2xy-2x-2y+1015
A = x2 - 2xy - 2x + y2 + 2y + 1 + y2 - 4y + 4 + 1010
A = [x2 - 2x(y + 1) + (y+1)2 ] + (y-2)2 + 1010
A = ( x - y - 1)2 + (y-2)2 + 1010 \(\ge1010\forall x,y\)
Dấu "=" xảy ra <=> \(\left\{{}\begin{matrix}x-y-1=0\\y-2=0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x=3\\y=2\end{matrix}\right.\)
Vậy MinA = 1010 <=> \(\left\{{}\begin{matrix}x=3\\y=2\end{matrix}\right.\)
CM
20 tháng 3 2019
A = x 2 + 2 y 2 – 2 x y + 2 x – 10 y ⇔ A = x 2 + y 2 + 1 – 2 x y + 2 x – 2 y + y 2 – 8 y + 16 – 17 ⇔ A = ( x 2 + y 2 + 1 2 – 2 . x . y + 2 . x . 1 – 2 . y . 1 ) + ( y 2 – 2 . 4 . y + 4 2 ) – 17 ⇔ A = ( x – y + 1 ) 2 + ( y – 4 ) 2 – 17
Vì x - y + 1 2 ≥ 0 y - 4 2 ≥ 0 với mọi x, y nên A ≥ -17 với mọi x, y
=> A = -17 ó x - y + 1 = 0 y - 4 = 0 ó x = y - 1 y = 4 ó x = 3 y = 4
Vậy A đạt giá trị nhỏ nhất là A = -17 tại x = 3 y = 4
Đáp án cần chọn là: C
N
1
13 tháng 1 2022
\(A=\left[\left(x^2-2xy+y^2\right)+2\left(x-y\right)+1\right]+\left(y^2-8y+16\right)-17\\ A=\left(x-y+1\right)^2+\left(y-4\right)^2-17\ge-17\)
Dấu \("="\Leftrightarrow\left\{{}\begin{matrix}x-y+1=0\\y-4=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=y-1=3\\y=4\end{matrix}\right.\)
A
Cho các số x khác 2y thỏa mãn x2- 2xy - 2y2 - 3x +6y=0
Tính giá trị biểu thức A= x2+ 2xy _y2 - 2x- 2y
0
AH
Akai Haruma
Giáo viên
27 tháng 5 2023
Lời giải:
$P=(x^2+y^2+2xy)+y^2-6x-8y+2028$
$=(x+y)^2-6(x+y)+(y^2-2y)+2028$
$=(x+y)^2-6(x+y)+9+(y^2-2y+1)+2018$
$=(x+y-3)^2+(y-1)^2+2018\geq 0+0+2018=2018$
Vậy $P_{\min}=2018$
Giá trị này đạt tại $x+y-3=y-1=0$
$\Leftrightarrow y=1; x=2$
21 tháng 9 2021
\(a,P=x^2-2x+5=\left(x^2-2x+1\right)+4=\left(x-1\right)^2+4\ge4\)
Dấu \("="\Leftrightarrow x=1\)
\(b,Q=2x^2-6x=2\left(x^2-2\cdot\dfrac{3}{2}x+\dfrac{9}{4}-\dfrac{9}{4}\right)=2\left(x-\dfrac{3}{2}\right)^2-\dfrac{9}{2}\ge-\dfrac{9}{2}\)
Dấu \("="\Leftrightarrow x=\dfrac{3}{2}\)
\(c,M=\left(x^2-x+\dfrac{1}{4}\right)+\left(y^2+6y+9\right)+\dfrac{3}{4}=\left(x-\dfrac{1}{2}\right)^2+\left(y+3\right)^2+\dfrac{3}{4}\ge\dfrac{3}{4}\)
Dấu \("="\Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{1}{2}\\y=-3\end{matrix}\right.\)
21 tháng 9 2021
a: Ta có: \(P=x^2-2x+5\)
\(=x^2-2x+1+4\)
\(=\left(x-1\right)^2+4\ge4\forall x\)
Dấu '=' xảy ra khi x=1
Bài làm:
a) \(P=x^2-5x=\left(x^2-5x+\frac{25}{4}\right)-\frac{25}{4}\)
\(=\left(x-\frac{5}{2}\right)^2-\frac{25}{4}\le-\frac{25}{4}\left(\forall x\right)\)
Dấu "=" xảy ra khi: \(x=\frac{5}{2}\)
Vậy \(Min_P=-\frac{25}{4}\Leftrightarrow x=\frac{5}{2}\)
a) P = x2 - 5x
= ( x2 - 5x + 25/4 ) - 25/4
= ( x - 5/2 )2 - 25/4
( x - 5/2 )2 ≥ 0 ∀ x => ( x - 5/2 )2 - 25/4 ≥ -25/4
Đẳng thức xảy ra <=> x - 5/2 = 0 => x = 5/2
=> MinF = -25/4 <=> x = 5/2
b) Q = x2 + 2y2 + 2xy - 2x - 6y + 2015
= ( x2 + 2xy + y2 - 2x - 2y + 1 ) + ( y2 - 4y + 4 ) + 2010
= [ ( x + y )2 - 2( x + y ) + 12 ] + ( y - 2 )2 + 2010
= ( x + y - 1 )2 + ( y - 2 )2 + 2010
\(\hept{\begin{cases}\left(x+y-1\right)^2\ge0\forall x,y\\\left(y-2\right)^2\ge0\forall x\end{cases}}\Rightarrow\left(x+y-1\right)^2+\left(y-2\right)^2+2010\ge2010\)
Đẳng thức xảy ra <=> \(\hept{\begin{cases}x+y-1=0\\y-2=0\end{cases}}\Rightarrow\hept{\begin{cases}x+y-1=0\\y=2\end{cases}}\Rightarrow\hept{\begin{cases}x=-1\\y=2\end{cases}}\)
=> MinQ = 2010 <=> x = -1 , y = 2