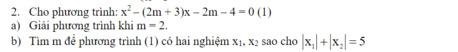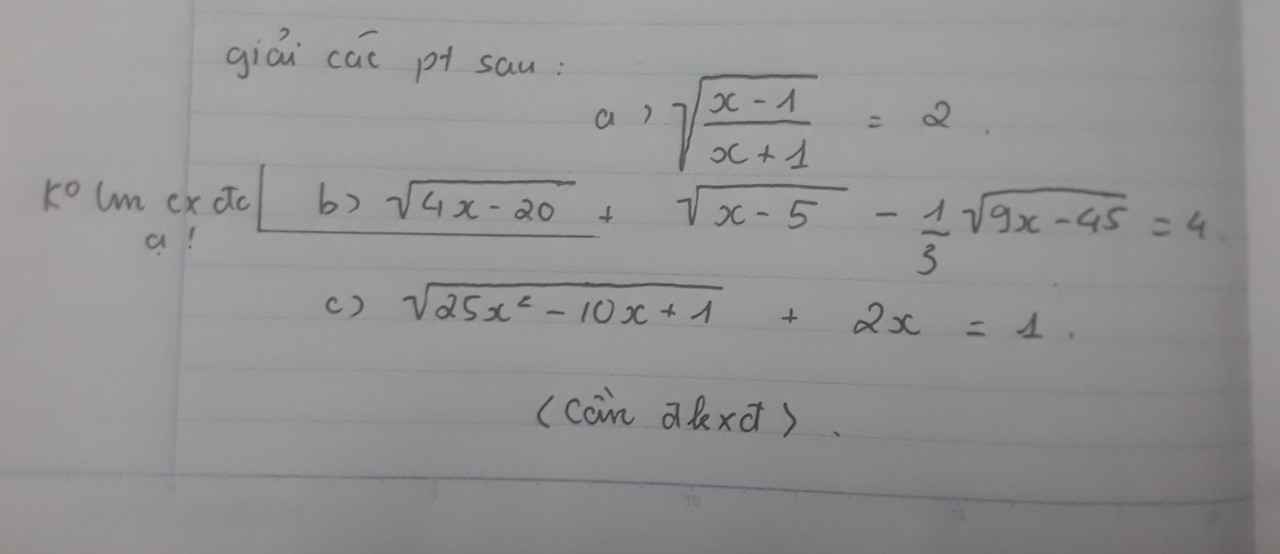T
0
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
NV
Nguyễn Việt Lâm
Giáo viên
5 tháng 3 2022
\(\Delta=\left(2m+3\right)^2+4\left(2m+4\right)=4m^2+20m+25=\left(2m+5\right)^2\ge0;\forall m\)
Pt đã cho luôn có 2 nghiệm với mọi m
Theo hệ thức Viet: \(\left\{{}\begin{matrix}x_1+x_2=2m-3\\x_1x_2=-2m-4\end{matrix}\right.\)
\(\left|x_1\right|+\left|x_2\right|=5\Leftrightarrow x_1^2+x_2^2+2\left|x_1x_2\right|=25\)
\(\Leftrightarrow\left(x_1+x_2\right)^2-2x_1x_2+2\left|x_1x_2\right|=25\)
\(\Leftrightarrow\left(2m-3\right)^2+2\left(2m+4\right)+2\left|2m+4\right|=25\)
\(\Leftrightarrow4m^2-8m-8+4\left|m+2\right|=0\)
TH1: \(m\ge-2\)
\(\Rightarrow4m^2-8m-8+4\left(m+2\right)=0\)
\(\Leftrightarrow4m^2-4m=0\Rightarrow\left[{}\begin{matrix}m=0\\m=1\end{matrix}\right.\) (thỏa mãn)
TH2: \(m\le-2\)
\(\Rightarrow4m^2-8m-8-4\left(m+2\right)=0\)
\(\Leftrightarrow4m^2-12m-16=0\Rightarrow\left[{}\begin{matrix}m=-1\left(loại\right)\\m=4\left(loại\right)\end{matrix}\right.\)
QH
2
18 tháng 9 2021
a)\(đkx\ge1,x\ne-1\)
\(\sqrt{\dfrac{x-1}{x+1}}=2\)
\(\Leftrightarrow\dfrac{x-1}{x+1}=4\)
\(\Leftrightarrow x-1=4x-4\)
\(\Leftrightarrow x=1\)(nhận)
Vậy S=\(\left\{1\right\}\)
c)đk\(25x^2-10x+1=\) \(\left(5x-1\right)^2\ge0\Leftrightarrow x\ge\dfrac{1}{5}\)
\(\sqrt{25x^2-10x+1}+2x=1\)
\(\Leftrightarrow\sqrt{\left(5x-1\right)^2}+2x=1\)
\(\Leftrightarrow5x-1+2x=1\)
\(\Leftrightarrow x=\dfrac{2}{7}\)(nhận)
Vậy S=\(\left\{\dfrac{2}{7}\right\}\)
18 tháng 9 2021
c: Ta có: \(\sqrt{25x^2-10x+1}+2x=1\)
\(\Leftrightarrow\left|5x-1\right|=1-2x\)
\(\Leftrightarrow\left[{}\begin{matrix}5x-1=1-2x\left(x\ge\dfrac{1}{5}\right)\\5x-1=2x-1\left(x< \dfrac{1}{5}\right)\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{2}{7}\left(nhận\right)\\x=0\left(nhận\right)\end{matrix}\right.\)
QN
0
