K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
2 tháng 7 2023
a: \(P=\dfrac{a+1+\sqrt{a}}{a+1}:\dfrac{a+1-2\sqrt{a}}{\left(\sqrt{a}-1\right)\left(a+1\right)}\)
\(=\dfrac{a+\sqrt{a}+1}{a+1}\cdot\dfrac{\left(a+1\right)\left(\sqrt{a}-1\right)}{\left(\sqrt{a}-1\right)^2}=\dfrac{a+\sqrt{a}+1}{\sqrt{a}-1}\)
b: P<1
=>P-1<0
=>\(\dfrac{a+\sqrt{a}+1-\sqrt{a}+1}{\sqrt{a}-1}< 0\)
=>căn a-1<0
=>0<a<1
c: Thay x=19-8căn3 vào P, ta được:
\(P=\dfrac{19-8\sqrt{3}+4+\sqrt{3}+1}{4+\sqrt{3}-1}=\dfrac{31-15\sqrt{3}}{2}\)
19 tháng 9 2021
\(1,\\ a,ĐK:\left\{{}\begin{matrix}x-2\ne0\\x-2\ge0\end{matrix}\right.\Leftrightarrow x>2\\ b,ĐK:\dfrac{1}{3-2x}\ge0\Leftrightarrow3-2x\ge0\left(1>0\right)\Leftrightarrow x\le\dfrac{3}{2}\)
\(2,\\ a,=\sqrt{\left(6-\sqrt{35}\right)\left(6+\sqrt{35}\right)}=\sqrt{36-35}=\sqrt{1}=1\\ b,=\sqrt{\left(9-\sqrt{17}\right)\left(9+\sqrt{17}\right)}=\sqrt{81-17}=\sqrt{64}=8\\ c,=4\sqrt{2}-6\sqrt{6}+9-4\sqrt{2}+6\sqrt{6}=9\\ d,=\dfrac{\sqrt{3}\left(\sqrt{3}+2\right)}{\sqrt{3}}+\dfrac{\sqrt{2}\left(\sqrt{2}+1\right)}{\sqrt{2}+1}-2-\sqrt{3}=\sqrt{3}+\sqrt{2}-2-\sqrt{3}=\sqrt{2}-2\\ e,=\left(200\sqrt{3}-225\sqrt{3}+25\sqrt{3}\right):\sqrt{15}=0:\sqrt{15}=0\)
lm hộ mk vs
AH
Akai Haruma
Giáo viên
3 tháng 8 2021
Lời giải:
a. Gọi biểu thức là $A$
\(A=\left[\frac{(1-\sqrt{x})(1+\sqrt{x}+x)}{1-\sqrt{x}}+\sqrt{x}\right].\left[\frac{1-\sqrt{x}}{(1-\sqrt{x})(1+\sqrt{x})}\right]^2\)
\(=(1+\sqrt{x}+x+\sqrt{x}).\frac{1}{(1+\sqrt{x})^2}=(\sqrt{x}+1)^2.\frac{1}{(\sqrt{x}+1)^2}=1\)
Ta có đpcm
b.
\(\sqrt{2012}-\sqrt{2011}=\frac{1}{\sqrt{2012}+\sqrt{2011}}< \frac{1}{\sqrt{2011}+\sqrt{2010}}=\sqrt{2011}-\sqrt{2010}\)
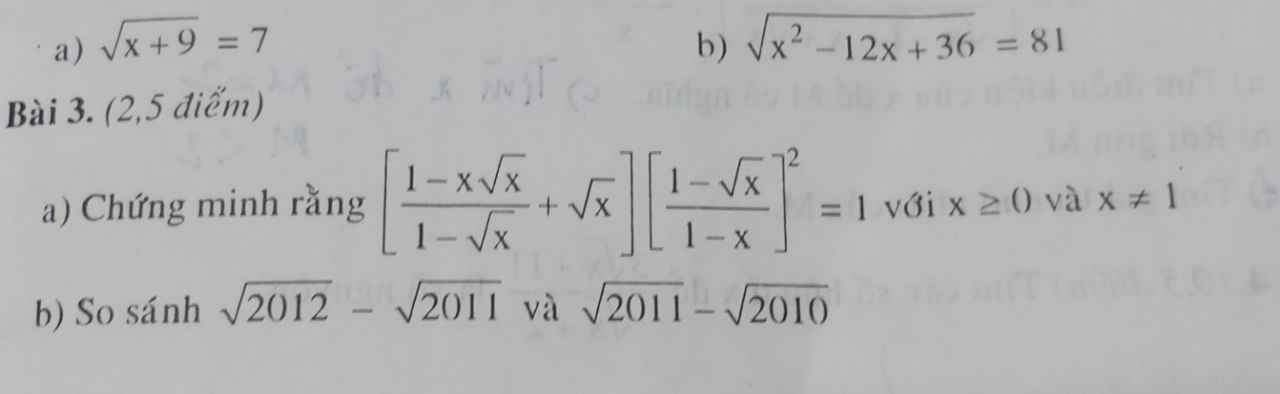
ĐKXĐ: \(m\ne-1,m\ne\dfrac{3}{2}\)
a) 2 đường thẳng song song
\(\Leftrightarrow\left\{{}\begin{matrix}m+1=3-2m\\n\ne-2\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}m=\dfrac{2}{3}\left(tm\right)\\n\ne-2\end{matrix}\right.\)
b) 2 đường thẳng cắt nhau tại một điểm trên trục tung:
\(\Leftrightarrow\left\{{}\begin{matrix}m\ne-1;m\ne\dfrac{3}{2}\\m+1\ne3-2m\\n=-2\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}m\ne-1;m\ne\dfrac{3}{2};m\ne\dfrac{2}{3}\\n=-2\end{matrix}\right.\)