Question

[Nguyễn Thị My Na]

giải phương trình :

\(x^4-3\text{x}^3+4\text{x}^2-3\text{x}+1=0\)

Answer 1

[Kiyotaka Ayanokoji]

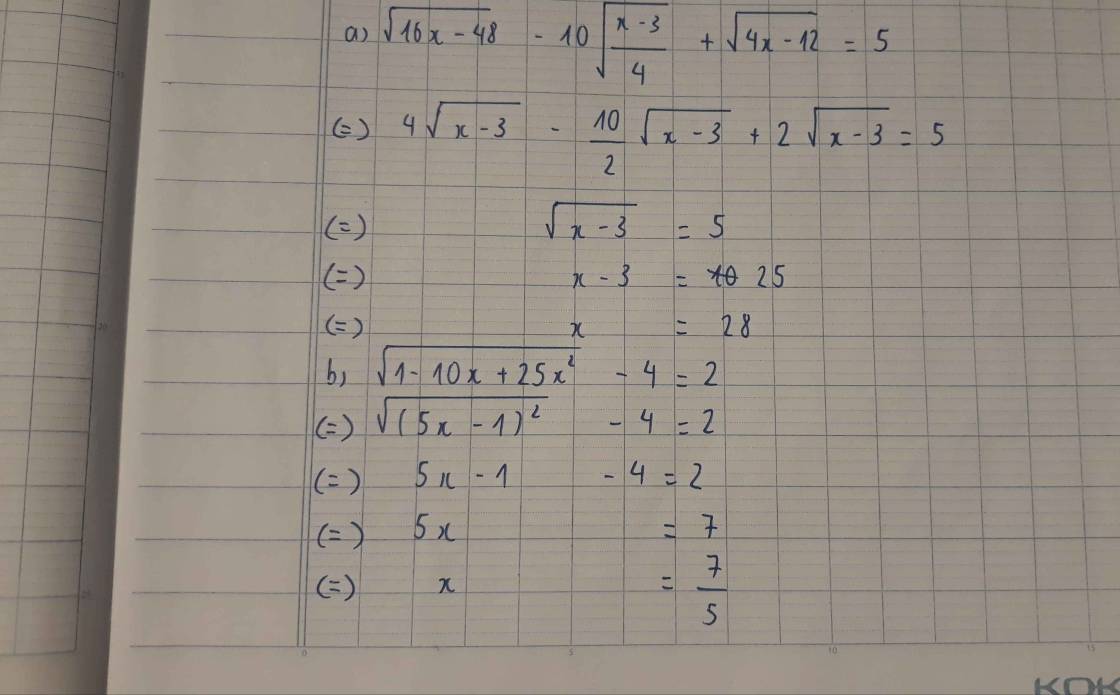
\(x^4-3x^3+4x^2-3x+1=0\)

Chia cả hai vế với \(x^2\)ta có

\(x^2-3x+4-\frac{3}{x}+\frac{1}{x^2}=0\)

\(\Leftrightarrow\left(x^2+\frac{1}{x^2}\right)-\left(3x+\frac{3}{x}\right)+4=0\)

\(\Leftrightarrow\left(x^2+\frac{1}{x^2}\right)-3.\left(x+\frac{1}{x}\right)+4=0\)

Đặt \(t=x+\frac{1}{x}\left(t>0\right)\)    \(\Rightarrow t^2-2=x^2+\frac{1}{x^2}\)

\(t^2-2-3t+4=0\)

\(\Leftrightarrow t^2-3t+2=0\)

\(\Leftrightarrow t^2-t-2t+2=0\)

\(\Leftrightarrow t.\left(t-1\right)-2.\left(t-1\right)=0\)

\(\Leftrightarrow\left(t-1\right).\left(t-2\right)=0\)

\(\Leftrightarrow\orbr{\begin{cases}t-1=0\\t-2=0\end{cases}\Leftrightarrow}\orbr{\begin{cases}t=1\left(TM\right)\\t=2\left(TM\right)\end{cases}}\)

TH1 \(t=1\)\(\Rightarrow x+\frac{1}{x}=1\)

\(\Leftrightarrow\frac{x^2+1}{x}=1\)\(\Leftrightarrow x^2+1=x\)

                                  \(\Leftrightarrow x^2-x+1=0\)

                                  \(\Leftrightarrow\left(x^2-x+\frac{1}{4}\right)+\frac{3}{4}=0\)

                                 \(\Leftrightarrow\left(x-\frac{1}{2}\right)^2+\frac{3}{4}=0\)   (Vô nghiệm)

TH2 \(t=2\)  \(\Rightarrow x+\frac{1}{x}=2\)

\(\Leftrightarrow\frac{x^2+1}{x}=2\)   \(\Leftrightarrow x^2+1=2x\)

                                     \(\Leftrightarrow x^2-2x+1=0\)

                                     \(\Leftrightarrow\left(x-1\right)^2=0\)

                                     \(\Leftrightarrow x-1=0\)

                                    \(\Leftrightarrow x=1\)

Vậy \(x=1\)