K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
2
16 tháng 12 2022
`a)1/[x-5x^2]-[25x-15]/[25x^2-1]`
`=[-(5x+1)-x(25x-15)]/[x(5x-1)(5x+1)]`
`=[-5x-1-25x^2+15x]/[x(5x-1)(5x+1)]`
`=[-25x^2+10x-1]/[x(5x-1)(5x+1)]`
`=[-(5x-1)^2]/[x(5x-1)(5x+1)]`
`=[1-5x]/[x(5x+1)]`
________________________________________________-
`b)(-1/[x^2-4x]+2/[16-x^2]-[-1]/[4x+16]):1/[4x]`
`=[-4(x+4)-8x+x(x-4)]/[4x(x-4)(x+4)].4x`
`=[-4x-16-8x+x^2-4x]/[(x-4)(x+4)]`
`=[x^2-16x-16]/[x^2-16]`
TT
30 tháng 11 2018
a, \(\dfrac{4x+13}{5x\left(x-7\right)}-\dfrac{x-48}{5x\left(7-x\right)}\)
\(=\dfrac{4x+13}{5x\left(x-7\right)}+\dfrac{x-48}{5x\left(x-7\right)}\)
\(=\dfrac{4x+13+x-48}{5x\left(x-7\right)}\)
\(=\dfrac{5x-35}{5x\left(x-7\right)}\)
\(=\dfrac{5\left(x-7\right)}{5x\left(x-7\right)}=\dfrac{1}{x}\)
b, \(\dfrac{1}{x-5x^2}-\dfrac{25x-15}{25x^2-1}\)
\(=\dfrac{1}{x\left(1-5x\right)}+\dfrac{25x-15}{\left(1-5x\right)\left(1+5x\right)}\)
\(=\dfrac{1+5x}{x\left(x-5x\right)\left(1+5x\right)}+\dfrac{x\left(25x-15\right)}{x\left(1-5x\right)\left(1+5x\right)}\)
\(=\dfrac{1+5x+25x^2-15x}{x\left(1-5x\right)\left(1+5x\right)}\)\(=\dfrac{25x^2-10x+1}{x\left(1-5x\right)\left(1+5x\right)}=\dfrac{\left(5x-1\right)^2}{x.\left(1-5x\right)\left(1+5x\right)}\)
\(=\dfrac{\left(5x-1\right)^2}{-x\left(5x-1\right)\left(1+5x\right)}\) \(=\dfrac{-\left(5x-1\right)}{x\left(1+5x\right)}\)
S
2
KT
14 tháng 12 2017
\(\frac{1}{x-5x^2}\)\(-\)\(\frac{25x-15}{25x^2-1}\)\(=\)\(\frac{-1}{x\left(5x-1\right)}\)\(-\)\(\frac{25x-15}{\left(5x-1\right)\left(5x+1\right)}\)
\(=\)\(\frac{-1\left(5x+1\right)}{x\left(5x-1\right)\left(5x+1\right)}\)\(-\)\(\frac{\left(25x^{ }-15\right)x}{\left(5x-1\right)\left(5x+1\right)x}\)
\(=\) \(\frac{-5x-1-25x^2+15x}{x\left(5x-1\right)\left(5x+1\right)}\)\(=\)\(\frac{-25x^2+10x-1}{x\left(5x-1\right)\left(5x+1\right)}\)
\(=\)\(\frac{-\left(5x-1\right)^2}{x\left(5x-1\right)\left(5x+1\right)}\)\(=\)\(\frac{-5x+1}{5x^2+x}\)
S
14 tháng 12 2017
\(\frac{1}{x-5x^2}-\frac{25x-15}{25x^2-1}\)
\(=\frac{1}{x\left(1-5x\right)}-\frac{25x-15}{\left(5x-1\right)\left(5x+1\right)}\)
\(=\frac{1}{x\left(1-5x\right)}+\frac{25x-15}{\left(1-5x\right)\left(5x+1\right)}\)
\(=\frac{5x+1}{x\left(1-5x\right)\left(5x+1\right)}+\frac{\left(25x-15\right)x}{x\left(1-5x\right)\left(5x+1\right)}\)
\(=\frac{5x+1+25x^2+15x}{x\left(1-5x\right)\left(5x-1\right)}\)
\(=\frac{25x^2-10x+1}{x\left(1-5x\right)\left(5x+1\right)}\)
\(=\frac{\left(5x-1\right)^2}{x\left(1-5x\right)\left(5x+1\right)}\)
\(=\frac{\left(1-5x\right)^2}{x\left(1-5x\right)\left(5x+1\right)}\)
\(=\frac{1-5x}{x\left(5x+1\right)}\)
DH
10 tháng 9 2017
(5x-1)(5x+1)=25x2-7x+15
<=> 25x2-1-25x2+7x-15=0
<=>7x=16
=>x=16/7
4 tháng 12 2016
a)\(dk,x\ne7;x\ne0\)
\(\frac{4x+13}{5x\left(x-7\right)}-\frac{x-48}{5x\left(7-x\right)}=\frac{4x+13}{5x\left(x-7\right)}+\frac{x-48}{5x\left(x-7\right)}=\frac{\left(4x+13\right)+\left(x-48\right)}{5x\left(x-7\right)}\\ \)
\(=\frac{5x-35}{5x\left(x-7\right)}=\frac{5\left(x-7\right)}{5x\left(x-7\right)}=\frac{1}{x}\)
b)
\(\frac{1}{x-5x^2}-\frac{25x-15}{25x^2-1}=\frac{1}{x\left(1-5x\right)}+\frac{25x-15}{1-\left(5x\right)^2}=\frac{1}{x\left(1-5x\right)}+\frac{25x-15}{\left(1-5x\right)\left(1+5x\right)}\)
\(\frac{1+5x}{x\left(1-5x\right)\left(1+5x\right)}+\frac{x\left(25x-15\right)}{x\left(1-5x\right)\left(1+5x\right)}=\frac{25x^2-15x+5x+1}{x\left(1-5x\right)\left(1+5x\right)}=\frac{25x^2-10x+1}{x\left(1-5x\right)\left(1+5x\right)}\)
PT
4 tháng 12 2017
\(\dfrac{1}{x-5x^2}+\dfrac{25x-15}{25x^2-1}\)
\(=\dfrac{1}{x\left(1-5x\right)}+\dfrac{25x-15}{\left(1-5x\right)\left(1+5x\right)}\)
\(=\dfrac{1+5x+x\left(25x-15\right)}{x\left(1-5x\right)\left(1+5x\right)}\)
\(=\dfrac{1+5x+25x^2-15}{x\left(1-5x\right)\left(1+5x\right)}\)
\(=\dfrac{1-10x+25x^2}{x\left(1-5x\right)\left(1+5x\right)}\)
\(=\dfrac{\left(1-5x\right)^2}{x\left(1-5x\right)\left(1+5x\right)}\)
\(=\dfrac{1-5x}{x\left(1+5x\right)}\)
NN
5 tháng 12 2017
\(\dfrac{1}{x-5x^2}-\dfrac{25x-15}{25x^2-1}\)
\(=\dfrac{1}{x-5x^2}+\dfrac{25x-15}{1-25x^2}\)
\(=\dfrac{1}{x\left(1-5x\right)}+\dfrac{25x-15}{\left(1-5x\right)\left(1+5x\right)}\) MTC: \(x\left(1-5x\right)\left(1+5x\right)\)
\(=\dfrac{1+5x}{x\left(1-5x\right)\left(1+5x\right)}+\dfrac{x\left(25x-15\right)}{x\left(1-5x\right)\left(1+5x\right)}\)
\(=\dfrac{1+5x+x\left(25x-15\right)}{x\left(1-5x\right)\left(1+5x\right)}\)
\(=\dfrac{1+5x+25x^2-15x}{x\left(1-5x\right)\left(1+5x\right)}\)
\(=\dfrac{25x^2-10x+1}{x\left(1-5x\right)\left(1+5x\right)}\)
\(=\dfrac{\left(5x-1\right)^2}{x\left(1-5x\right)\left(1+5x\right)}\)
\(=\dfrac{\left(1-5x\right)^2}{x\left(1-5x\right)\left(1+5x\right)}\)
\(=\dfrac{1-5x}{x\left(1+5x\right)}\)
Giups mk vs thank cacs bn
5 tháng 2 2021
b) PT \(\Leftrightarrow15x\left(5x+3\right)-35\left(5x+3\right)=0\)
\(\Leftrightarrow\left(15x-35\right)\left(5x+3\right)=0\) \(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{7}{3}\\x=-\dfrac{3}{5}\end{matrix}\right.\)
Vậy \(S=\left\{-\dfrac{3}{5};\dfrac{7}{3}\right\}\)
c) PT \(\Leftrightarrow\left(2-3x\right)\left(x-11\right)+\left(2-3x\right)\left(2-5x\right)=0\)
\(\Leftrightarrow\left(2-3x\right)\left(-9-4x\right)=0\) \(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{2}{3}\\x=-\dfrac{9}{4}\end{matrix}\right.\)
Vậy \(S=\left\{\dfrac{2}{3};-\dfrac{9}{4}\right\}\)
R
5 tháng 2 2021
a)(x-1)(5x+3)=(3x-8)(x-1)
\(\Leftrightarrow\)(x-1)(5x+3)-(3x-8)(x-1)=0
\(\Leftrightarrow\left(x-1\right)\left(5x-3-3x+8\right)=0\)
\(\Leftrightarrow\left(x-1\right)\left(2x-5\right)=0\)
\(\left[{}\begin{matrix}x-1=0\\2x-5=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=1\\x=\dfrac{5}{2}\end{matrix}\right.\)
Vậy \(x\in\left\{1;\dfrac{5}{2}\right\}\)
24 tháng 10 2021
d: ta có: \(x^2-4x+4=9\left(x-2\right)\)
\(\Leftrightarrow\left(x-2\right)\left(x-11\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=2\\x=11\end{matrix}\right.\)
???????????????????????????????????????????????????????????????????????????
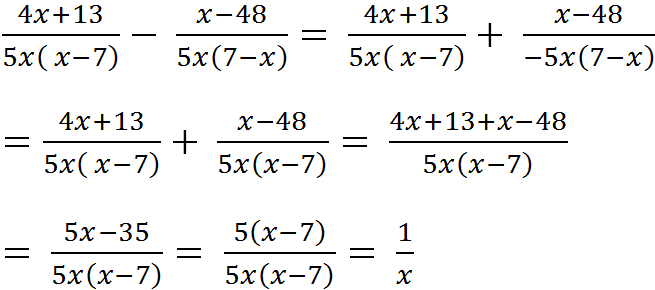