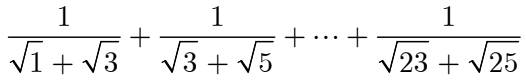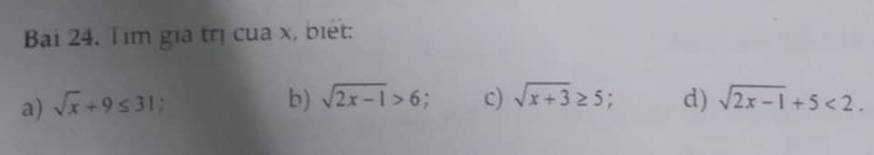a) \(x^2-11=\left(x-\sqrt{11}\right)\left(x+\sqrt{11}\right)\)

b) \(x-3\sqrt{x}+4=x-4\sqrt{x}+\sqrt{x}-4=\left(\sqrt{x}-4\right)\left(\sqrt{x}+1\right)\)

c) \(x-5=\left(\sqrt{x}-\sqrt{5}\right)\left(\sqrt{x}+\sqrt{5}\right)\)

d) \(x+5\sqrt{x}+6=\left(x+3\sqrt{x}\right)+\left(2\sqrt{x}+6\right)=\left(\sqrt{x}+3\right)\left(\sqrt{x}+2\right)\)

a) \(^{x^2}\)- 11 = ( x - \(\sqrt{11}\) )(x + \(\sqrt{11}\) )

Ta có \(\dfrac{1}{\sqrt{n}+\sqrt{n+2}}=\dfrac{\sqrt{n+2}-\sqrt{n}}{\left(\sqrt{n+2}+\sqrt{n}\right)\left(\sqrt{n+2}-\sqrt{n}\right)}\) \(=\dfrac{\sqrt{n+2}-\sqrt{n}}{\left(\sqrt{n+2}\right)^2-\left(\sqrt{n}\right)^2}\) \(=\dfrac{\sqrt{n+2}-\sqrt{n}}{2}\)

Như vậy, ta có \(\dfrac{1}{\sqrt{1}+\sqrt{3}}+\dfrac{1}{\sqrt{3}+\sqrt{5}}+...+\dfrac{1}{\sqrt{23}+\sqrt{25}}\) 

\(=\dfrac{\sqrt{3}-1}{2}+\dfrac{\sqrt{5}-\sqrt{3}}{2}+\dfrac{\sqrt{7}-\sqrt{5}}{2}+...+\dfrac{\sqrt{25}-\sqrt{23}}{2}\)

\(=\dfrac{\sqrt{3}-1+\sqrt{5}-\sqrt{3}+\sqrt{7}-\sqrt{5}+...+\sqrt{25}-\sqrt{23}}{2}\) 

\(=\dfrac{\sqrt{25}-1}{2}=\dfrac{5-1}{2}=2\)

\(A=\dfrac{1}{\sqrt{1}+\sqrt{3}}+\dfrac{1}{\sqrt{3}+\sqrt{5}}+...+\dfrac{1}{\sqrt{23}+\sqrt{25}}\)

\(=\dfrac{\sqrt{3}-\sqrt{1}}{\left(\sqrt{3}-\sqrt{1}\right)\left(\sqrt{3}+1\right)}+\dfrac{\sqrt{5}-\sqrt{3}}{\left(\sqrt{5}-\sqrt{3}\right)\left(\sqrt{5}+\sqrt{3}\right)}+...+\dfrac{\sqrt{25}-\sqrt{23}}{\left(\sqrt{25}-\sqrt{23}\right)\left(\sqrt{25}+\sqrt{23}\right)}\)

\(=\dfrac{\sqrt{3}-1}{2}+\dfrac{\sqrt{5}-\sqrt{3}}{2}+...+\dfrac{\sqrt{25}-\sqrt{23}}{2}\)

\(=-\dfrac{1}{2}+\dfrac{\sqrt{3}}{2}-\dfrac{\sqrt{3}}{2}+\dfrac{\sqrt{5}}{2}-...-\dfrac{\sqrt{23}}{2}+\dfrac{\sqrt{25}}{2}\\ =\dfrac{1}{2}+\dfrac{\sqrt{25}}{2}=\dfrac{1}{2}+\dfrac{5}{2}=3\)

a) ĐK: \(x\ge0\)

\(\sqrt{x}+9\le31\Leftrightarrow\sqrt{x}\le22\Leftrightarrow x\le484\)

Kết hợp với đk ta được \(0\le x\le484\)

b) ĐK: \(2x-1\ge0\Leftrightarrow x\ge\dfrac{1}{2}\)

Ta có \(\sqrt{2x-1}>6\Leftrightarrow2x-1>36\Leftrightarrow x>\dfrac{37}{2}\) (TM)

Vậy \(x>\dfrac{37}{2}\)

c) ĐK: \(x+3\ge0\Leftrightarrow x\ge-3\)

Ta có \(\sqrt{x+3}\ge5\Leftrightarrow x+3\ge25\Leftrightarrow x\ge22\) (TM)

Vậy \(x\ge22\)

d) ĐK: \(2x-1\ge0\Leftrightarrow x\ge\dfrac{1}{2}\)

Ta có \(\sqrt{2x-1}+5< 2\Leftrightarrow\sqrt{2x-1}< -3\) (vô lí)

Vậy không có \(x\) thỏa mãn.

giúp mình với ạ

                (phương pháp phản chứng )

giả sử x + \(\dfrac{1}{x}\) ϵ Q ⇔ x + \(\dfrac{1}{x}\) = \(\dfrac{a}{b}\) (a,b ϵN, b#0)

⇔ x = \(\dfrac{a}{b}\) - \(\dfrac{1}{x}\)⇔ x - \(\dfrac{1}{x}\) = \(\dfrac{a}{b}\) - \(\dfrac{1}{x}\) - \(\dfrac{1}{x}\) ⇔ x - \(\dfrac{1}{x}\) = \(\dfrac{a}{b}\)- \(\dfrac{2}{x}\)

nếu x = 2 ta có x - \(\dfrac{1}{x}\) = 2 - \(\dfrac{1}{2}\) = \(\dfrac{3}{4}\) (loại vì \(\dfrac{3}{4}\) không thuộc Z)

nếu \(\dfrac{a}{b}\)= \(\dfrac{2}{x}\) ⇔ x - \(\dfrac{1}{x}\) = 0 ⇔ x = +- 1 (loại) ⇔ \(\dfrac{a}{b}\) # \(\dfrac{2}{x}\)

vậy với x # +-1

⇔ x - \(\dfrac{1}{x}\)= \(\dfrac{a}{b}\) - \(\dfrac{2}{x}\)  \(\notin\)  Z ⇔ x + \(\dfrac{1}{x}\) \(\notin\) Q ⇔ x + \(\dfrac{1}{x}\) \(\in\) I (đpcm)

ĐKXĐ: \(x\ge-\dfrac{9}{2};x\ne0\)

\(\dfrac{2x^2}{\left(3-\sqrt{9+2x}\right)^2}=x+9\)

\(\Rightarrow\dfrac{2x^2\left(3+\sqrt{9+2x}\right)^2}{\left(3-\sqrt{9+2x}\right)^2\left(3+\sqrt{9+2x}\right)^2}=x+9\)

\(\Rightarrow\dfrac{2x^2\left(3+\sqrt{9+2x}\right)^2}{4x^2}=x+9\)

\(\Rightarrow\left(3+\sqrt{9+2x}\right)^2=2x+18\)

Đặt \(\sqrt{2x+9}=t\ge0;t\ne3\)

\(\Rightarrow\left(3+t\right)^2=t^2+9\Rightarrow t=0\)

\(\Rightarrow\sqrt{2x+9}=0\Rightarrow x=-\dfrac{9}{2}\)