giúp em làm câu đầu với ạ em cảm ơn
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
T
1
2 tháng 1 2022
a: Xét (O) có
MA là tiếp tuyến
MB là tiếp tuyến
Do đó: MA=MB
hay M nằm trên đường trung trực của AB(1)
Ta có: OA=OB
nên O nằm trên đường trung trực của AB(2)
Từ (1) và (2) suy ra OM⊥AB
S
_silverlining
CTVVIP
12 tháng 5 2022
Para 1 - b
Para 2 - a
Para 3 - c
T - F - T - T - NG
1 B
2 A
3 D
4 D
5 A
16 tháng 11 2021
Bài 3:
\(b,\Leftrightarrow\left(x+8\right)\left(x+8-3x\right)=0\\ \Leftrightarrow\left(x+8\right)\left(8-2x\right)=0\\ \Leftrightarrow2\left(4-x\right)\left(x+8\right)=0\Leftrightarrow\left[{}\begin{matrix}x=4\\x=-8\end{matrix}\right.\)
GN
GV Nguyễn Trần Thành Đạt
Giáo viên
18 tháng 2 2022
Bài 79:
\(2Fe+3Cl_2\rightarrow\left(t^o\right)2FeCl_3\\ Cu+Cl_2\rightarrow\left(t^o\right)CuCl_2\\ Đặt:n_{Fe}=a\left(mol\right);n_{Cu}=b\left(mol\right)\left(a,b>0\right)\\ \Rightarrow\left\{{}\begin{matrix}56a+64b=60\\33,6a+22,4b=28\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}a=0,5\\b=0,5\end{matrix}\right.\\ \Rightarrow\%m_{Cu}=\dfrac{64.50\%}{60}.100=\approx53,333\%\)
Chọn B
GN
GV Nguyễn Trần Thành Đạt
Giáo viên
18 tháng 2 2022
Bài 80:
\(M+Cl_2\rightarrow\left(t^o\right)MCl_2\\ ĐLBTKL:m_M+m_{Cl_2}=m_{MCl_2}\\ \Leftrightarrow2,42+m_{Cl_2}=9,5\\ \Leftrightarrow m_{Cl_2}=7,08\left(g\right)\\ \Rightarrow n_{Cl_2}=\dfrac{7,08}{71}=\dfrac{177}{1775}\left(mol\right)\\ \Rightarrow n_M=n_{Cl_2}=\dfrac{177}{1775}\left(mol\right)\\ \Rightarrow M_M=\dfrac{2,42}{\dfrac{177}{1775}}\approx24,268\left(\dfrac{g}{mol}\right)\\ \Rightarrow M:Magie\left(Mg\right)\\ \Rightarrow ChọnB\)
NV
Nguyễn Việt Lâm
Giáo viên
7 tháng 6 2021
7a.
\(y'=3x^2-2\left(m-1\right)x-m-3\)
Hàm nghịch biến trên \(\left(-1;0\right)\) khi và chỉ khi \(y'\le0\) ; \(\forall x\in\left(-1;0\right)\)
\(\Leftrightarrow3x^2-2\left(m-1\right)x-m-3\le0\)
\(\Leftrightarrow\left\{{}\begin{matrix}\Delta'=\left(m-1\right)^2+3\left(m+3\right)>0\\x_1\le-1< 0\le x_2\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}m^2+m+10>0\left(\text{luôn đúng}\right)\\f\left(-1\right)\le0\\f\left(0\right)\le0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}3+2\left(m-1\right)-m-3\le0\\-m-3\le0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}m-2\le0\\-m-3\le0\end{matrix}\right.\) \(\Leftrightarrow-3\le m\le2\)
NV
Nguyễn Việt Lâm
Giáo viên
7 tháng 6 2021
7b.
\(y'=-x^2+2\left(m-1\right)x+m+3\)
Hàm đồng biến trên \(\left(0;3\right)\) khi và chỉ khi \(y'\le0\) ; \(\forall x\in\left(0;3\right)\)
\(\Leftrightarrow-x^2+2\left(m-1\right)x+m+3\ge0\) ; \(\forall x\in\left(0;3\right)\)
\(\Leftrightarrow m\left(2x+1\right)\ge x^2+2x-3\)
\(\Leftrightarrow m\ge\dfrac{x^2+2x-3}{2x+1}\)
\(\Leftrightarrow m\ge\max\limits_{\left[0;3\right]}\dfrac{x^2+2x-3}{2x+1}\)
Xét hàm \(f\left(x\right)=\dfrac{x^2+2x-3}{2x+1}\) trên \(\left(0;3\right)\)
\(f'\left(x\right)=\dfrac{2\left(x^2+x+4\right)}{\left(2x+1\right)^2}>0\) ; \(\forall x\Rightarrow f\left(x\right)\) đồng biến
\(\Rightarrow f\left(x\right)< f\left(3\right)=\dfrac{12}{7}\)
\(\Rightarrow m\ge\dfrac{12}{7}\)
AT
3 tháng 7 2021
2) \(\dfrac{\left(1+\sqrt{a}\right)^2-\left(2-\sqrt{a}\right)^2}{1-2\sqrt{a}}:\dfrac{\sqrt{a}}{3}\left(a>0,a\ne\dfrac{1}{4}\right)\)
\(=\dfrac{\left(1+\sqrt{a}-2+\sqrt{a}\right)\left(1+\sqrt{a}+2-\sqrt{a}\right)}{1-2\sqrt{a}}.\dfrac{3}{\sqrt{a}}\)
\(=\dfrac{3.\left(2\sqrt{a}-1\right)}{1-2\sqrt{a}}.\dfrac{3}{\sqrt{a}}=-\dfrac{9}{\sqrt{a}}\)
5) \(\left(5-\dfrac{a+3\sqrt{a}}{\sqrt{a}+3}\right)\left(2-\dfrac{3a+\sqrt{a}}{3\sqrt{a}+1}\right)\left(a\ge0\right)\)
\(=\left(5-\dfrac{\sqrt{a}\left(\sqrt{a}+3\right)}{\sqrt{a}+3}\right)\left(2-\dfrac{\sqrt{a}\left(3\sqrt{a}+1\right)}{3\sqrt{a}+1}\right)\)
\(=\left(5-\sqrt{a}\right)\left(2-\sqrt{a}\right)=10-7\sqrt{a}+a\)
6) \(\left(2-\dfrac{a-3\sqrt{a}}{\sqrt{a}-3}\right)\left(2-\dfrac{5\sqrt{a}-\sqrt{ab}}{\sqrt{b}-5}\right)\left(a,b\ge0,a\ne9,b\ne25\right)\)
\(=\left(2-\dfrac{\sqrt{a}\left(\sqrt{a}-3\right)}{\sqrt{a}-3}\right)\left(2+\dfrac{\sqrt{a}\left(\sqrt{b}-5\right)}{\sqrt{b}-5}\right)\)
\(=\left(2-\sqrt{a}\right)\left(2+\sqrt{a}\right)=4-a\)
3 tháng 7 2021
3) Ta có: \(\dfrac{a+4\sqrt{a}+4}{\sqrt{a}+2}+\dfrac{4-a}{\sqrt{a}-2}\)
\(=\dfrac{\left(\sqrt{a}+2\right)^2}{\sqrt{a}+2}-\dfrac{\left(\sqrt{a}+2\right)\left(\sqrt{a}-2\right)}{\sqrt{a}-2}\)
\(=\sqrt{a}+2-\sqrt{a}-2\)
=0
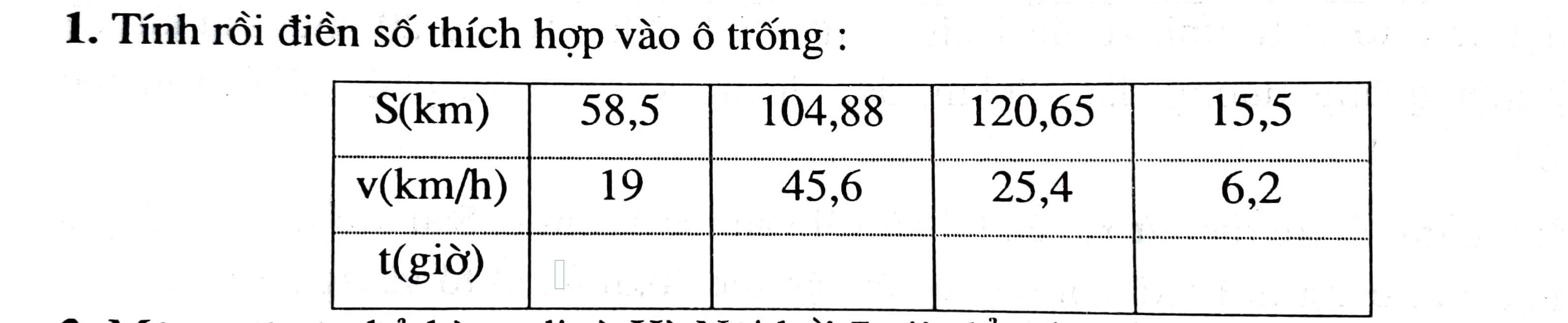
117/38 | 2,3 | 4,75 | 2,5 |
1. 3.078947368421053 giờ
2. 2.3giờ
3. 4.75 giờ
4. 2.5 giờ